José L. Rodríguez
Artists
José L. Rodríguez, Diego Cangas
Associate Professor of Mathematics
Department of Mathematics, University of Almería
Almería
http://topologia.wordpress.com
Statement
I enjoy making real models of known geometrical and topological objects by hand, so useful to students to learn and understand their properties. In my blogs, you can find many math experiences where we play with objects of rubber, felt, thread, wire, and even with soap films. As a string artist I like very much to reproduce big polytopes like the E8 Polytope that I presented last Bridges 2013, or islamic patterns like the one of the present exhibition.
Artworks
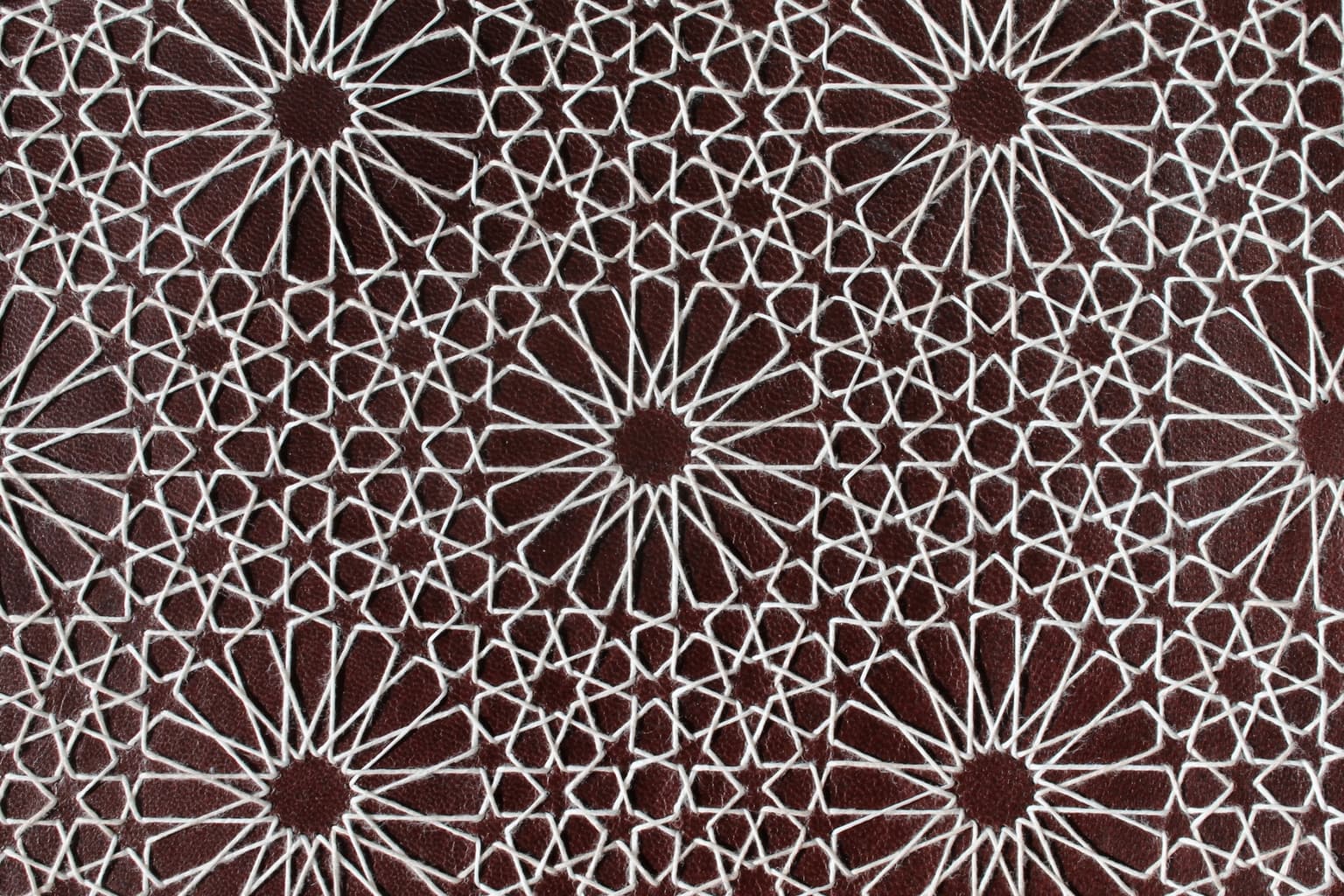
This hand work reproduces the knotting structure of a famous tile in the Alhambra of Granada, Spain. We had already made this and other islamic patterns with threads and pins nailed on a wooden board, but the use of cotton thread and leather would surely be a beautiful way to represent these islamic geometric patterns at the time.
The symmetry group of this tiling is the wallpaper group P4, generated by two rotation centres of order four (90°), and one rotation centre of order two (180°). If one does not take into account the crossings, then its symmetry group is the wallpaper group PM4, generated by three mirror reflections having angles of 90º, 45º and 45º.