Horst Schaefer
Artists
Statement
I am trying to apply formal concepts from mathematics, logic or science in my work. One of my goals is to reach a balance between these formal aspects, artistic freedom and the resulting aesthetic appearance. For some years I was experimenting with the golden section. If you apply the golden section to one distance you split this distance into two parts. The two parts have then the ratio called PHI (or roughly 1,618). One can apply this procedure to the sides of a square or a rectangle recursively. As a result you get a recursive tiling of the square or the rectangle using the golden section as a ratio.
Artworks
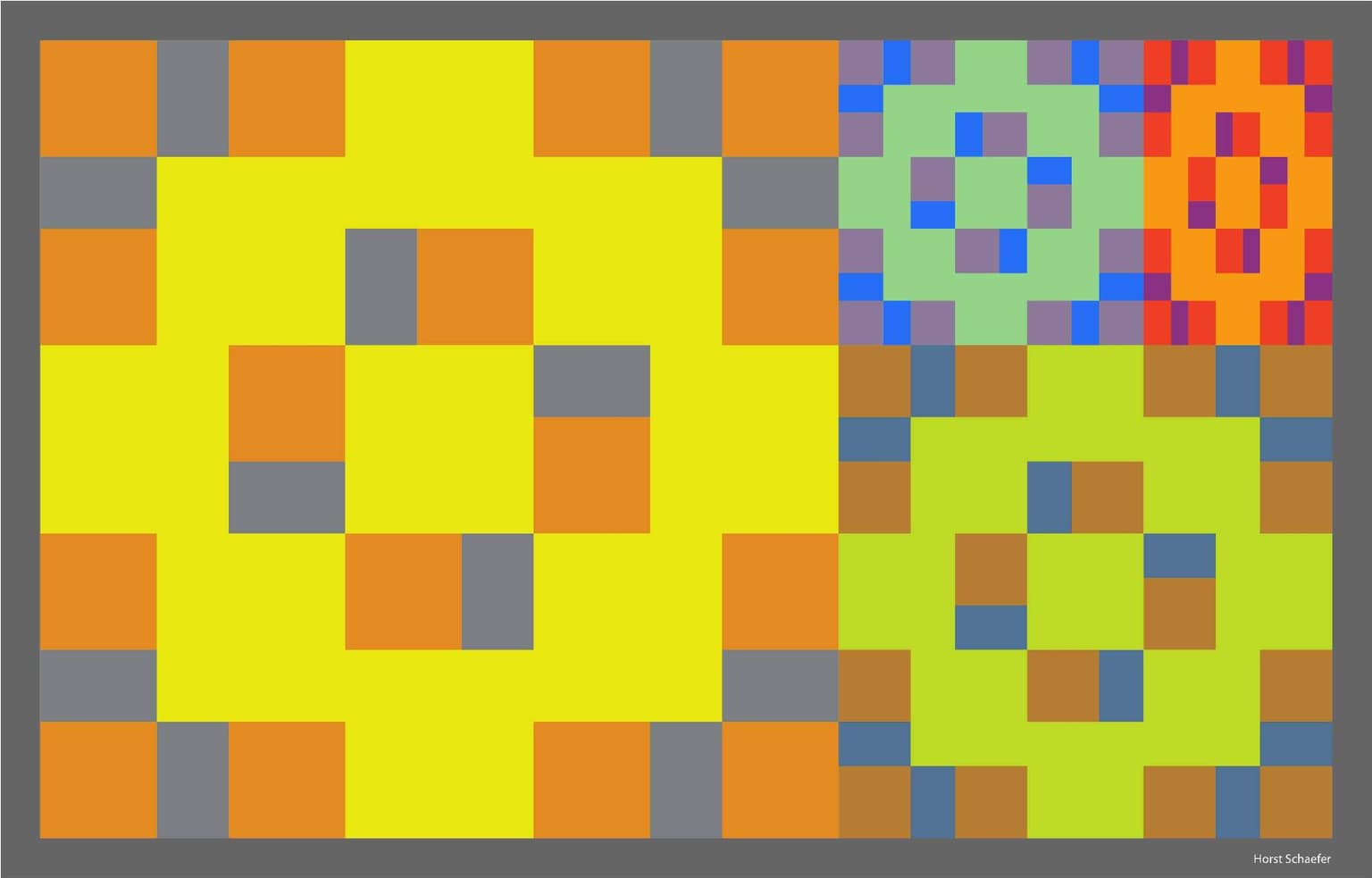
Golden Section - Recursive Tilings II
40 cm x 65 cm
Digital Print
2014
This golden section tiling starts with a rectangle having the proportions of the golden section. The next level is visible through separate colorings. The last level contains a special golden section pattern.
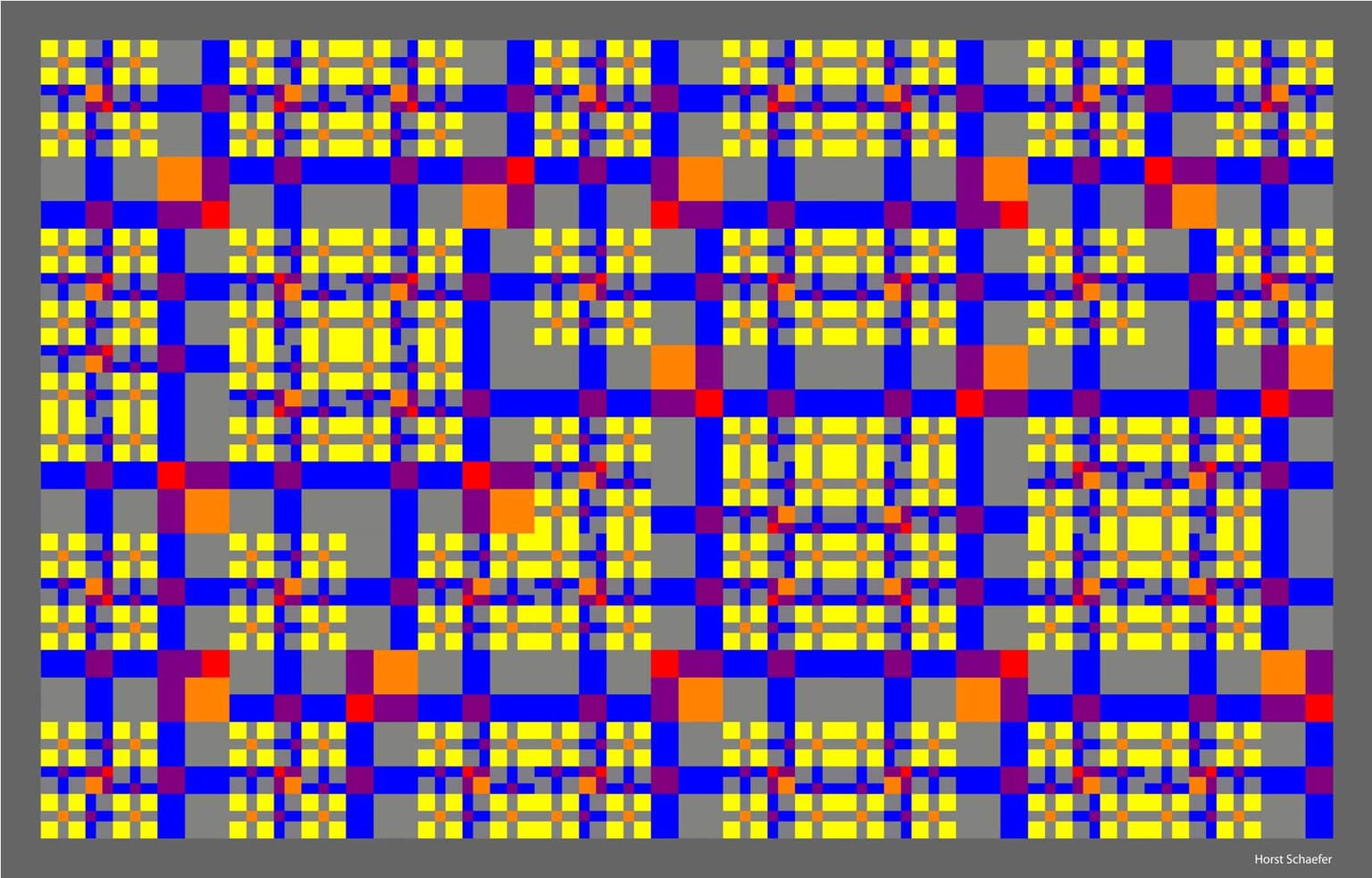
Golden Section - Recursive Tilings III
40 cm x 65 cm
Digital Print
2014
This golden section tiling starts with a rectangle having the proportions of the golden section. The different levels of the recursive tilings are laid over each other.
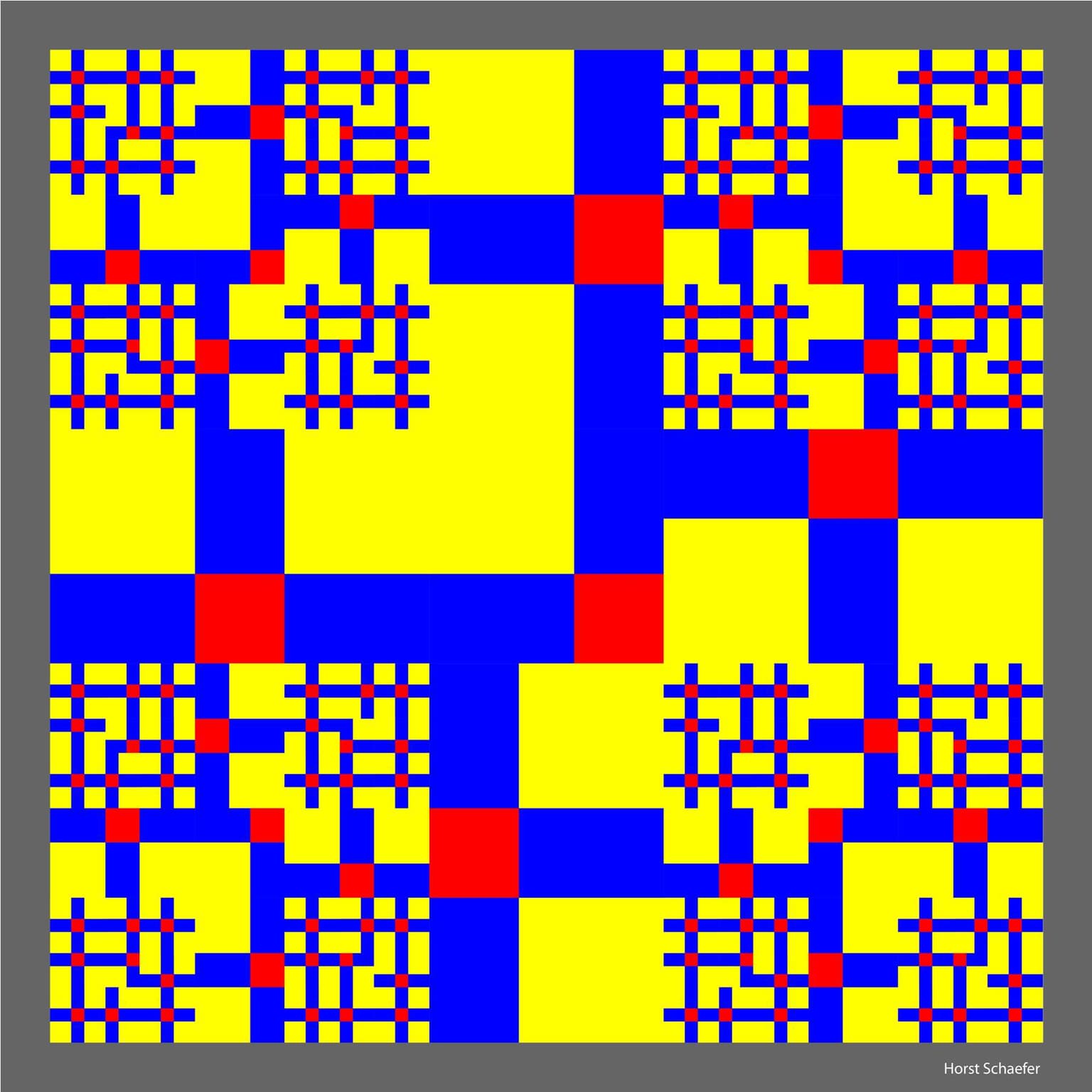
Golden Section - Recursive Tilings I
40 cm x 40 cm
Digital Print
2014
This recursive golden section tiling starts with a square. Only the thick lines remain visible from the first set of recursive tilings. The next level is partially visible, and the last level, with the thinnest lines is fully visible, giving a some how symmetical / asymmetrical impression.