Allen Hirsh

Statement

Instead of painting by hand, I have written software that employs linked equation sets of elementary functions to ferret out a small sample of the vast array of patterns hidden in photographs I use as raw material. Part of my art is the images created, part of it is the invention of my image transformation engine. I try to demonstrate that mathematical systems, properly crafted, can produce textures and abstractions that are as “painterly” as possible. Part of my work also involves the “hybridization” of images that are seemingly incompatible, e.g. flowers and printers, often producing startlingly unexpected results. Finally I have an overarching goal of producing images that are compellingly beautiful.

Artworks

This is a mathematical hybrid of a photograph of my brother Gene, dressed in a striped gray and white shirt and a bright red Hibiscus flower from my garden, silhouetted against dull gray aluminum siding. The dominant mathematical transformations were trigonometric and logarithmic and include significant mirroring of the initial hybridized image in the x direction. This produces the repetition of the image horizontally, i.e. a totem type structure, while the highly nonlinear local transformations insure that each repetition has a fascinatingly unique character. There is also a lower frequency mirroring in the negative y direction and it produces its own very different repetition of the original hybrid image.

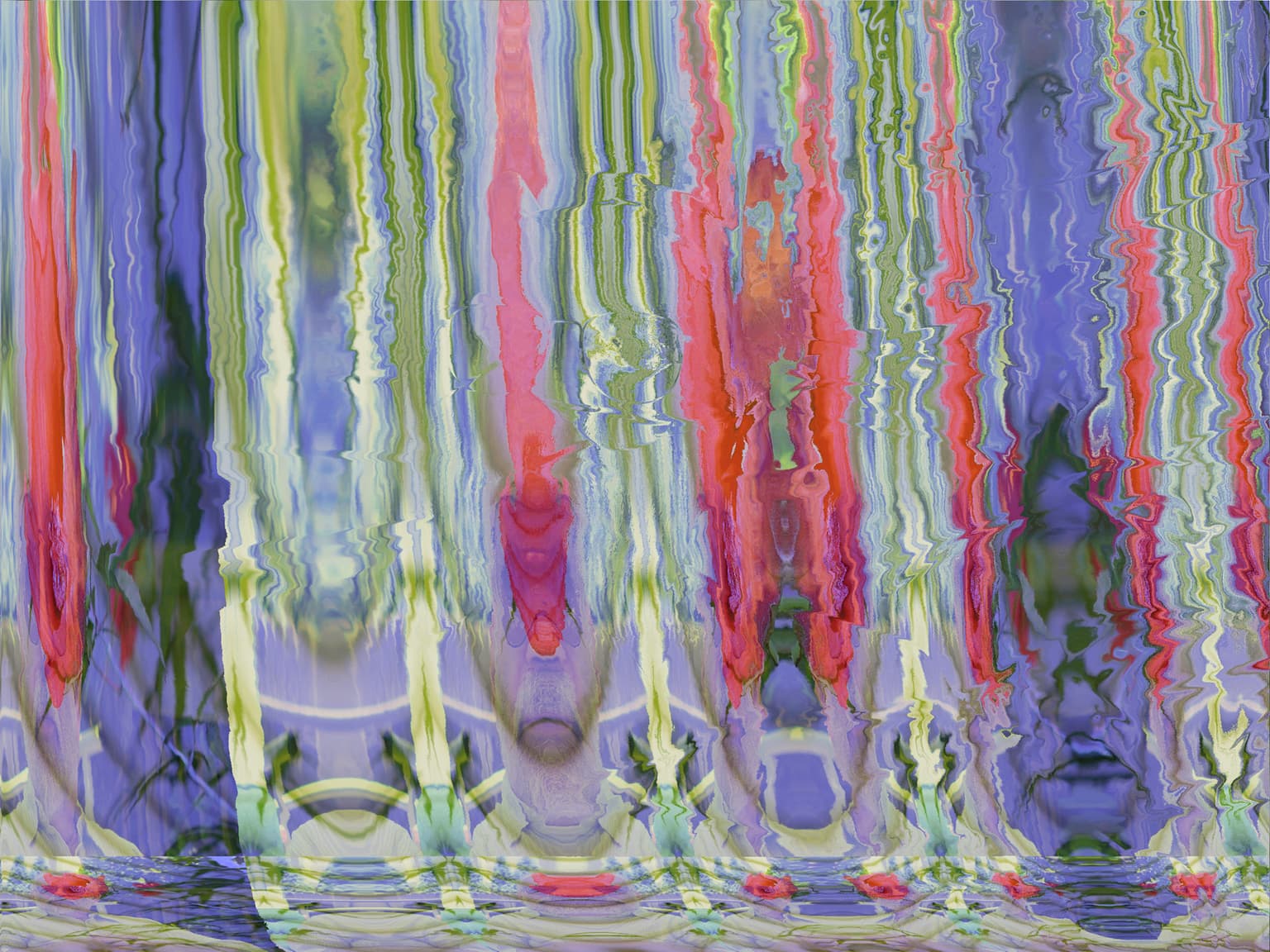
This transformation of a hybrid of a Hibiscus photo and a pink Camellia flower is dominated by exponential trigonometric and power function transformations. However, like Brother Gene's Totems it is strongly mirrored in the x direction and less strongly in the negative y direction producing the illusion of a strangely surreal reflection in water. This image was also run through a purely local color transformation part of the engine that is dominated by trigonometric transformations.