2014 Joint Mathematics Meetings

Christopher Bartlett

Artists

Christopher Bartlett

Professor Emeritus of Art

Towson University

Baltimore, Maryland, USA

cbartlett@towson.edu

Statement

I am interested in the geometry of composition in painting. An important principle of design and composition is repetition, so creating any structure such that the elements of a painting are aligned within self-similar areas and consequently at repeating measures from each other would satisfy the goal of unity. It is the thread that holds together an otherwise loose tapestry of forms in space and provides a structure of harmonizing ratios of proportion.

Artworks

Image for entry 'Grand Canal, Venice'

Grand Canal, Venice

40 x 54 cm.

acrylic on canvas

2013

I recently discovered the properties of a rectangle proportioned 1:1.356... If a line is drawn that is perpendicular to a diagonal of the canvas, that line is a diagonal of a smaller rectangle that is similar to the canvas rectangle. The gnomon is a golden rectangle. This 1.356 rectangle (I call 'chi' and is mathematically supported) has similar generative properties as the golden ratio rectangle itself. I have used the 1.356 proportions to define the geometric design of this painting of a palace on the Grand Canal, Venice where the main horizontal division at the bridge gives a golden ratio rectangle at the base and a similar 1.356 rectangle at the top. The upper rectangle divides again into golden ratio and similar rectangles, and so on.
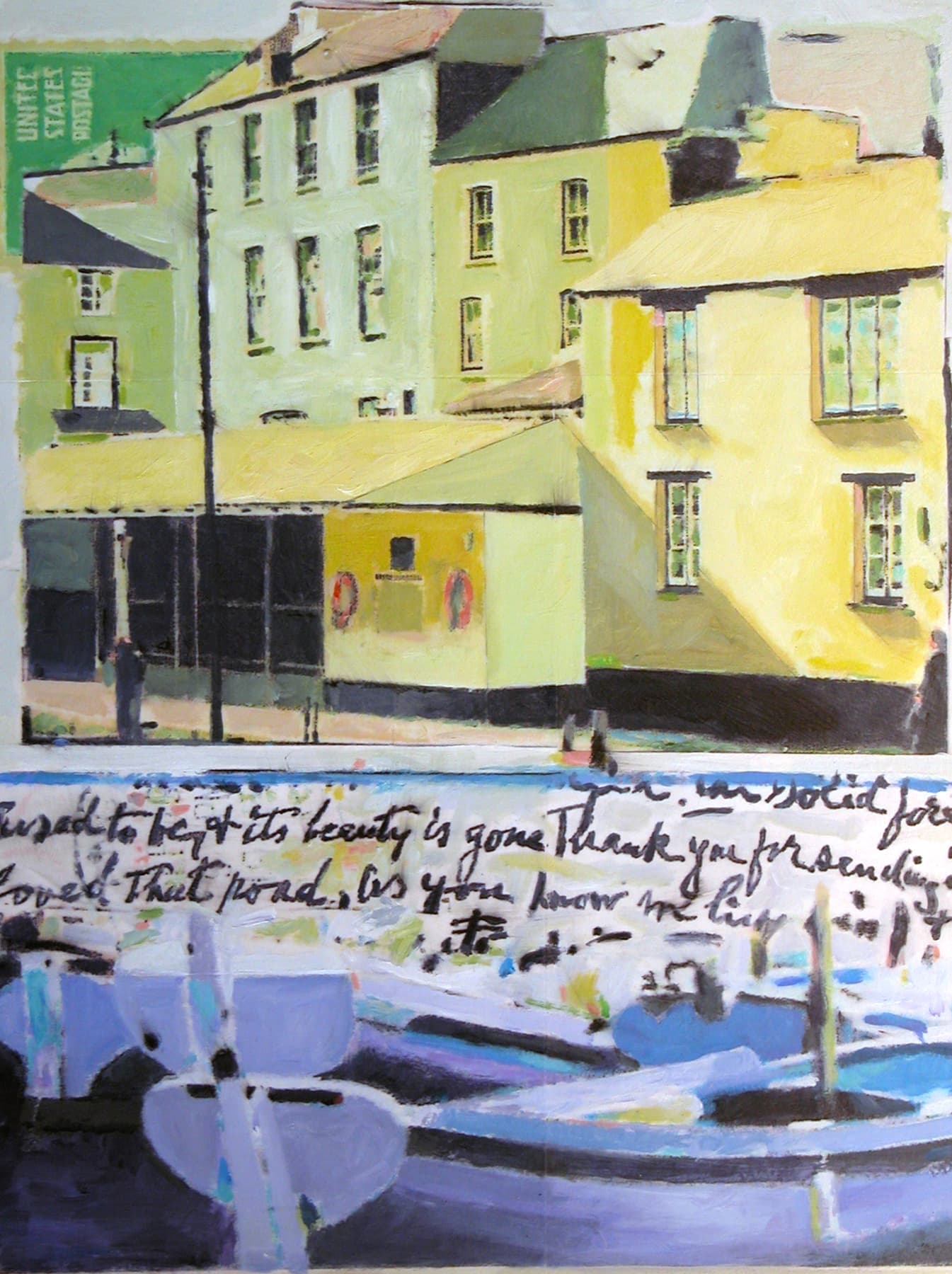
Image for entry 'Wish you were here.'

Wish you were here.

40 x 54 cm.

acrylic on canvas

2013

This work is based on the concept of picture postcards taking elements from front and back. The imagery is from my photos of an English harbor, Greek fishing boats and the overlaid handwriting from an antique postcard. The canvas size is in the 1:1.356... ('chi') ratio. This is a rectangle I discovered while analyzing the geometry of master paintings. This ratio governs the primary horizontals and verticals in the composition of the painting. A mathematical proof of the properties of the chi rectangle can be found in 'Art and Math of the 1.35 Ratio Rectangle', co-authored by Dirk Huylebrouck, Symmetry: Culture and Science, 2013 Vol. 24, No.1-4, 451-462.