2014 Joint Mathematics Meetings
Charles Gunn
Artists
Charles Gunn and Thomas Banchoff
Researcher and Teacher
Matheon, Technisches Universität Berlin
Berlin, Germany
Statement
I enjoy exploring two- and three-dimensional tessellations -- euclidean, spherical and hyperbolic, especially the "insider's" view of such spaces. I have been experimenting recently with alternative perspective rendering based on a spherical canvas surrounding the viewer. This "viewable sphere" is then flattened out via stereographic projection to make the image. In contrast to standard perspective images, the resulting images are conformal (small spheres in the scene are projected to small circles in the picture) and show a large fraction of the world. I have found this technique is well-suited to visualizing space-filling tessellations. I hope my work stimulates reflection in the observer on what "true perspective" means.
Artworks
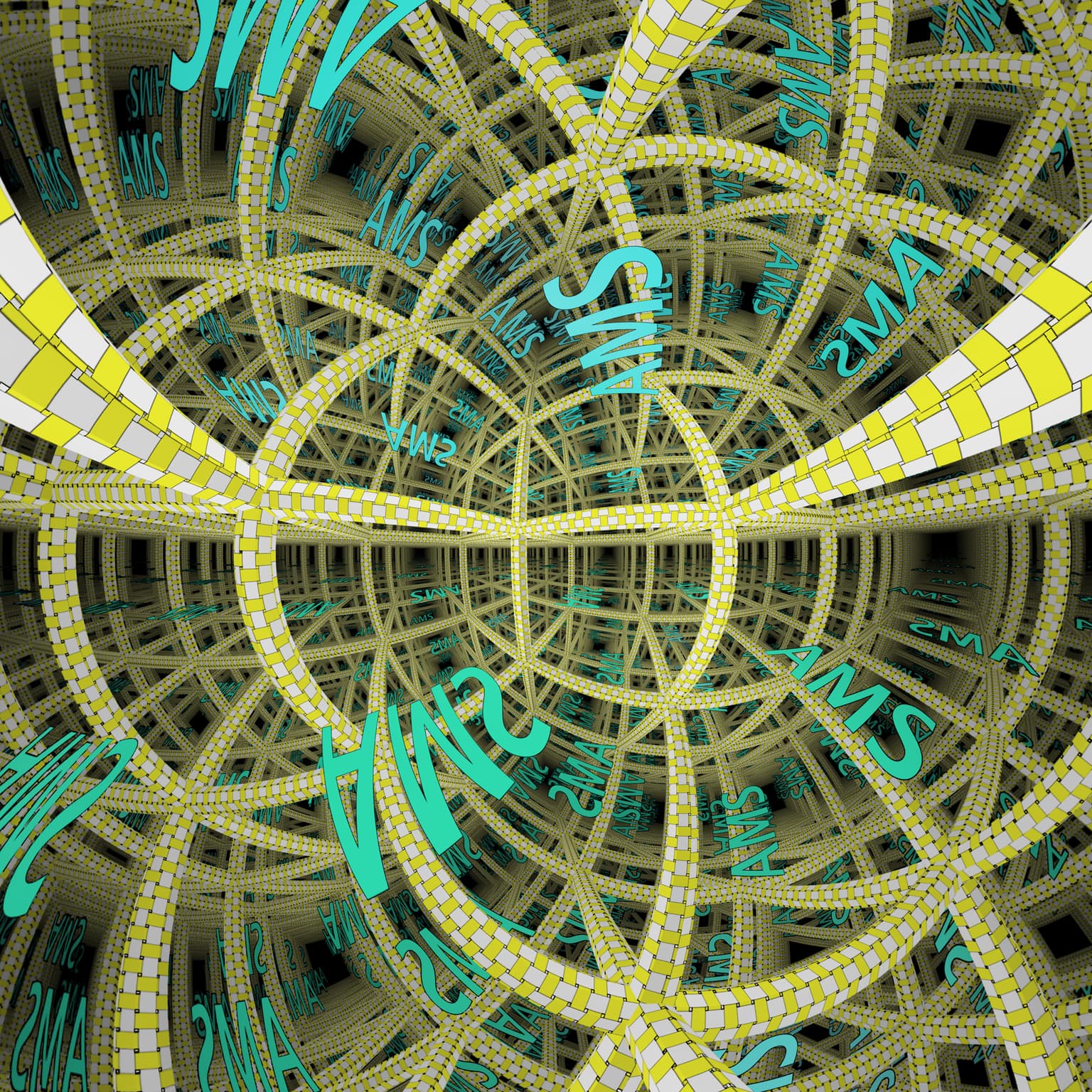
View of the platycosm -a2, decorated with AMS
18" x 18"
Print of computer image
2013