Adrian Dumitrescu
Art could come from anywhere. One just wants to be careful and not overlook it.
It is like when playing tennis: you have to show up at the game if you want to win.
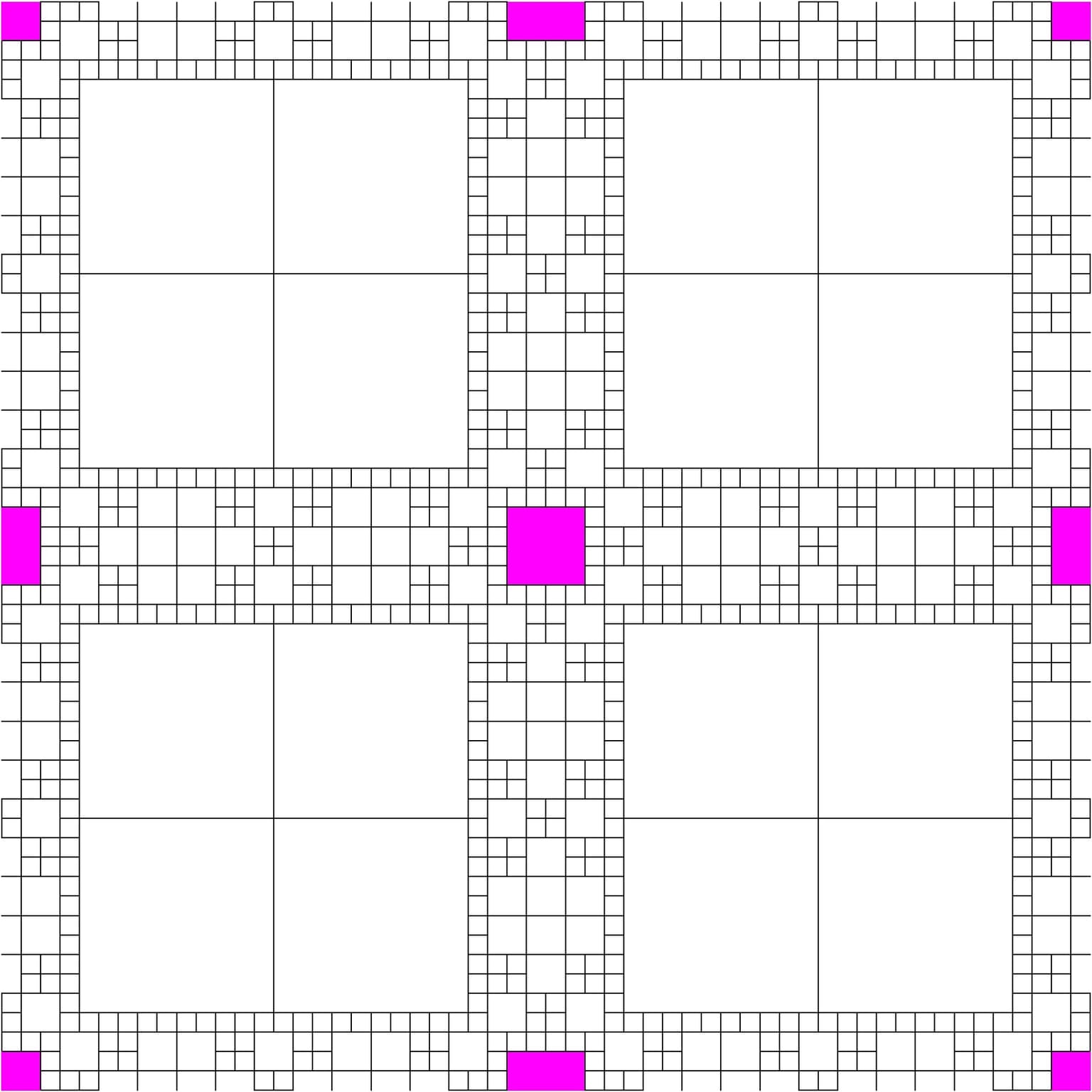
T. Rado conjectured in 1928 that if F is a finite set of axis-parallel
squares in the plane, then there exists an independent subset
I of pairwise disjoint squares, such that I covers at least 1/4 of the area covered by F.
This conjecture was disproved in 1973 by Ajtai. The construction we illustrate here
(2008, by Bereg, Dumitrescu and Jiang) is a refinement of Ajtai's construction
and yields the current record upper bound, 1/4 - 1/384.
What you see is a composite tile made of larger squares of size10,
smaller ones of sizes 1 and 2, and holes (the coloured parts).
By replicating this composite tile one gets a plane tiling where every independent set
covers a fraction of at most 1/4 - 1/384 of the total union area.