Mayer Schwartz

Artists

Mayer Schwartz

Retired Computer Scientist

Portland, Oregon

http://www.mayerschwartzphoto.com

Statement

As a computer scientist, I have long been fascinated with the intersection of art, mathematics, and computer science. Abstract visual artists who have enlightened and inspired me include the Constructivist artists Josef Albers, Ellsworth Kelly, and Piet Mondrian. Recently, I have written software specifically for generating mathematically oriented abstract art. The software uses geometric transformations as its underlying principle, while also allowing for the precise specification of color, texture, and opacity; it has a rich, descriptive input language as well as a high quality rendering engine. This software has enabled me to explore abstract, mathematically oriented art which computer generation makes feasible.

Artworks

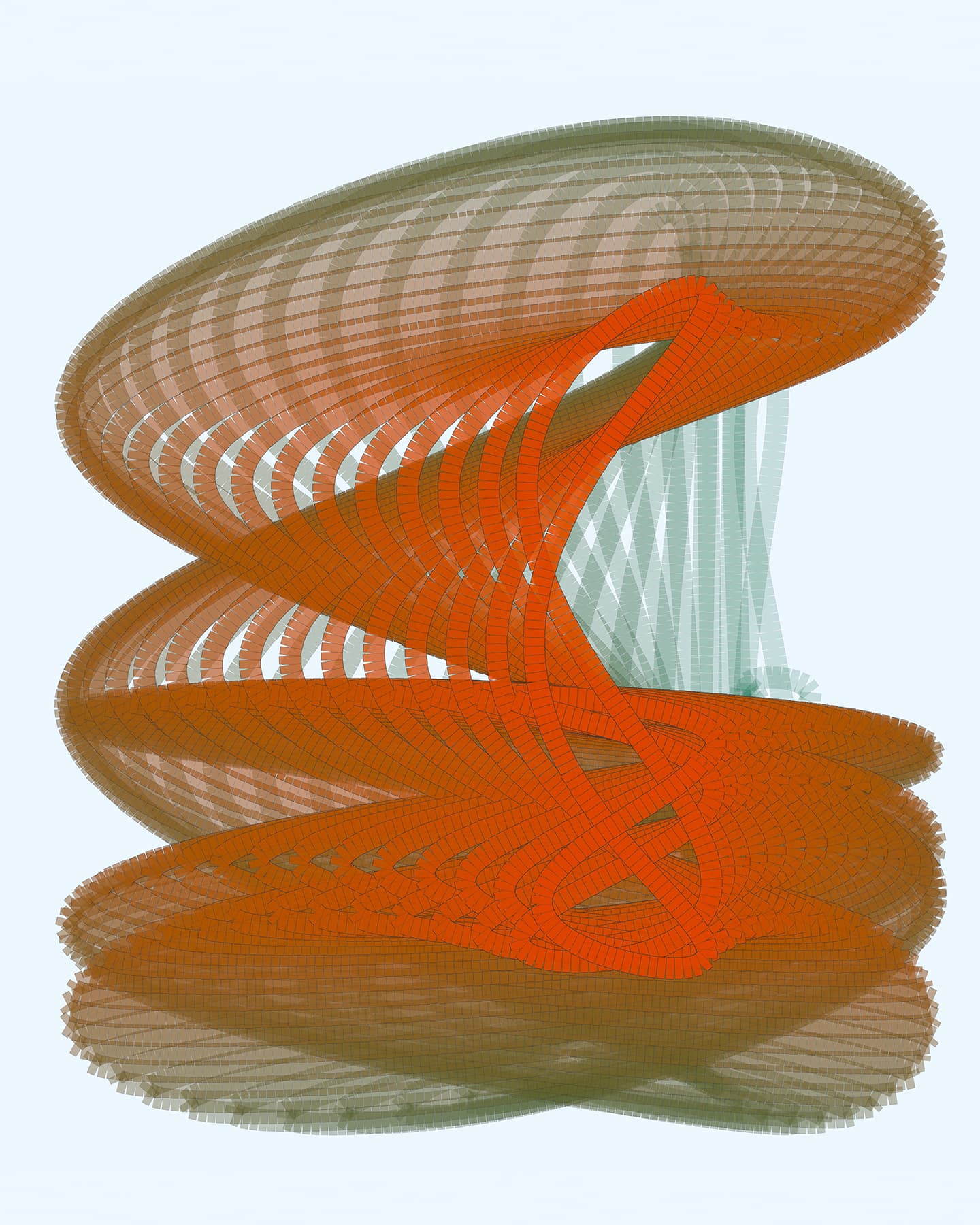
Image for entry 'Perambulation #2' — Perambulation #2
28" x 24" (framed)
pigment inkjet print
2013

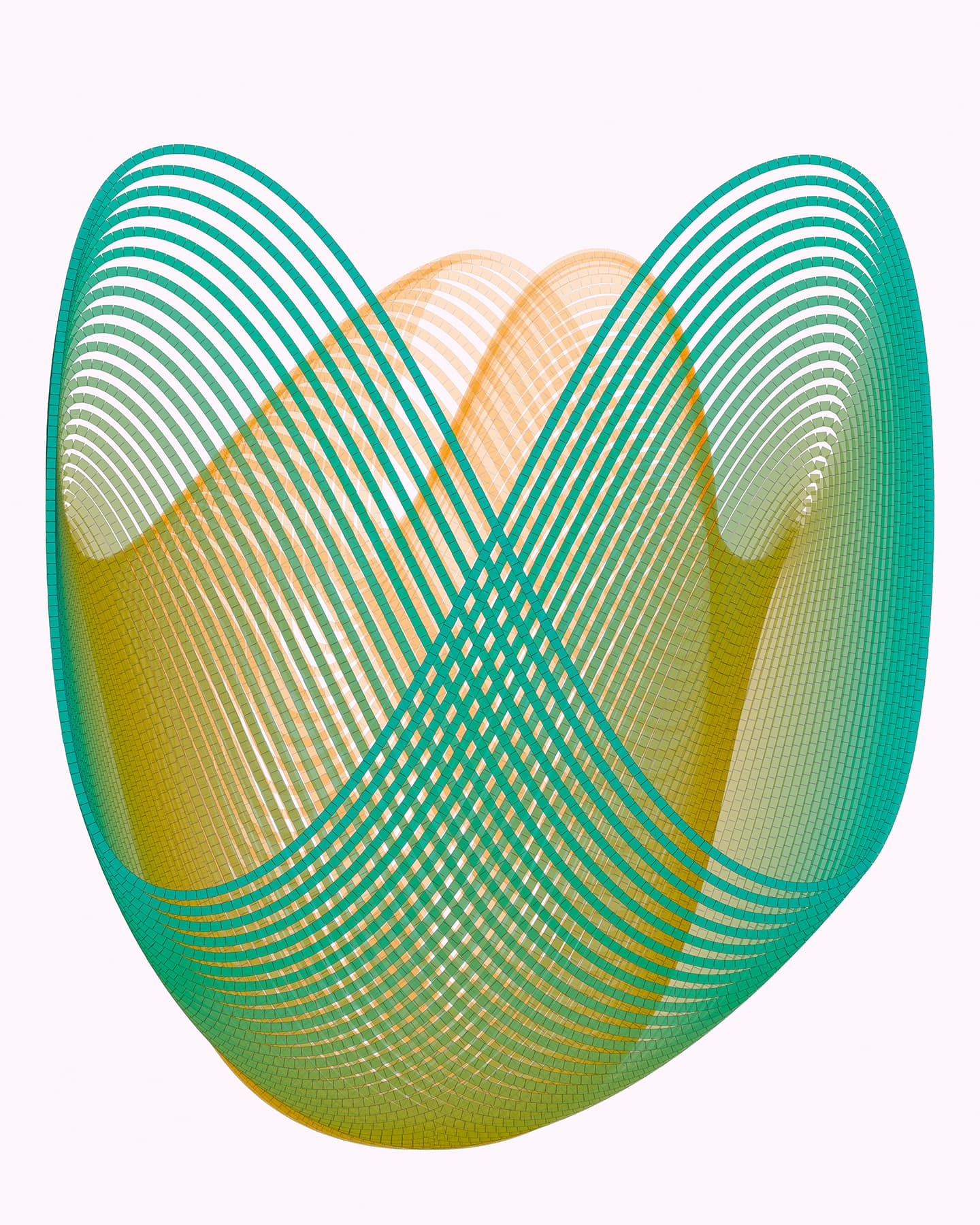
Image for entry 'Perambulation #5' — Perambulation #5
28" x 24" (framed)
pigment inkjet print
2013

I have long enjoyed looking at harmonographs; they were the initial inspiration for "Perambulation #5" and "Perambulation #2." The curve represented in each of these images is defined by parametric equations. For a given distance along a curve from its initial point, the x and y co-ordinates of the corresponding point along the curve, as well as the tangent to the curve, are calculated. This allows for the changing of shape, orientation, color, and opacity as a function of distance along the curve. The number of possibilities and opportunities for aesthetically satisfying results are endless. The program Mathematica has allowed me to rapidly experiment with different parametric curves until a pleasingly shaped curve is uncovered.