Anne Burns
Artists
Anne Burns
Professor Emerita (Mathematics)
Long Island University
Huntington, New York, USA
Statement
I began as an Art major, but became interested in Mathematics and taught Mathematics at Long Island University for 38 years until I retired last year. In the late1980's I attended a talk on Fractals at NYU and I was hooked. After the conference I ran over to the bookstore and bought "The Beauty of Fractals". I bought a home computer whose screen resolution was 200 by 320; it had 3 colors and used floppy disks. I would program my fractals in QBasic and let the program run all night to get one picture. Over the years I learned Fortran, Turbo Pascal, Assembly Language, C++, JAVA and now I use Processing which is great. I am interested in Complex Dynamics, Applications of Complex Variable Theory, Iterative processes and recursion.
Artworks

Tangent Circles I
32 x 32 cm
Digital print
2015
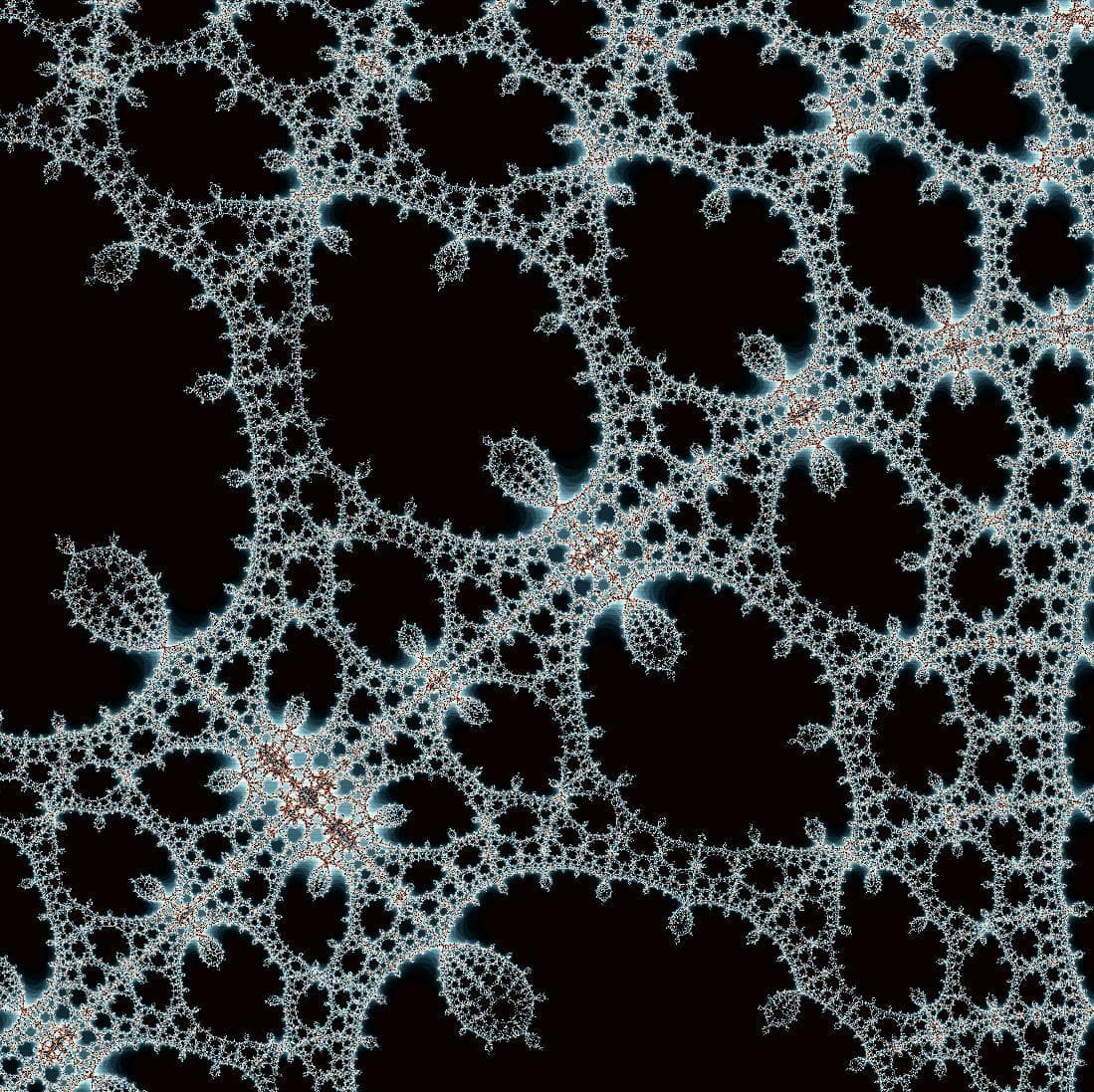
Basilica Zoom
32 x 32 cm
Digital Print
2014
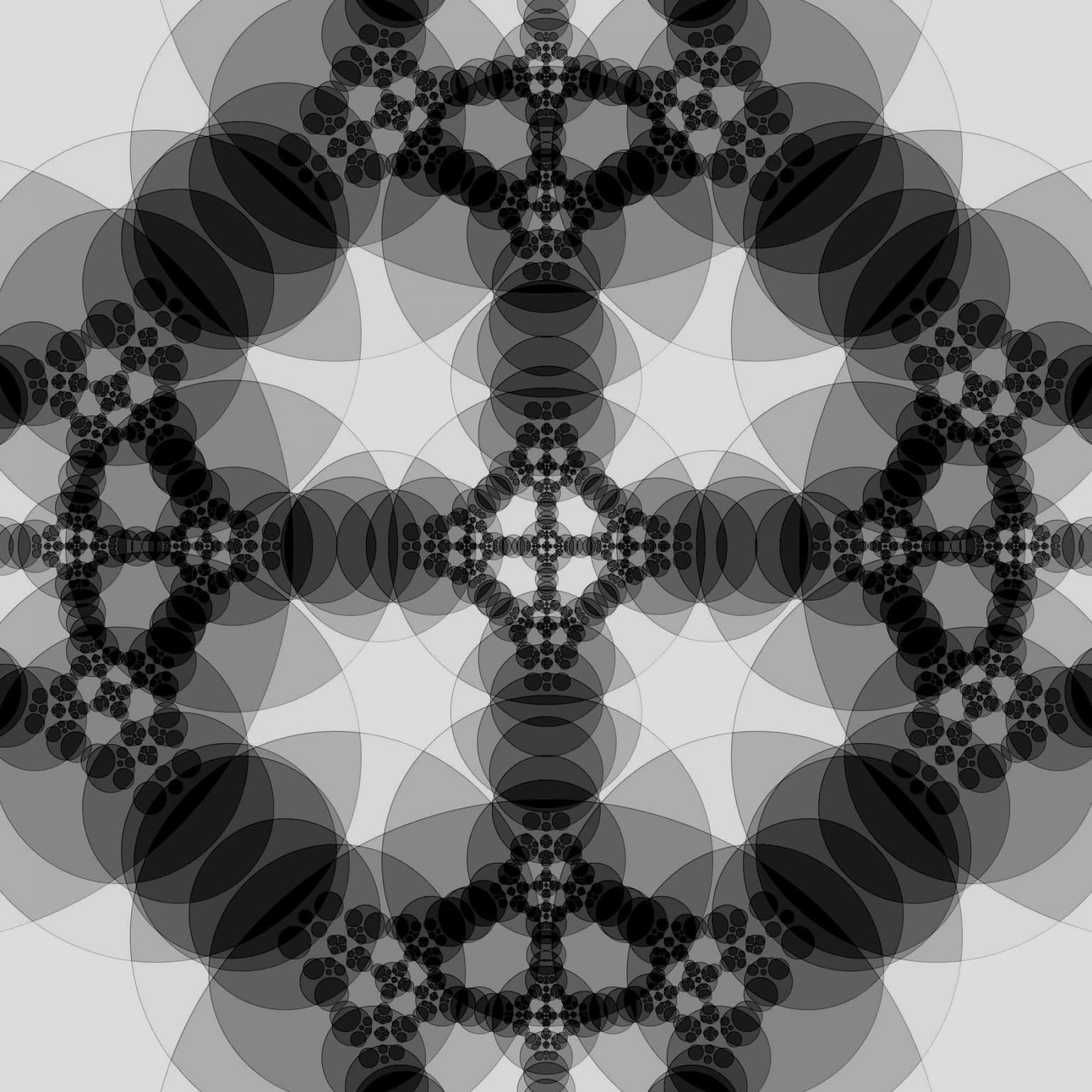
Twenty-five Shades of Grey
32 x 32 cm
Digital Print
2015