Bruce Torrence
Artists
Bruce Torrence
Professor Emeritus of Mathematics
Randolph-Macon College
Ashland, Virginia, USA
Statement
As someone with a passion for the visual arts, I've long been fascinated by tilings, photo-montages, and the ways in which our brains process visual information. As a mathematician, I am familiar with the joy that comes from the hard work of solving a difficult problem. With my art I hope to present viewers with patterns and symmetry from pure mathematics, to convey a sense of wonder in its intricacy and scale, and to challenge viewers to make sense of what they see.
Artworks

Portrait of Tibor Gallai
60 x 60 cm
Archival Inkjet Print
2015
Tibor Gallai (1912-1992), a Hungarian mathematician, was the first to prove that for the "lights out" game on any graph, it is always possible to get from the all-on to the all-off state. This portrait is based on the transition matrix for the lights out game played on the 28x28 grid graph. This matrix is invertible and has order 32,766. It is the smallest grid graph whose transition matrix has such a rich array of distinct powers. The tiles used in the portrait are 28x28 blocks of zeros and ones (represented by white and black pixels) taken from various powers of this transition matrix. Each tile is a symmetric matrix, and has been selected to match both tone and features from the underlying image.
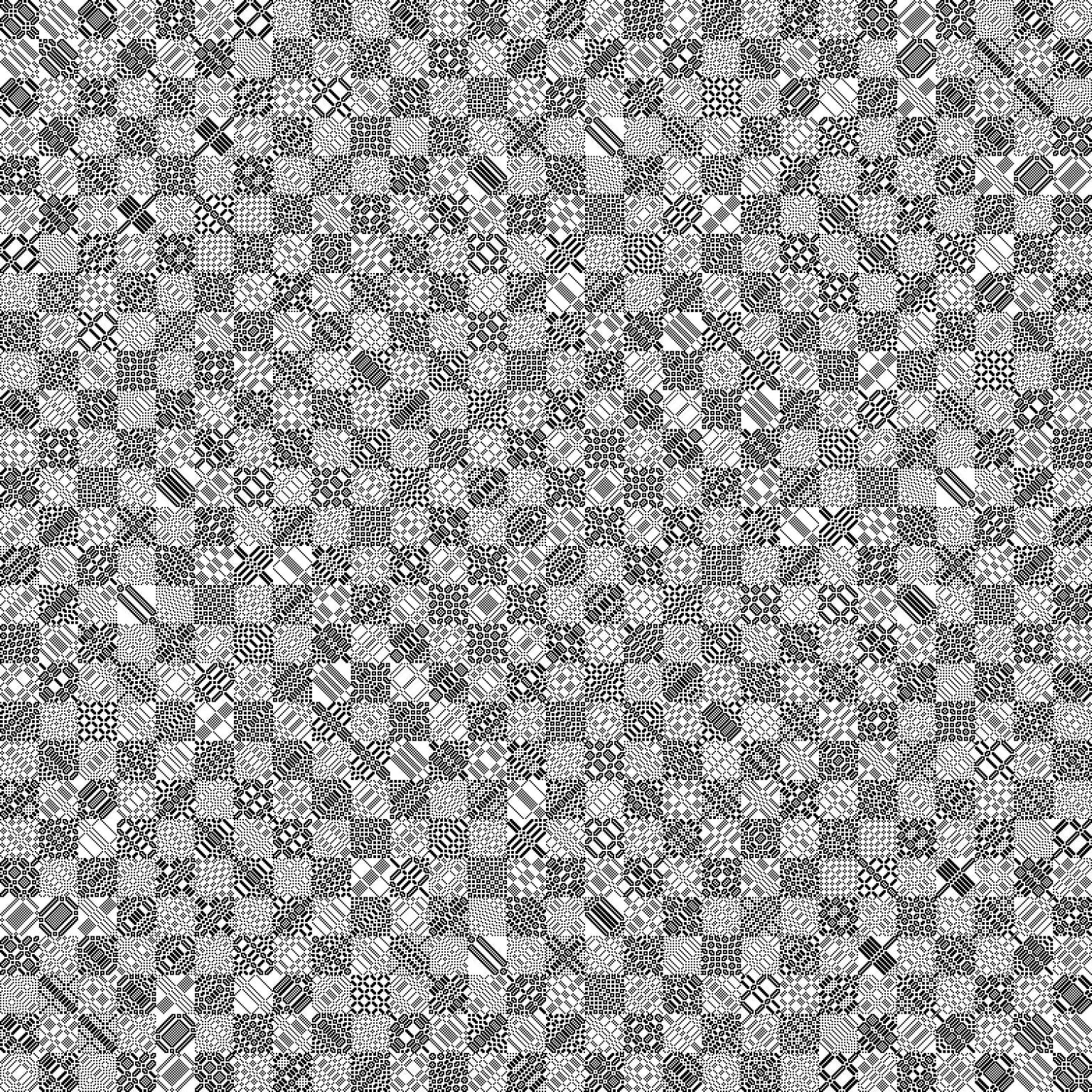
Bit Quilt
60 x 60 cm
Archival Inkjet Print
2015
This is one image from a series of 32,766. The transition matrix for the "lights out" game on a 28x28 grid graph has 28 blocks in each row and column, where each block is itself a 28x28 array of zeros and ones. For this special graph, the transition matrix is invertible and has order 32,766. No smaller grid graph has a matrix with such a rich array of distinct powers. This image shows the 10,101st power of this transition matrix, with ones represented by black pixels, and zeros represented by white. There are no intermediate shades of gray. Despite this simplicity, the viewer is presented with patterns, symmetry, and a glimpse of the vastness of the universe of combinatorial possibilities.