Francesco De Comité
Artists
Francesco De Comité
Associate Professor of Computer Science
University of Sciences Lille (France)
Mouscron (Belgium)
Statement
Manipulation of digital images, and use of ray-tracing software can help you to concretize mathematical concepts. Either for giving you an idea of how a real object will look like, or to represent imaginary landscapes only computers can handle. 3D printing has opened new opportunities to make mathematical objects real; I am just beginning to explore this new world.
Artworks
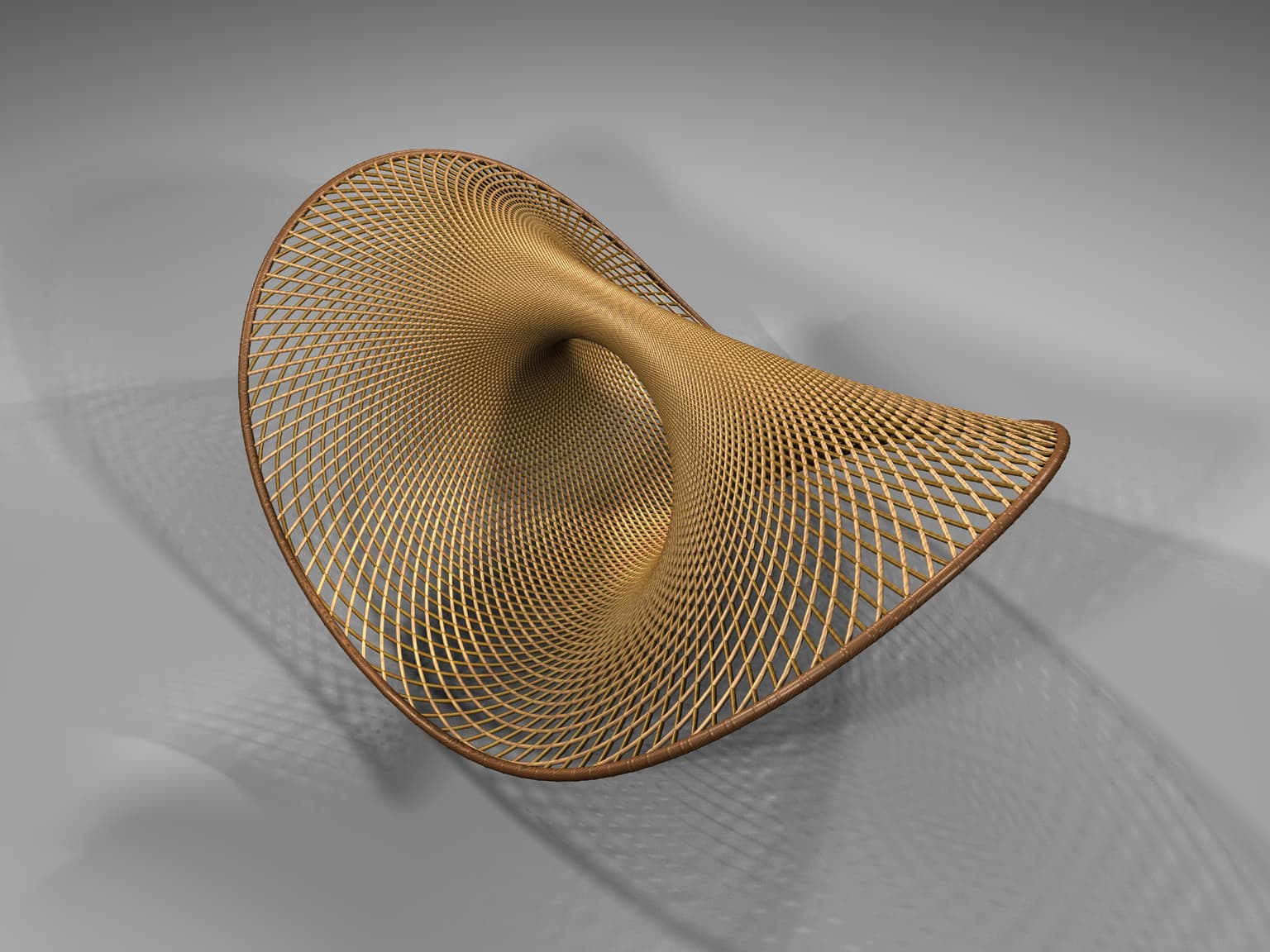
Young King Agamemnon's Rocking Horse
60 x 80 cm
Digital print on cardboard
2014
Cyclid Islands fishermen were famous in Ancient Greece for their science of weaving fishtraps and baskets. Living under the protection of King Atreus and Queen Aerope of Mycenae, they put all their knowledge in building a very special rocking horse for their first son, the future king Agamemnon.
Recent mathematical studies reveal that this toy, as all other examples of Cyclid islands weavers artworks, are in fact Dupin cyclids, defined with their four families of circles.
This particular model is a part of an infinite cyclid, limited by a bounding sphere.
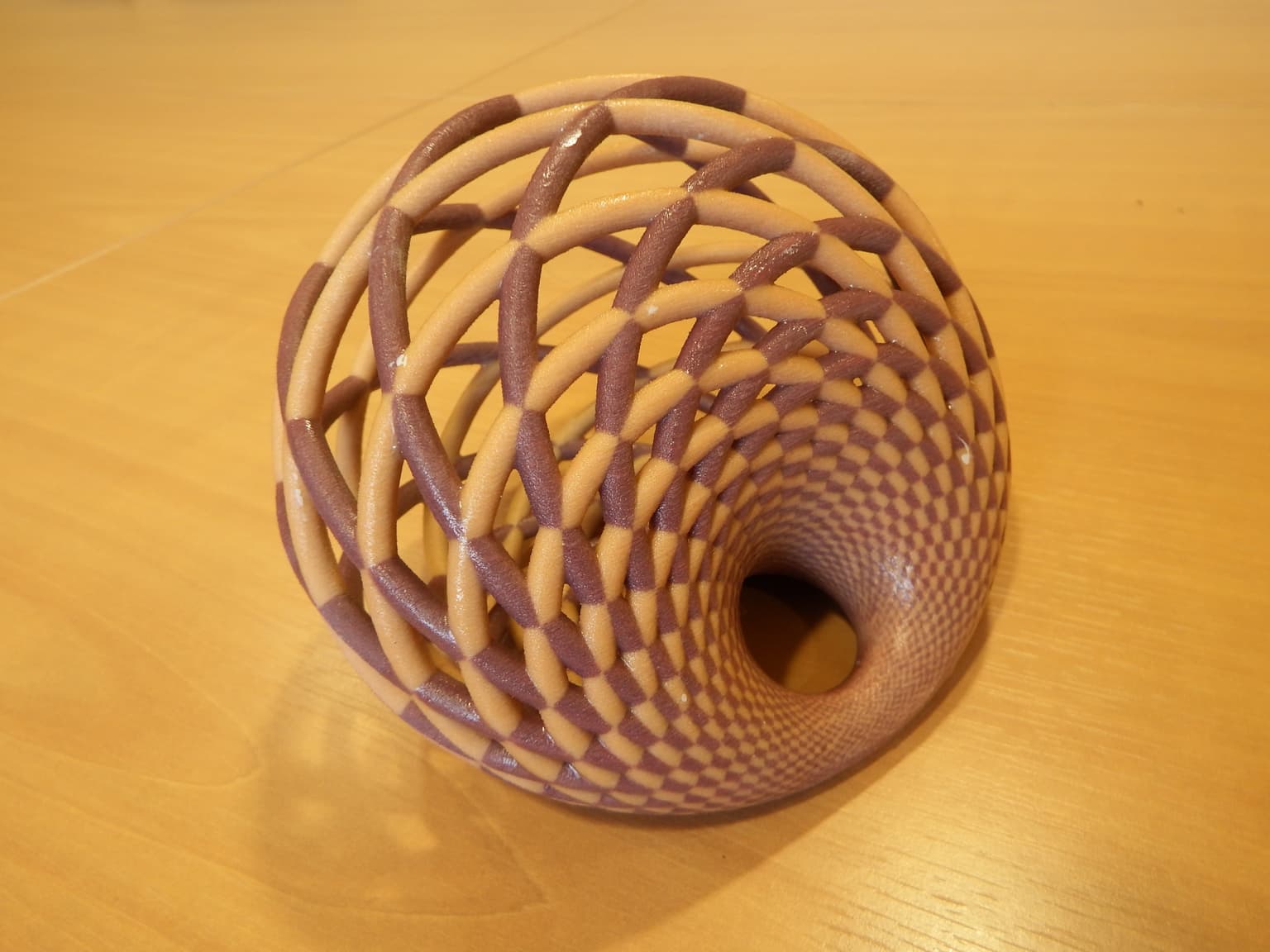
Cyclid islands Ritual Weaved Basket
15 x 20 x 20 cm
3D Print (Full color sandstone)
2014
Dupin cyclids are images of tori under sphere inversion. Since inversion preserves circles, cyclids contain four families of circles, like tori : parallels, meridians and images of Villarceau circles. This model is build using only both families of Villarceau circles.

Cyclid Islands Fish Trap
15 x 20 x 20 cm
3D Print (Full color sandstone)
2014
It is not evident, at first sight, that this object, another Dupin cyclid, is made only from circles. The inside part, when seen from a short range is quite fascinating. That is what makes 3D printing interesting : the ability of manipulating objects, and find unexpected perspectives.