Jean Constant

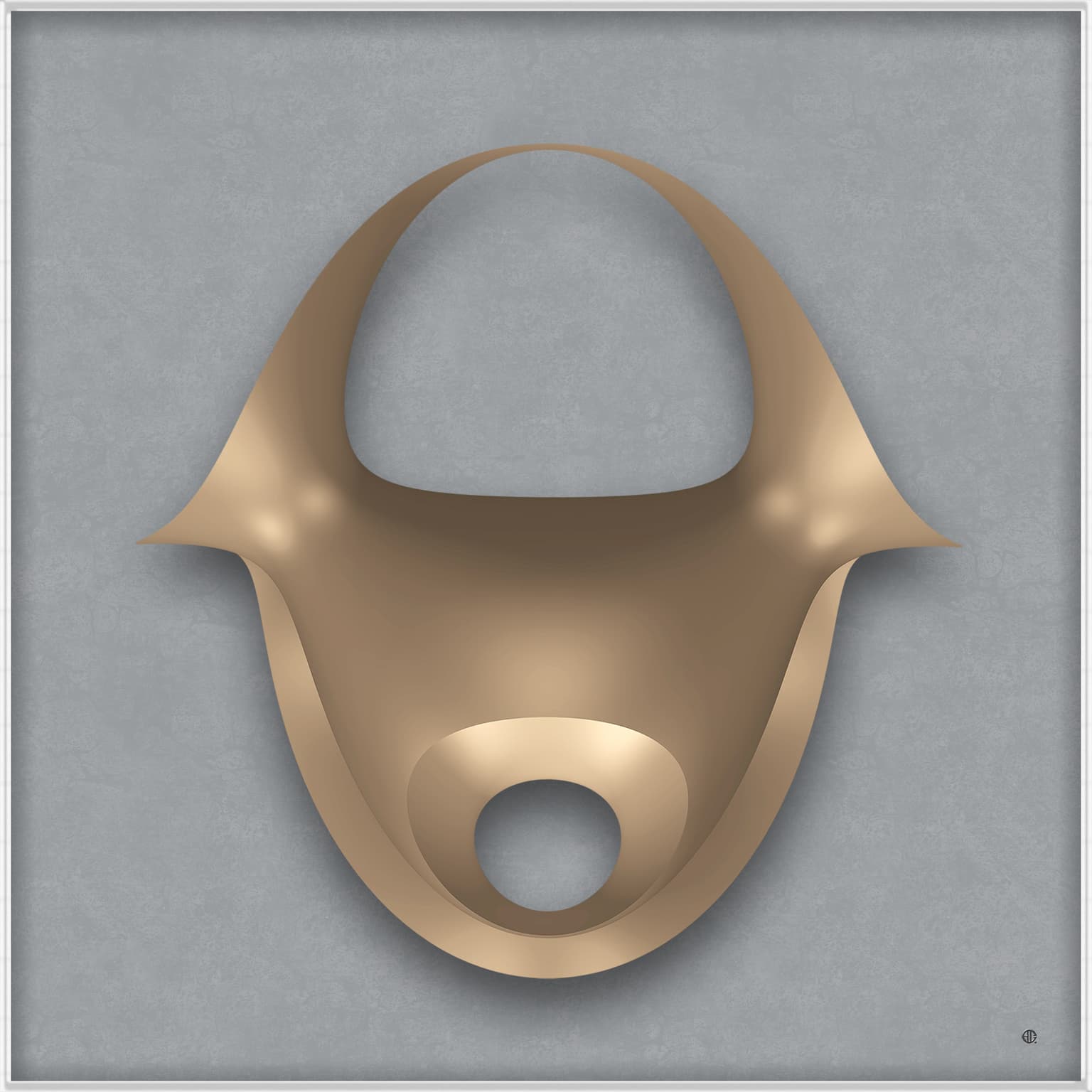
A Cayley's cubic surface is a unique cubic surface having four ordinary double points. The Cayley cubic is invariant under the tetrahedral group and contains exactly nine lines, six of which connect the four nodes pairwise and the other three of which are coplanar. The original image outline was created with the MFO–Surfer program, using the following formula: (b-0.5)(x+y+z-1)^2+(a-0.5)(x+y+z+3)^2+x^3+y^3+z^3+1-9*(x+y+z+1)^1=0

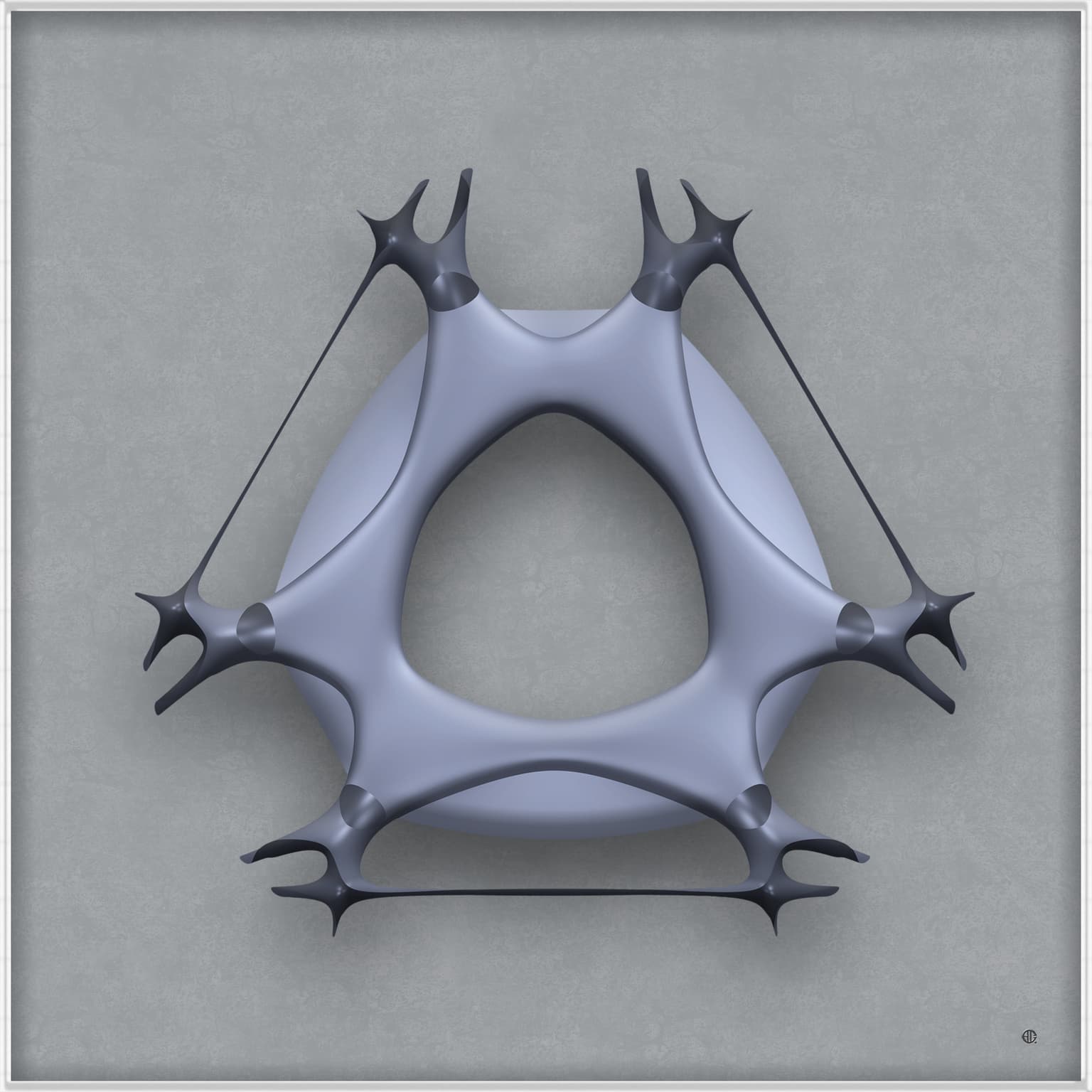
Algebraic surfaces can be smooth, or they can have several sharp peaks, known as isolated singularities. In the Euclidean plane in Degree 7: they have 3 (−1)-curves. Calculating in finite fields has countless applications, in particular coding theory and cryptograph. In 2004, Oliver Labs constructed a surface of degree 7 (a septic) with 99 singularities. This image was originated in the MFO-Surfer program after the following equation: x^9-1136x^7y^2+126x^5y^4-1184x^3y^6+9xy^8+64z^9-119x^8+126x^6y^2-11126x^2y^6+9y^8+27x^7-1127x^5y^2-11135x^3y^4-1181xy^6-11144z^7-1121x^6-11225x^4y^2+45x^2y^4-1139y^6-1136x^5+72x^3y^2+108xy^4+108z^5+54x^4+108x^2y^2+54y^4+9x^3-1127xy^2-1130z^3-1127x^2-1127y^2+2.25z+4=0

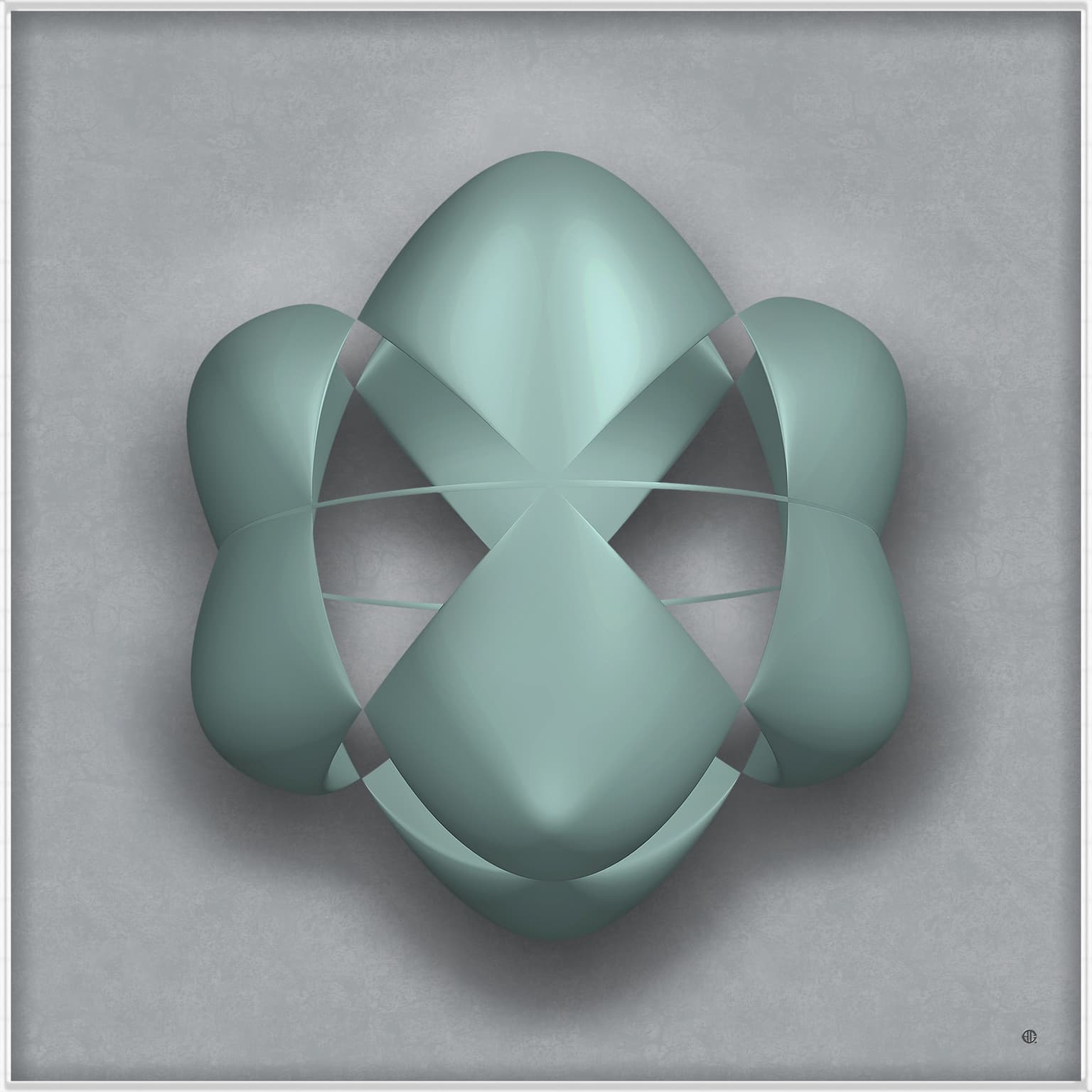
The Barth-sextic is a sextic surface in complex three-dimensional projective space having the maximum possible number of ordinary double points. The surface was discovered by W. Barth in 1994. The outline was created in the MFO-Surfer program after a variation on the original equation: 2((a(3+sqrt(5))/7)^11x^2-1y^2)((a(1+sqrt(5))/2)^2y^2-1z^2)((a(1+sqrt(5))/2)^2z^2-1x^2)-1(1+2(a(1+sqrt(5))/2))(x^2+y^2+z^2-1*1)^2=0