Bih-Yaw Jin & Chia-Chin Tsoo
Artists
Bih-Yaw Jin
Professor
Department of Chemistry, National Taiwan University
Taipei, Taiwan
Statement
Tubular bugle beads are the perfect material for constructing the pin-jointed truss models of many polyhedral complexes consisting of rigid building blocks such as tetrahedra and octahedra. We presented two examples, the Sierpinski tetrahedron and the frequency-4 Mackay polyhedron, at the 2014 Bridges conference in Seoul. Interestingly, the great majority of structures of inorganic substances can be described by the linking of several basic coordination polyhedra: tetrahedron, octahedron and icosahedron. This time, we are providing another two examples.
Artworks

The perovkite structure
16 x 16 x 16 cm
Tubular glass beads
2014
The crystal structure of a perovskite can be viewed as a 3D uniform space-filling tesselation of octahedrons and cuboctahedrons in a ratio of 1:1. We used two different colors of tubular bugle beads to construct a 2x2x2 bead model of the perovskite structure.
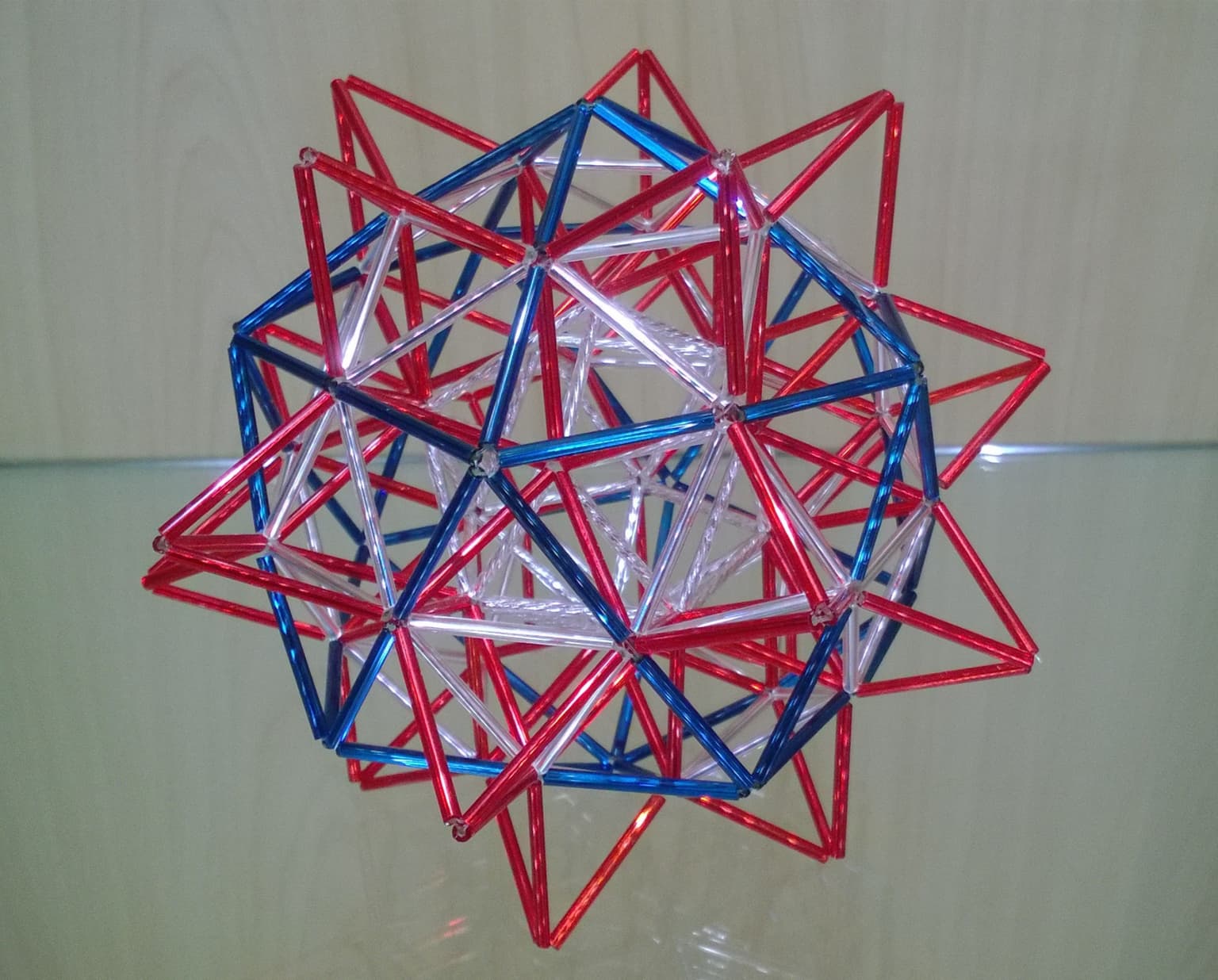
Truss models of icosahedral complexes
12 x 12 x 12 cm
Tubular glass beads
2014
A quasiperiodic crystal is a structure that is ordered but not periodic due to the incompatibility between the five-fold rotational symmetry and the translational symmetry. Here we have built an icosahedral complex, which can be considered as a finite quasicrystal, by adding sufficient numbers of tetrahedra, octahedra, and pentagonal bipyramids around the central icosahedron. The colors of beads are chosen to depict four different polyhedra with the icosahedral symmetry, namely, an icosahedron (beads with spiral pattern), an icosidodecahedron (white), a frequency-2 Mackay dodecahedron (blue), and a hexecontahedron (red).