Frank A Farris
Artists
Frank A. Farris
Professor of Mathematics and Computer Science
Santa Clara University
San Jose, CA
Statement
My artistic impulse is to let the beauty of the real world shine into the realm of mathematical patterns. My method combines photographs with complex-valued functions in the plane to create images with all possible types of symmetry: Euclidean, hyperbolic, and spherical symmetries (as these act on the plane). For some works, I then transfer plane images back to the sphere. All these methods are explained in detail in my book Creating Symmetry: The Artful Mathematics of Wallpaper Patterns, published in 2015 by Princeton University Press.
Artworks
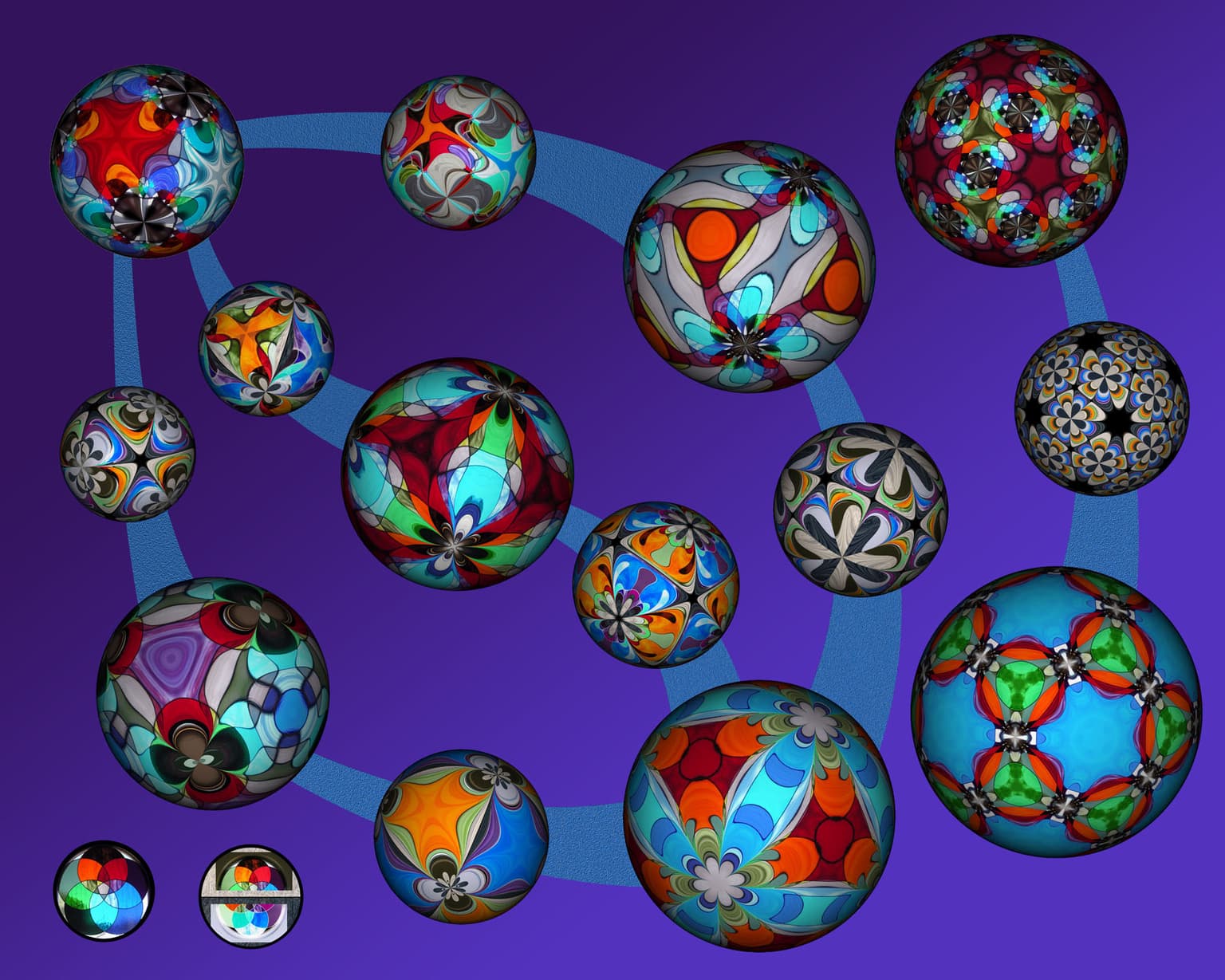
A panorama of fourteen imaginary planets illustrates all possible types of polyhedral symmetry and antisymmetry. The seven largest globes show patterns invariant under the groups that preserve the Platonic solids, with the three large globes along the top showing the three groups of rigid motions of the tetrahedron, the cube, and the icosahedron. (Swirling shapes occur when mirror symmetry is not present.) Other large globes show mirror possibilities. Two large globes are connected with a blue path when one group is a normal subgroup of the other. The small globe in that path illustrates the color-reversing pattern type that this group condition allows. All globes are colored with the same stained glass window or its negative.

A wallpaper pattern for the Poincaré Upper Halfplane is colored with a photograph of a peach and its negative. As a consequence of the “shrinking ruler” geometry in this non-Euclidean universe, all the similarly painted shapes are exactly the same size. For instance, the light triangles with bright blue bobs at the corners are actually centers of 3-fold rotational symmetry. And there are impossibly infinitely many of them: all the same size, all the way down. Likewise, all the light fan shapes that spring up from the edge, though they may seem larger and smaller, consist of infinitely many perfectly congruent arms. From a mathematical point of view, this image depicts a function invariant under the modular group.