2015 Joint Mathematics Meetings
Francesco De Comité
Artists
Francesco De Comité
Associate Professor of Computer Science
University of Sciences Lille (France)
Mouscron (Belgium)
Statement
Manipulation of digital images, and use of ray-tracing software can help you to concretize mathematical concepts. Either for giving you an idea of how a real object will look or to represent imaginary landscapes only computers can handle. Things become yet more interesting, when you can transform your two-dimensional dream objects in real three dimensional sculptures. You can then handle your creations, and look at them from an infinity of view angles.
Artworks

When Objects Fall Out From the Frames
12*12*12cm
Color sandstone 3D printing
2014
The three dimensional version of a virtual image is much more fascinating than the original picture; it is like having in your hands an infinity of different versions of the object, at no cost.
Now, new technics let you use colours in your 3D prints. This makes it yet more interesting: you can try to reproduce the virtual object in real world: a kind of inverted "Trompe l'oeil"...
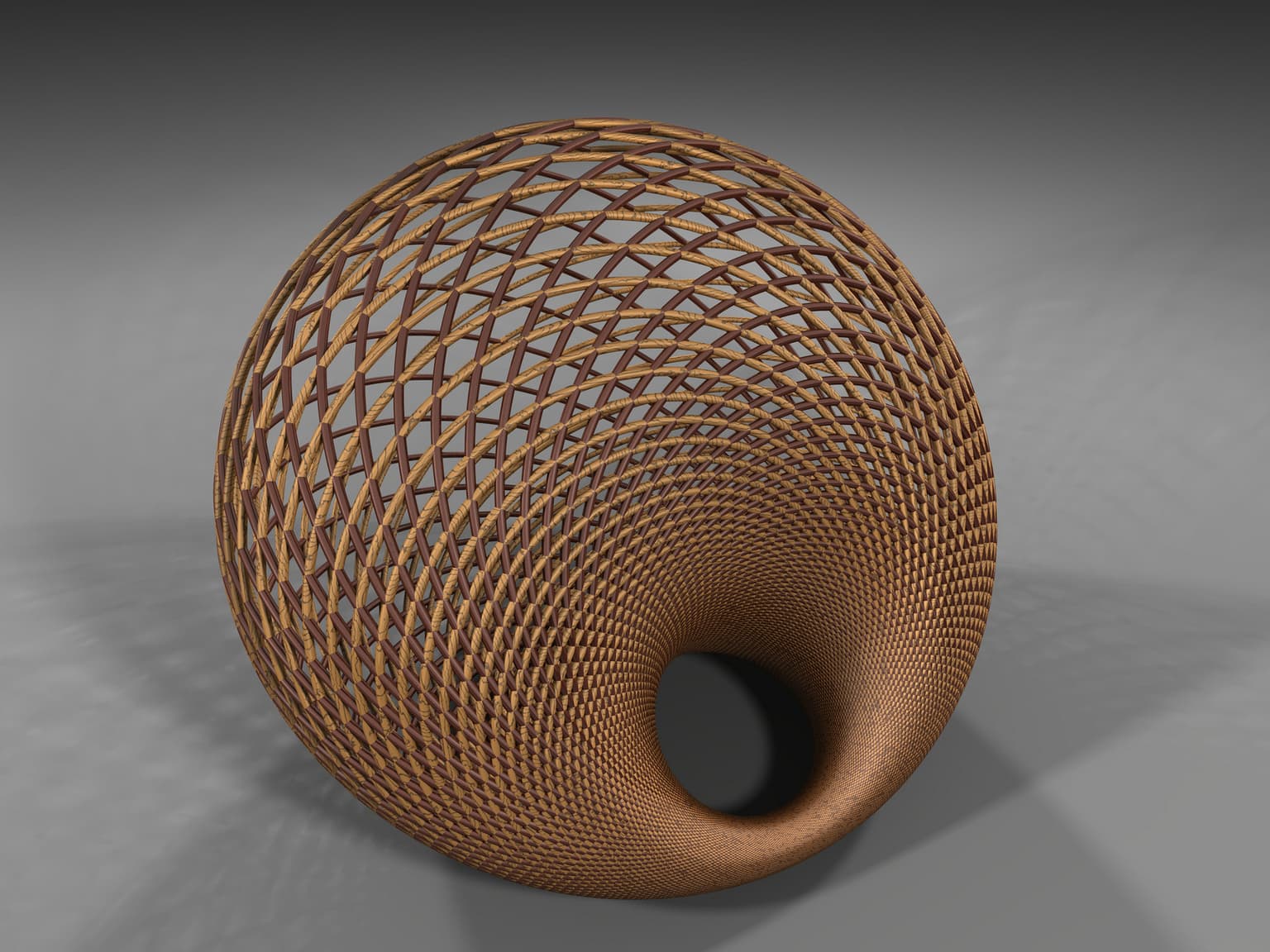
The Forgotten Art of Cyclides Islands Fishermen Fish-Trap Weaving
60*80cm
Digital print on cardboard
2014
Adding more Villarceau-like circles makes the shape of the cyclide itself more visible, but we then loose the color contrast between the two set of circles. As usual, one has to find a compromise between those two ways of considering the mathematical concept.
Anyway, itis still funny to find titles...

The Lost Art of Cyclides Islands Weavers
60*80cm
Digital print on cardboard
2014
Dupin cyclides are the images of tori by sphere inversion. Since sphere inversion preserves circles, the set of Villarceau circles one can draw on a torus is transformed in a set of circles on the cyclide.
The game is then to find nice images illustrating this fact, together with some story 'à la Raymond Roussel' to reinforce the magic.