2016 Bridges Conference

Dmitri Kozlov

Artists

Dmitri Kozlov

Architect, scientist

Research Institute of Theory and History of Architecture and Town-planning, Russian Academy of Architecture and Building Sciences

Moscow, Russian Federation

kozlov.dmitri@gmail.com

Statement

Knots have been the subject of traditional art since ancient time. Woven patterns and ornaments, stone and wood carving of knots were popular throughout the world. My approach is based on the idea that cyclic periodic knots made of resilient filaments like steel wire or fiberglass behave as kinetic structures of topological surfaces. Knots tied with such materials must have a large number of physically contacting crossings that form vertices of the surfaces. The crossings slide along the filaments which twist around their central axes. The waves on the filaments move and change their lengths to adapt to desired spatial disposition of the contact crossings. The complicated knots of this type I designated as NODUS-structures.

Artworks

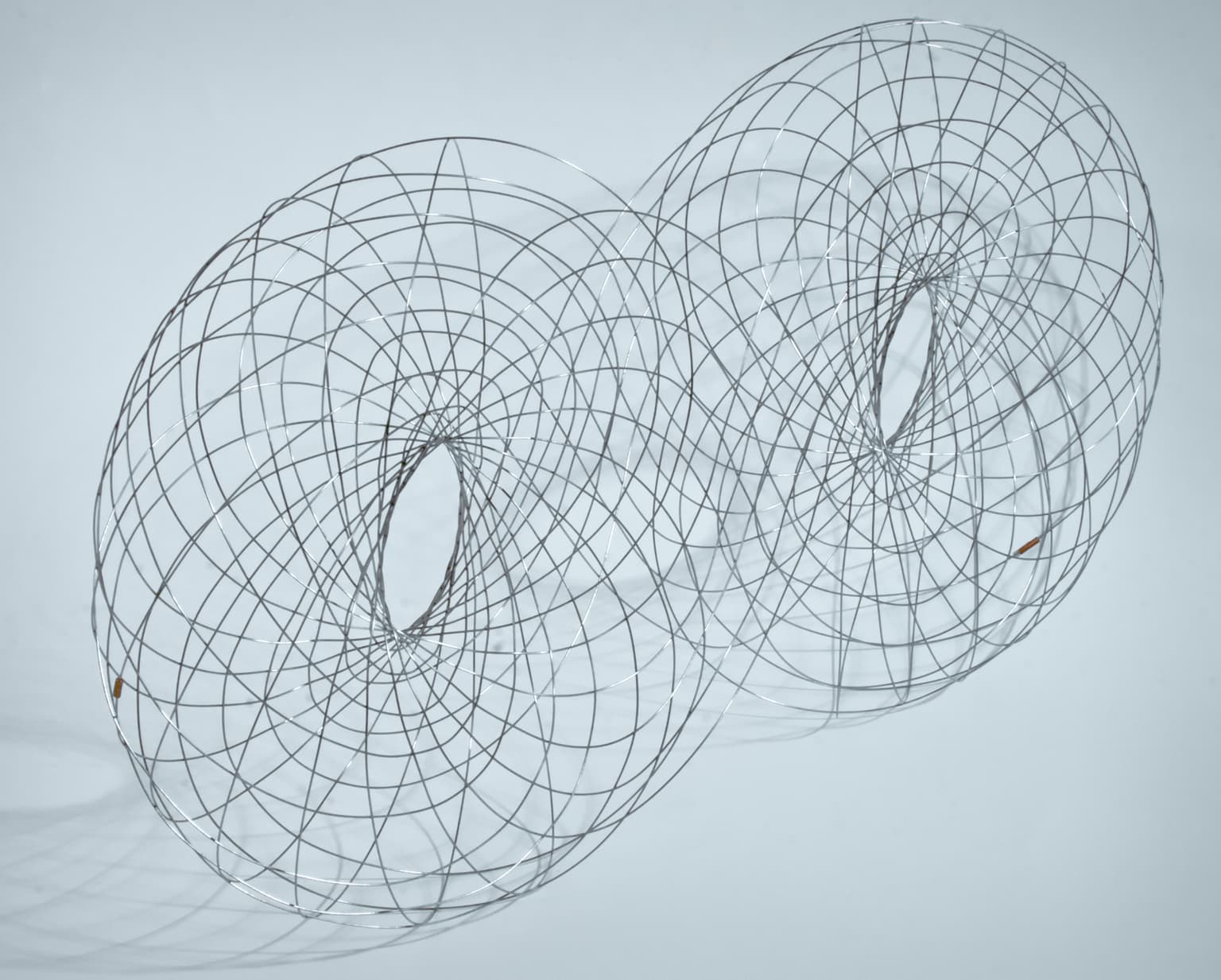
Image for entry 'Pretzel NODUS-structure or "Tissue of Space-Time"'

Pretzel NODUS-structure or "Tissue of Space-Time"

40 x 60 x 20 cm

steel wire

2016

Torus and pretzel knots are the knots that can be tied on the surfaces of tori and pretzels. The flower-like shapes of torus knots and similar to them alternating Turk's Head knots are very popular in different fields of math-art both traditional and modern. At the same time pretzel knots and corresponding to them alternative knots are chosen by math artists quite rarely. Here I present the linkage of two pretzel knots that form left and right windings on a pretzel surface with two holes as an example of my NODUS-structures. I dedicate this my work to the recent discovery in physics, namely detecting of gravitational waves. My woven pretzel surface may serve as a mechanical model of spreading of g-waves through the space-time tissue.