2016 Joint Mathematics Meetings
Douglas G. Burkholder
Artists
Douglas G. Burkholder
Professor of Mathematics
Lenoir-Rhyne University
Hickory, North Carolina, USA
Statement
Long-long ago in a place far-far away, my love of art was placed on the back burner as science and mathematics consumed my life. My love for geometry and my desire for visualization for the sake of mathematical understanding have always been central to my teaching and to comprehension of mathematical concepts. However, only decades later, have I begun to explore the artist within and attempted to merge art with my mathematics. Recent work involves searching for beauty and patterns within Conway and Radin’s non-periodic Pinwheel Tiling of the plane with 1 by 2 right triangles.
Artworks
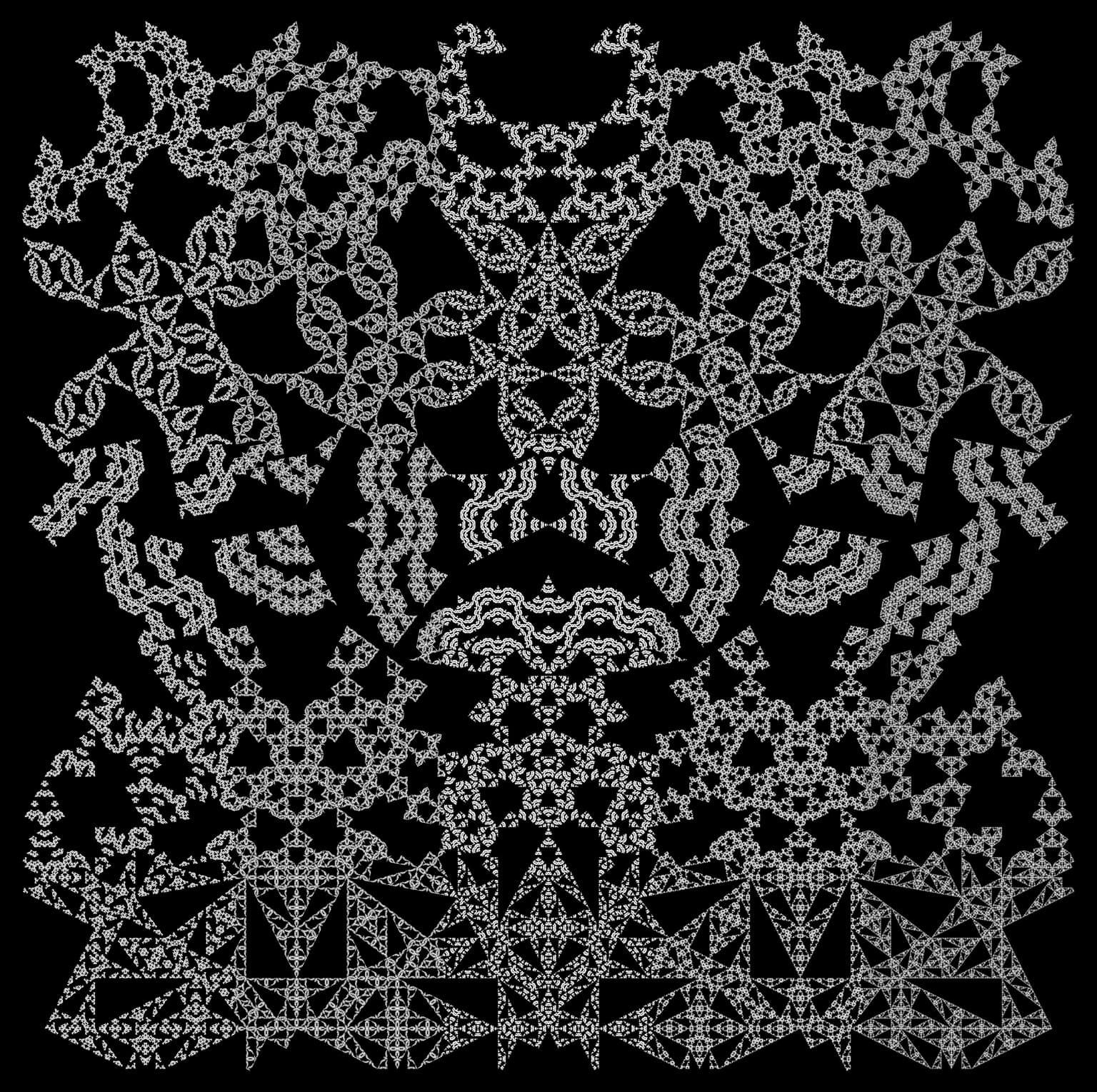
A Radin-Conway Pinwheel Lace Sampler
50 x 50 cm
Digital Print
2015
This artwork evolved from a search for beauty and patterns within Conway and Radin’s non-periodic Pinwheel Tiling of the plane by 1x2 right triangles. The Pinwheel tiling can be created by repeatedly subdividing every triangle into five smaller triangles. This lace resulted from alternately subdividing triangles and removing triangles. Triangles are removed based upon their location in the next larger triangle. First, on the macro level, the five distinctive removal rules are applied one to each row. This removal rule is especially easy to see in the bottom row. These same five rules are then applied, on the micro level, to the columns. The remaining triangles form a sampling of twenty-five styles of lace generated by the Pinwheel tiling.
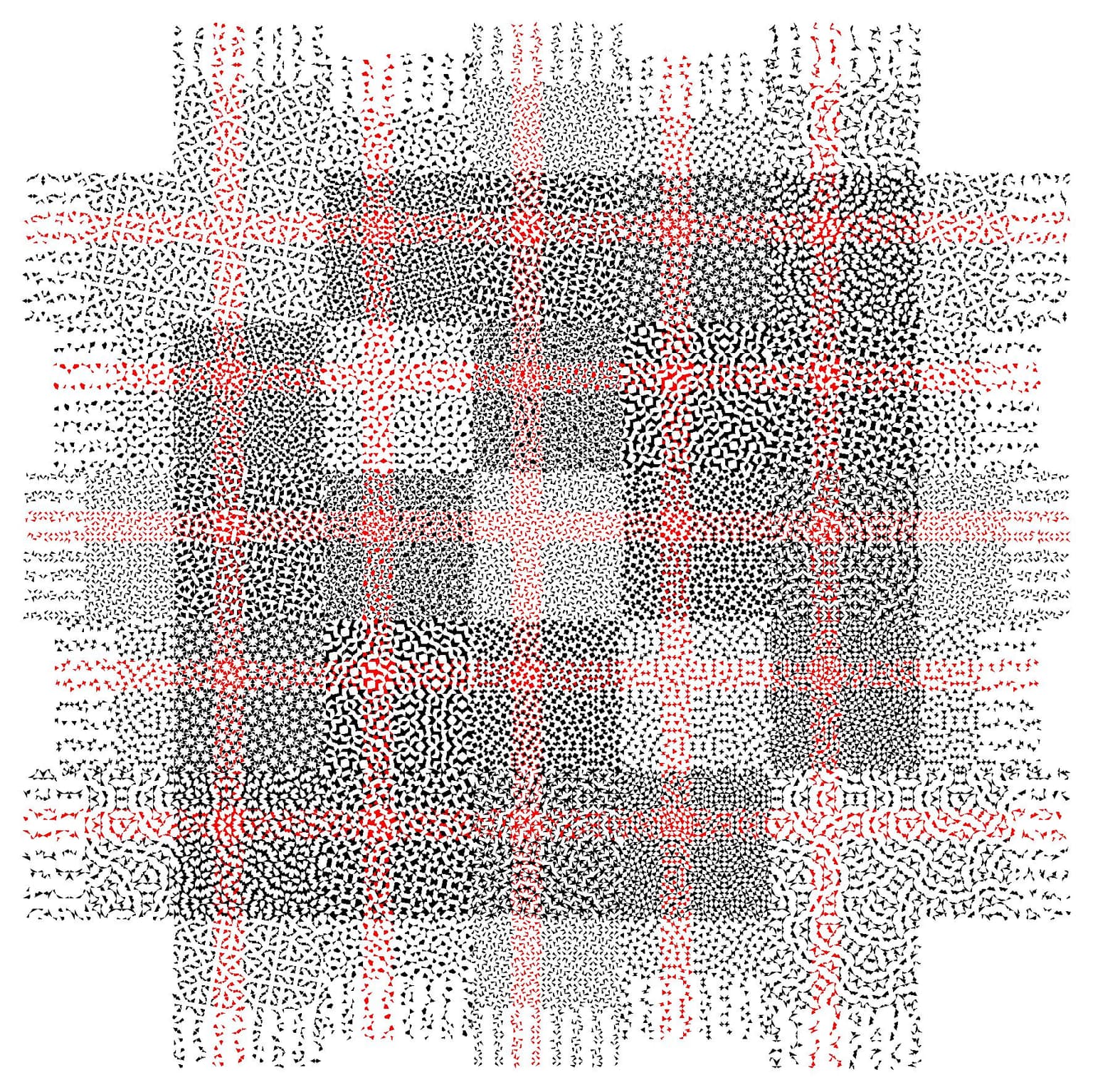
A Radin-Conway Quilt
50 x 50 cm
Digital Print
2015
A Radin-Conway Quilt starts by cutting a square with fringe containing almost 1,000,000 triangles from Conway and Radin’s non-periodic Pinwheel Tiling of the plane. Next, we paint about 30% of the triangles by dividing the square into five rows and five columns. A triangle is painted depending only upon its relative location in the next larger triangle of the tiling procedure and upon its row and column. Thus all triangles located in position 1 of their larger triangle are painted in the first row and are painted in the first column, etc. Thus, exactly two out of every five triangles is painted in each of the rectangles of intersections of distinct rows and columns. This provides all 30 combinations of painting the five types of tiles.