2016 Joint Mathematics Meetings

Doug Dunham

Artists

Doug Dunham

Professor Emeritus of Computer Science

Dept. of Computer Science, University of Minnesota Duluth

Duluth, Minnesota, USA

ddunham@d.umn.edu

https://www.d.umn.edu/~ddunham/

Statement

The goal of my art is to create aesthetically pleasing repeating hyperbolic patterns. One way to do this is to place patterns on (connected) triply periodic polyhedra in Euclidean 3-space. These polyhedra are considered to be hyperbolic since the sum of the angles around each vertex is greater than 360 degrees. The first polyhedron below is decorated with a fractal circle pattern. The second and third polyhedra are decorated with motifs inspired by M.C. Escher. There is a two-step connection between these latter two polyhedra and hyperbolic plane patterns: (1) they approximate triply periodic minimal surfaces, and (2) those surfaces have the hyperbolic plane as their universal covering surface.

Artworks

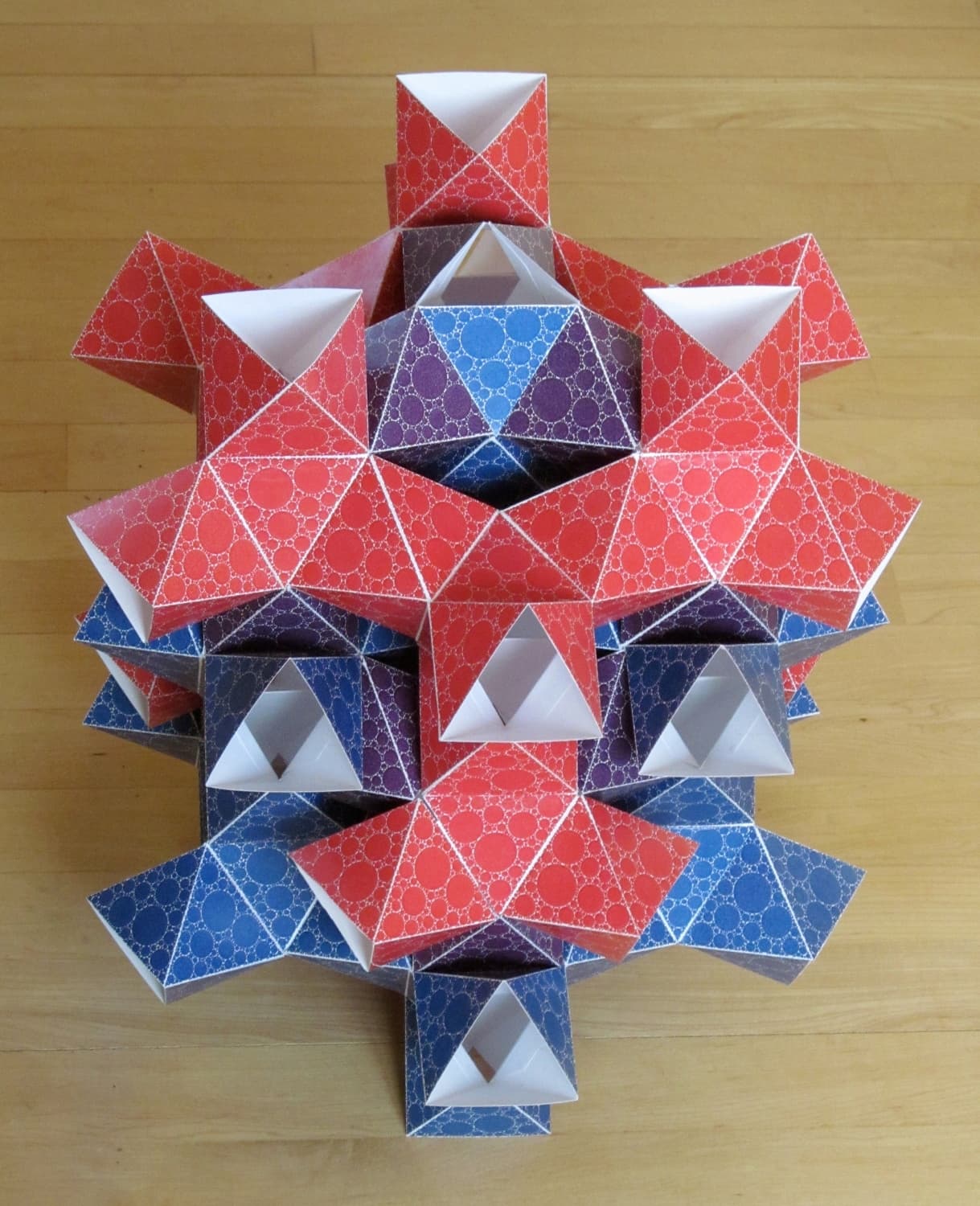
Image for entry 'A Fractal Circle Pattern on the {3,12} Polyhedron'

A Fractal Circle Pattern on the {3,12} Polyhedron

50 x 30 x 30 cm

Printed Cardboard

2015

This polyhedron is constructed by placing regular octahedra on all the faces of another such octahedron, so there are 12 equilateral triangles about each vertex. Each of the triangular faces has been 90% filled by a fractal pattern of circles provided by John Shier. The polyhedron consists of red and blue "diamond lattice" polyhedra and purple octahedra that connect the red and blue polyhedra. Each of the red and blue polyhedra consists of octahedral "hubs" connected by octahedral "struts", each hub having 4 struts projecting from alternate faces. The red and blue polyhedra are in dual position with respect to each other - they form interlocking cages. Each purple connector has a red and a blue octahedron on opposite faces.