2016 Joint Mathematics Meetings
Robert Fathauer
Artists
Robert Fathauer
Owner
Tessellations
Apache Junction, Arizona, USA
Statement
I'm endlessly fascinated by certain aspects of our world, including symmetry, chaos, and infinity. Mathematics allows me to explore these topics in distinctive artworks that I feel are an intriguing blend of complexity and beauty. The laws that govern our physical universe can be succinctly expressed by mathematical equations. As a result, mathematics can be seen throughout the natural world, and much of my work plays on mathematical forms in nature.
Artworks

This piece combines a ceramic polyhedral form with organic fingers that are formed from willow branches. The polyhedron, known as a pentagonal icositetrahedron, has an opening in each of its 24 faces from which "tendrils" emerge that are suggestive of plant growth or possibly appendages of an insect or sea creature.
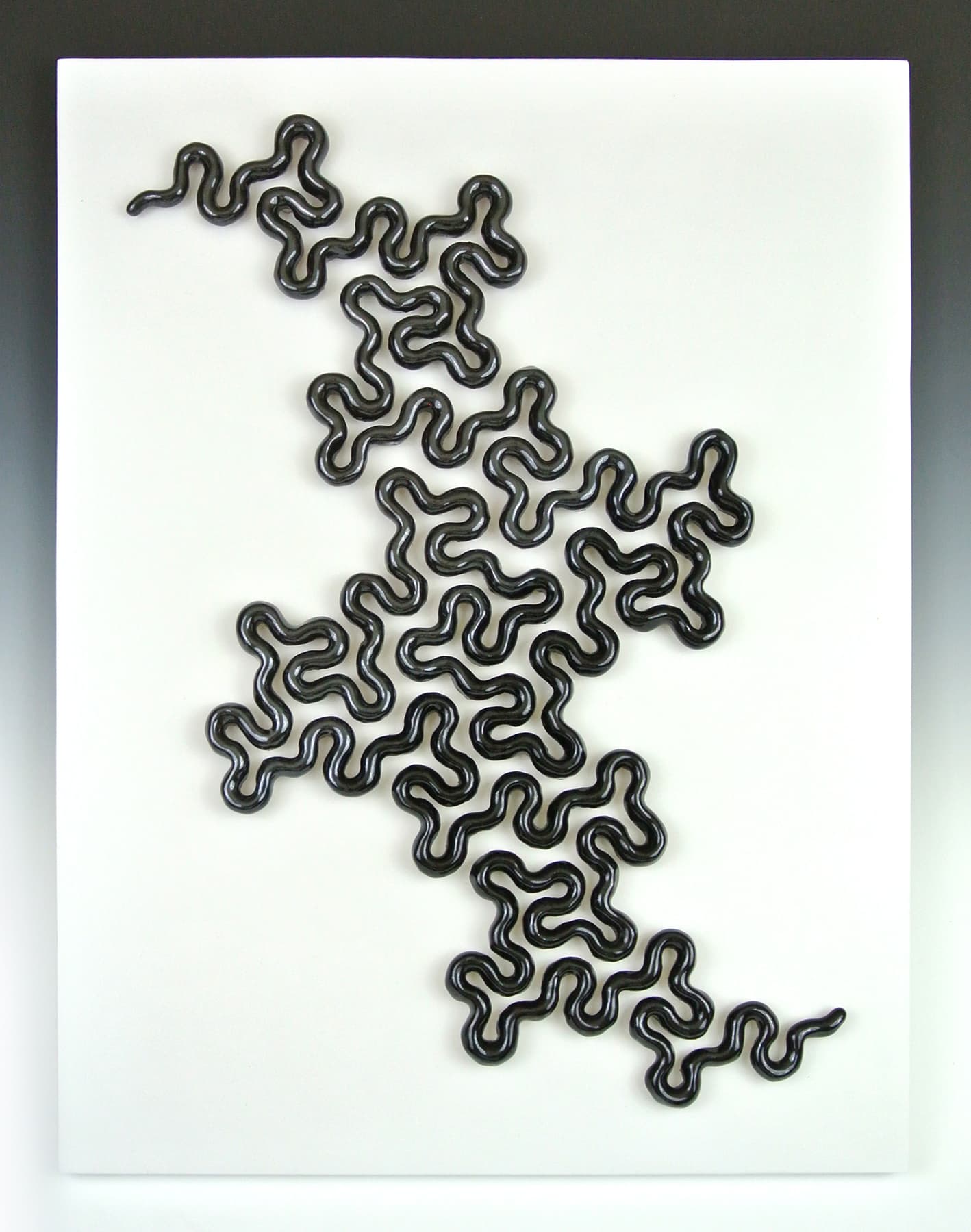
This sculpture is based on a particular stage in the development of a fractal curve known as the ternary dragon. This ceramic piece has been mounted on a board, with standoffs, partly to make it easier to handle without breaking. The resulting construct could be viewed as either a two-dimensional or three-dimensional artwork, which echoes the manner in which fractal curves can be considered as one-dimensional (a line), two-dimensional (a plane-filling object), or something in between.