Jean Constant

Statement

Mathematics and mathematical visualizations are meaningful at many scientific and technological levels. They are also an endless source of inspiration for artists. The following artworks are part of the 12-30 project – one mathematical image a day for one year, 12 mathematical visualization software, January 1st, 2015 – December 31, 2015. The 365 images portfolio is available at https://jcdigitaljournal.wordpress.com/ and a printed compilation of the work will be available in the coming months at Hermay.org

Artworks

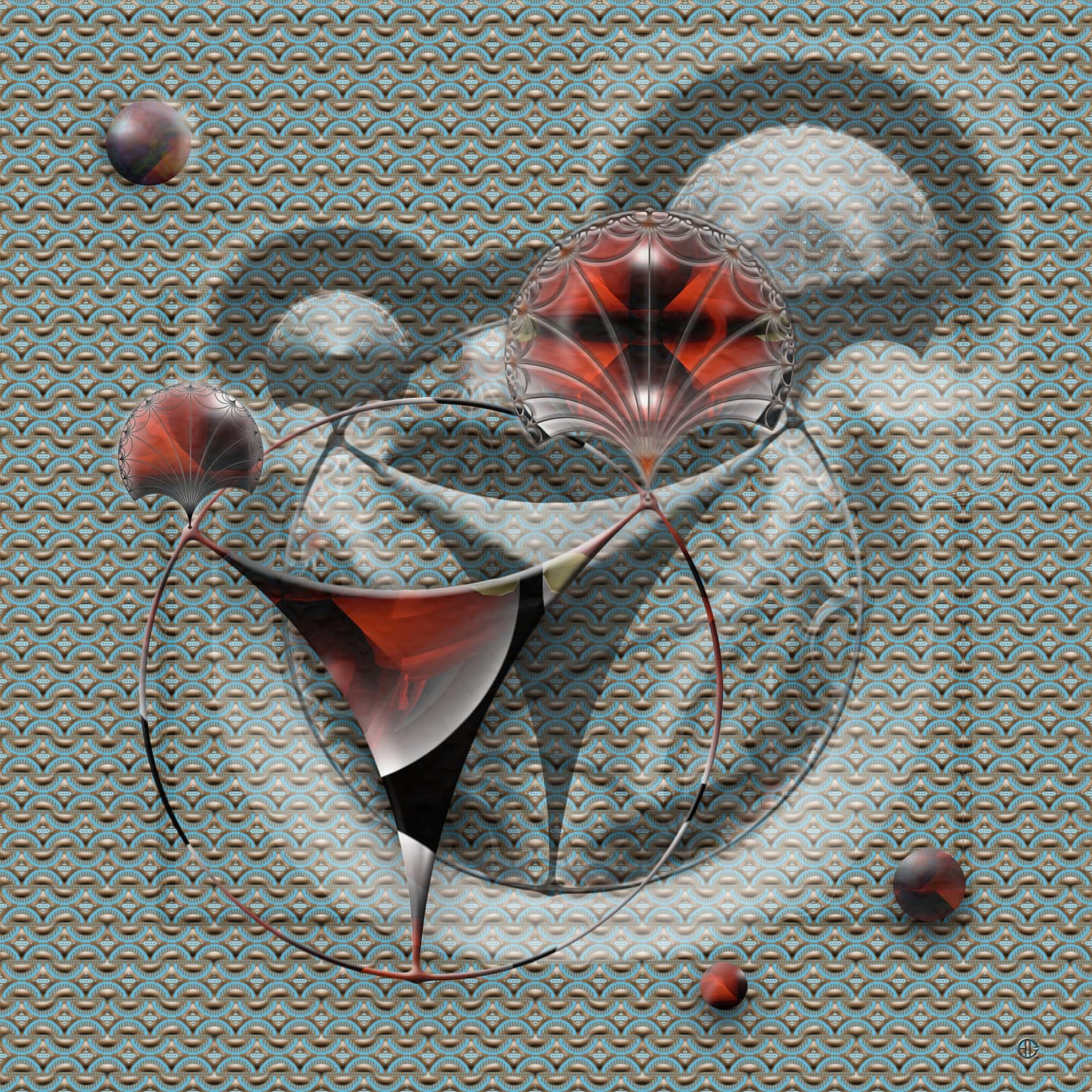
03 - Poincaré’s “Pas de deux”. The Poincare disk is a model for hyperbolic geometry in which a line is represented as an arc of a circle whose ends are perpendicular to the disk's boundary. From an original script by Eric W. Weisstein based on a program by Matthew Cook for Wolfram-Mathematica.

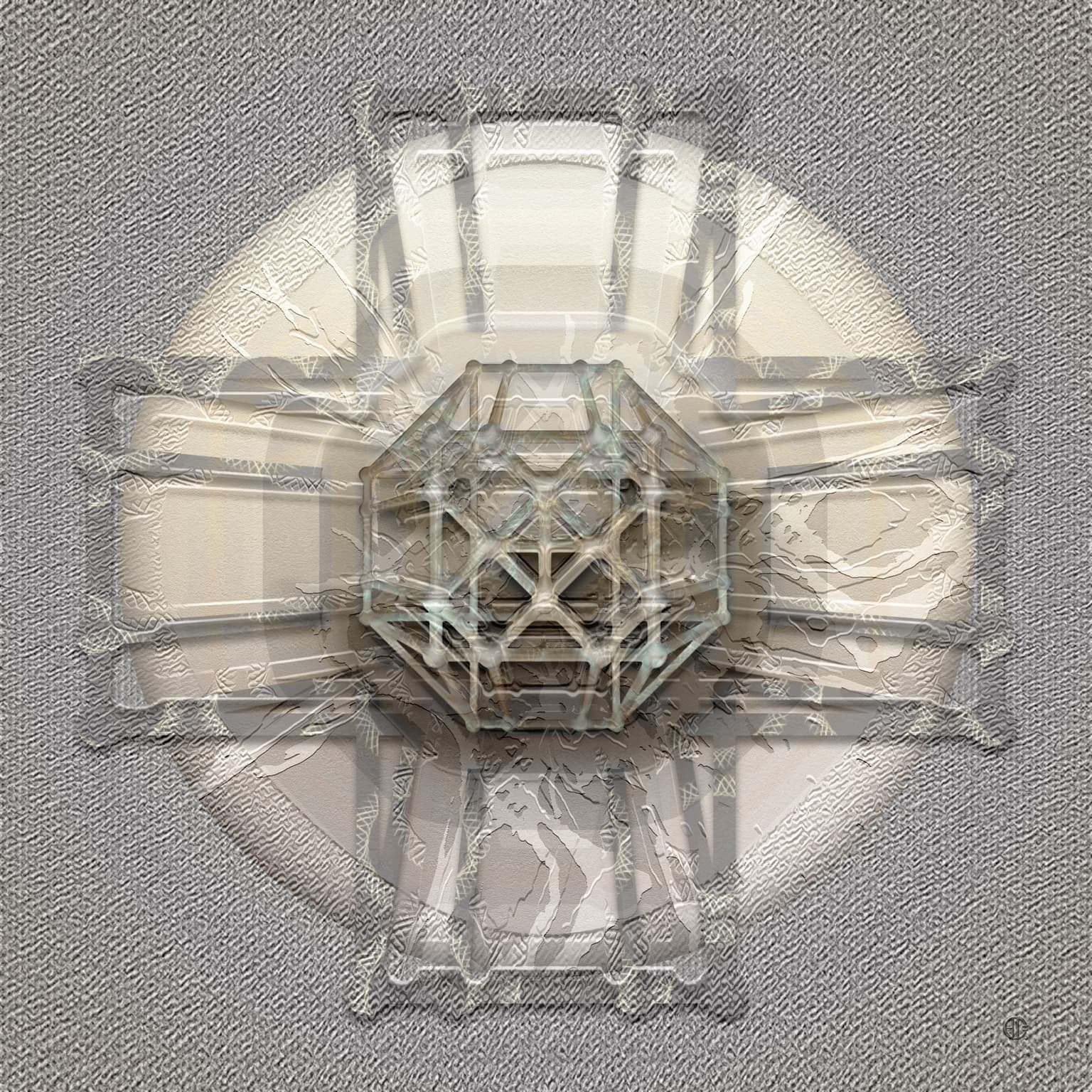
02 - Unfolding polytope. The bitruncated 5-cell is a 4-dimensional polytope. The vertices of the truncated 5-cell can also be constructed on a hyperplane in 5-space. From an original script by Jeff Weeks for his geometry and topology software.