2016 Joint Mathematics Meetings
Robert Orndorff
Artists
Robert Orndorff
Statement
My paper folding art is math plus paper. Folded paper is simply tangible math. These particular figures can be folded from most types of paper. No tools are needed. Each is folded from a simple uncut rectangle. Do you listen to music or perform it yourself? Likewise, I invite you to contemplate these figures or fold them yourself. For centuries, artists have used math, technology or special tools. My work is part of that tradition. I will give a talk at the meeting, and will share the crease patterns for these figures with anybody who is interested.
Artworks

OSU Triptych No. 2
20 x 46 cm
Paper and Acrylic
2015
This is a permanent manifestation of an ephemeral artwork, namely, one solution for a specific one-straight-cut problem. Such problems are usually stated as follows: How must one fold a paper rectangle into a flat figure such that one straight cut through all of the layers will produce a given planar straight-line graph? Here the problem has been solved with paper and then represented in acrylic. To a significant degree the work relies on transmitted and reflected light, and so it never looks the same twice. The figure (the letters "OSU") has been divided into three frames. The crease patterns for the left and right letters are pedestrian but that for the central letter is sublime.
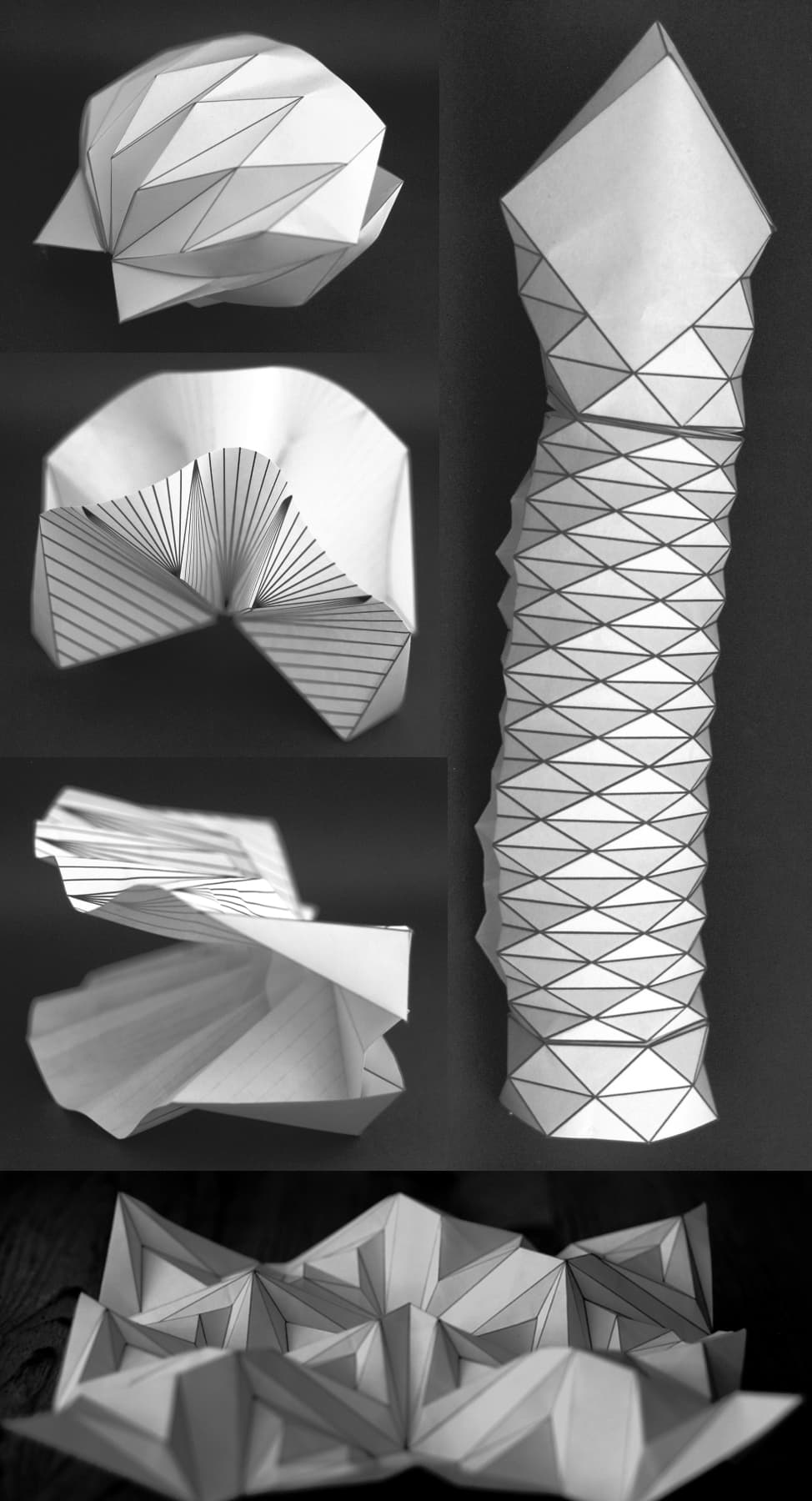
Five Examples from "Three & Twenty Bivalve Mollusks:" Cosmopolitan Scallop; Giant Clam Nos. 4 and 5; Hypar Seabed; and Geoduck
51 x 28 cm
Paper, Straight Pins and Cork
2012-15
Five small three dimensional figures from my book , mounted like butterflies with straight pins to a cork base in a two dimensional frame. These figures make use of both planar and curved faces. Some of the math behind such curved face figures was only presented for the first time last summer. The behavior at each of the crease pattern vertices is governed by a set of equations; some vertices permit the vertex to fold flat and others do not. Designing figures like these requires not only math but also trial and error, in a process that goes back and forth between the computer and the hands any number of times over days or weeks.