2016 Joint Mathematics Meetings
Frank A. Farris
Artists
Frank A. Farris
Professor of Mathematics and Computer Science
Santa Clara University
San Jose, CA
Statement
My artistic impulse is to let the beauty of the real world shine into the realm of mathematical patterns. My method combines photographs with complex-valued functions in the plane to create images with all possible types of symmetry: Euclidean, hyperbolic, and spherical symmetries (considered as actions on the plane). For some works, I then transfer plane images back to the sphere. All these methods are explained in detail in my book Creating Symmetry: The Artful Mathematics of Wallpaper Patterns, published in 2015 by Princeton University Press.
Artworks
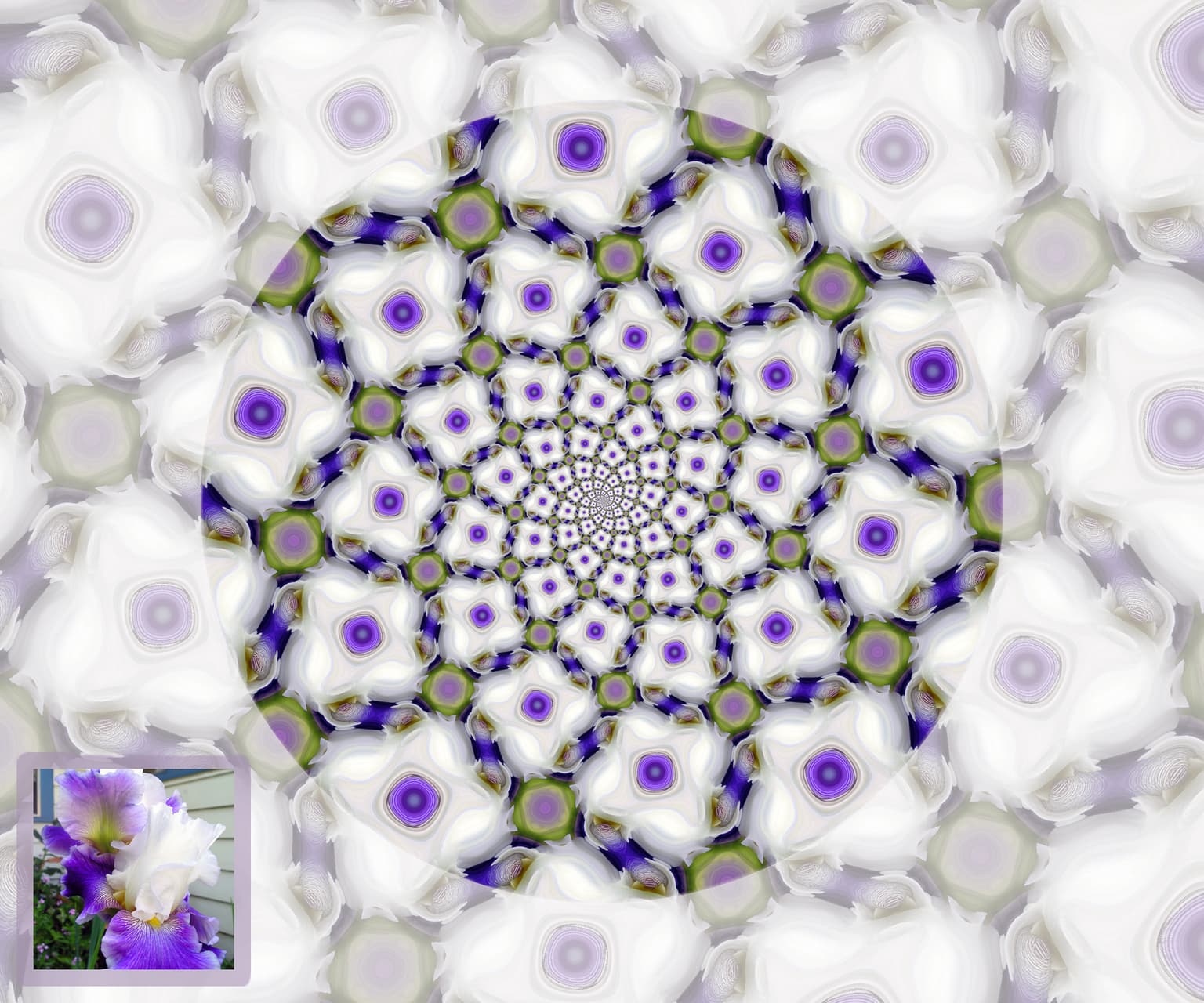
I photographed the irises and used complex wave functions to turn the image into a pattern with four-fold rotational symmetry. Then I applied a complex exponential mapping to wind the wallpaper around the complex plane, choosing just the right scaling to make the pattern match, while also creating five-fold symmetry. I bleached an outer ring to bring focus to the center of the spiral and to allow the original photograph of the iris to stand out. Details about wallpaper waves appear in my book, Creating Symmetry: The Artful Mathematics of Wallpaper Patterns.
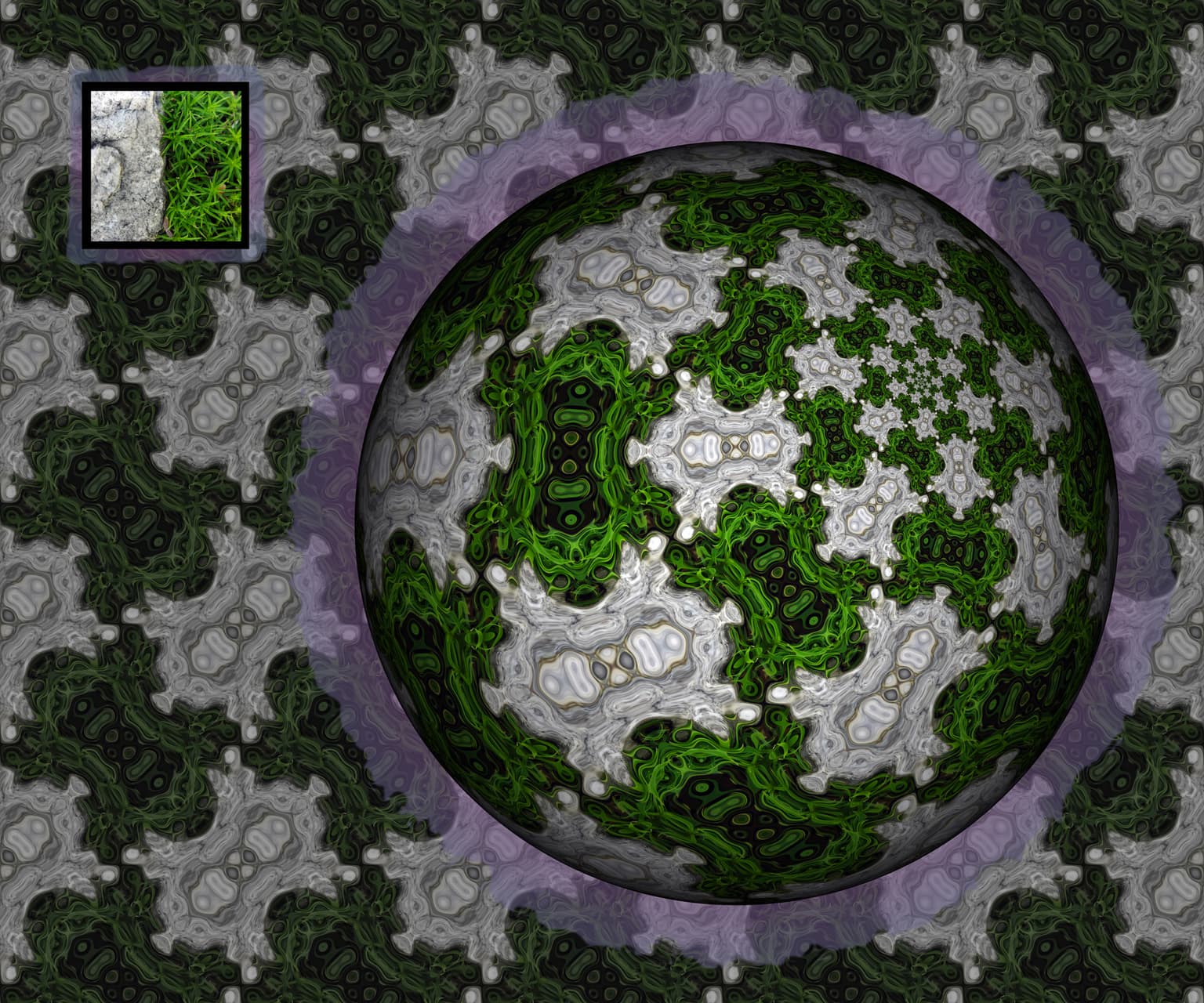
In my book, Creating Symmetry: The Artful Mathematics of Wallpaper Patterns, I explain how to construct complex wave functions to create color-reversing symmetry. These frogs and bugs are shaped by waves invariant under the wallpaper group cmm, with color group p4g. Rather than color the waves with a perfectly color-reversing source image, I used a photograph where the two halves are only loosely symmetric, creating approximate color symmetry. The chosen waves could produce a tiling with two congruent shapes, but the asymmetric photo produces two slightly different tiles. I wound the original wallpaper image around the sphere with a complex exponential map and stereographic projection and assembled the ingredients in a collage.