Felicia Tabing
Artists
Felicia Tabing
Assistant Professor (Teaching) of Mathematics
University of Southern California
Los Angeles, California, USA
Statement
I recently learned about Lomonaco and Kauffman’s knot mosaics, which inspired me to create a set of carved blocks so that I can create prints of projections of knots. As a reference to nautical knots and a physical knot, I carved an image of rope into the blocks. I carved the rope in negative on square blocks so in printing, the ink will inevitably overlap. In the process of printing, I went from printing knot mosaics with a minimal number of tiles to designing knot projections so that the image of the knot fills up a given space with only non-empty tiles. I also transitioned from printing in solid color to printing with a gradient of colors to exhibit the different rotations of the tile, which resulted in more interesting visual effects.
Artworks
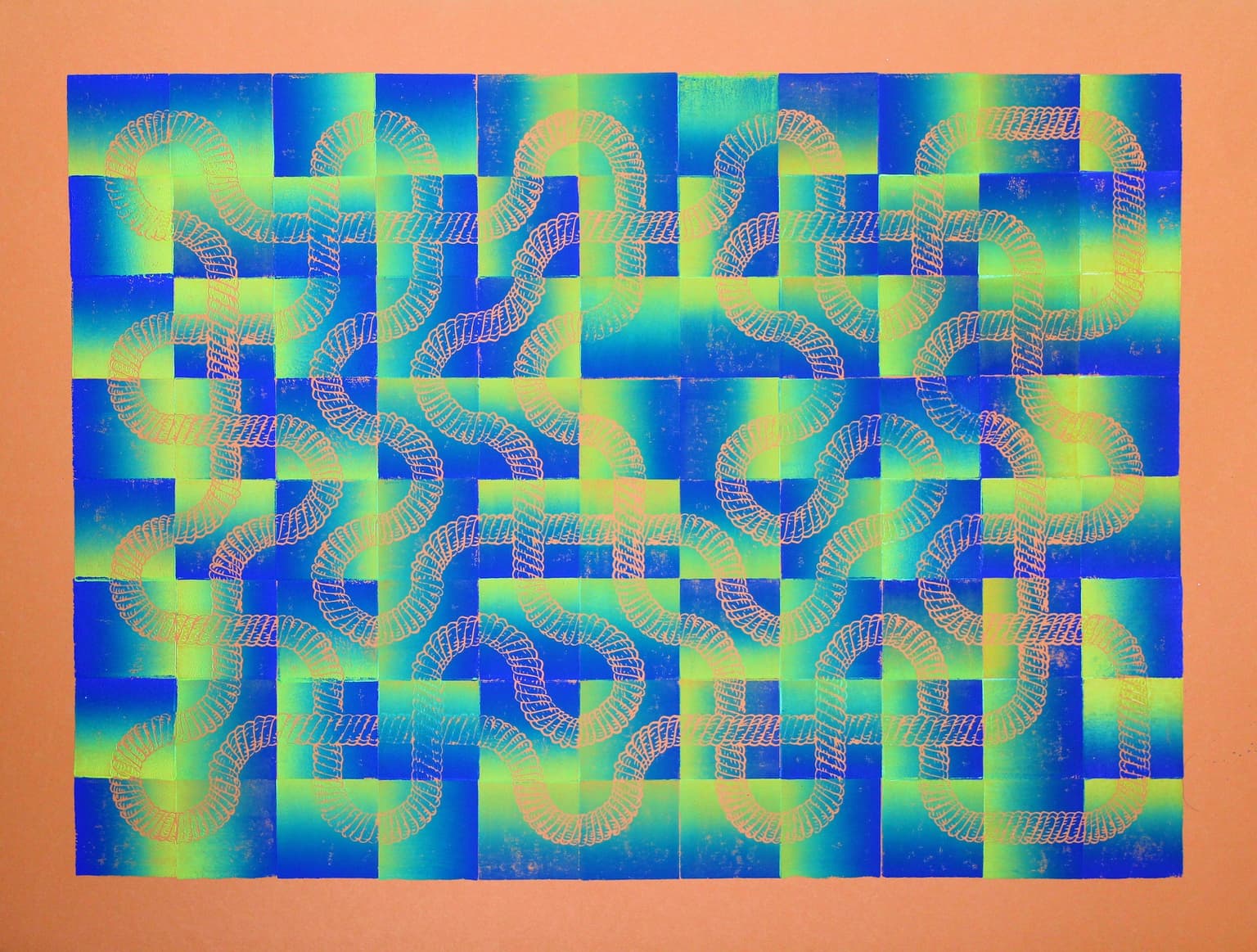
Seventeen Crossings
50 x 65 cm
Block Print, Ink and Paper
2017