Manuel Diaz Regueiro
Artists
Manuel Diaz Regueiro
Artist
Math Teacher, Retired
Lugo, Galiza, Spain
Statement
This year the theme of my two artworks are the projections or maps (we are in the International Year of Tourism). Mathematical projections are the most useful means of representing the surface of the Earth. And by using them in the geographical maps allows us to travel. But, here, artworks are made with reverse projections onto predefined surfaces. From a 2d plane I create a complete 3d artwork, so that its projection in the plane is the original map. Surfaces, in this case, are two examples of non-Euclidean geometry: the sphere and the pseudo-sphere.
Artworks
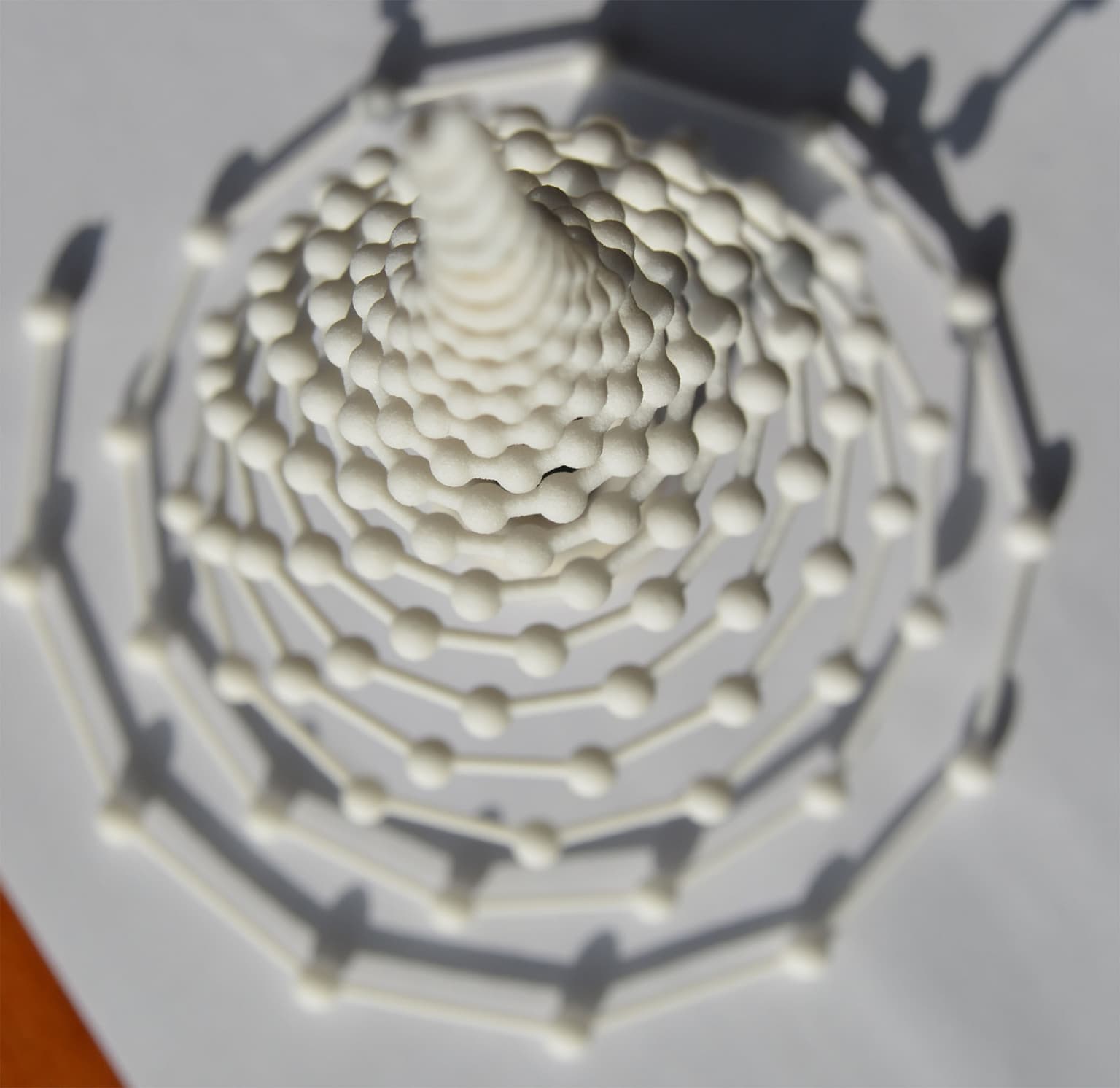
Fibonacci Spiral in a Lobachevski World
18 x 15 x 14 cm
Strong & Flexible Plastic
2017
We begin by creating a Fibonacci spiral in the plane we pass into space giving it a height, at each point, proportional to the inverse of its distance from the origin.
Thus we obtain a hyperboloid of revolution, which approximates a world of Lobachevsky, to a pseudo-sphere. But we do not do it with the whole surface of the hyperboloid, but in the curve that fits the key points of the Fibonacci spiral raised from the plane.

Bourgoin design in a Sphere
16 x 14 x 12 cm
Strong & Flexible Plastic White
2017
Following the design number 9 of the book "Arabic geometrical patterns & designs" I made a digital and vectorial 2d version of it. And then, I put it in a plane passing through the center of a sphere. The projection (up and down) on the sphere deforms the projected result in the vicinity of its equatorial zone. So, to preserve form and visibility, I made a partial projection of the mosaic. As in the exhibition "Unfinished" in the Metropolitan there are reasons to do so: an unfinished work of art allows to show the steps of creation and to imagine several possible and different continuations. The observer's imagination is what allows him to finish the work of art in his mind.