Nick Mendler
Artists
Nick Mendler
Undergraduate Student of Mathematics
University of San Francisco
San Francisco, California, USA
Statement
Generative art helps me explore recursion and express the mysterious nature of iterative systems recently made visible by the advent of computers. In particular, pieces here express the evolution of pairs of fractal trees through selected parameter spaces. Trees are defined by n transformations which specify the self-similarity of an n-branch tree to the n respective copies emanating from an initial trunk segment. Branches are generated by recursively transforming and appending segments in a chain beginning with the trunk, so that the whole tree may be defined as the union of all such chains. Artwork diverges from the expected appearance of such trees and abstractly views a continuum of parameters all at once through transparency overlay
Artworks
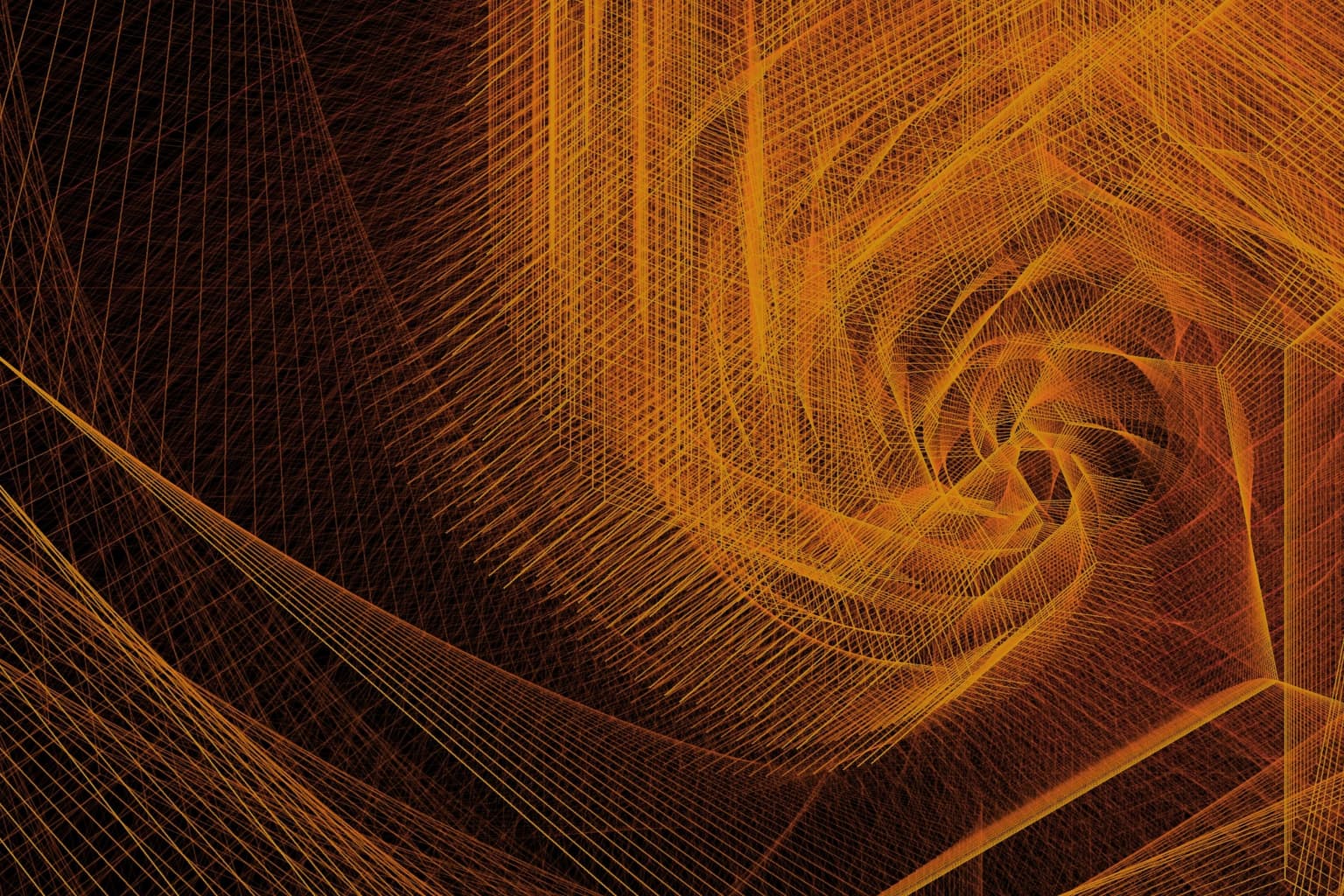
Parameter Wind
30 x 20 cm
Digital Print
2017

13's Flower
17 x 30 cm
Digital Print
2017