Regina Bittencourt

Statement

Our culture has two completely separate disciplines for mathematics and for art. I believe that mathematics has a unique intrinsic beauty. In my work I want to show the elegance of Mathematics: the beauty of its curves, the game of algorithms and how organized numbers may form algebraic surfaces. I am not a mathematician, so sometimes I have to make great efforts to understand what has caught my attention. But my deepest motivation is to dominate something that necessarily implies understanding before developing the images of what has captivated me. Mathematics are to be discovered. It exists since the Universe exists and is waiting to be discovered by someone. I want to discover its beauty.

Artworks

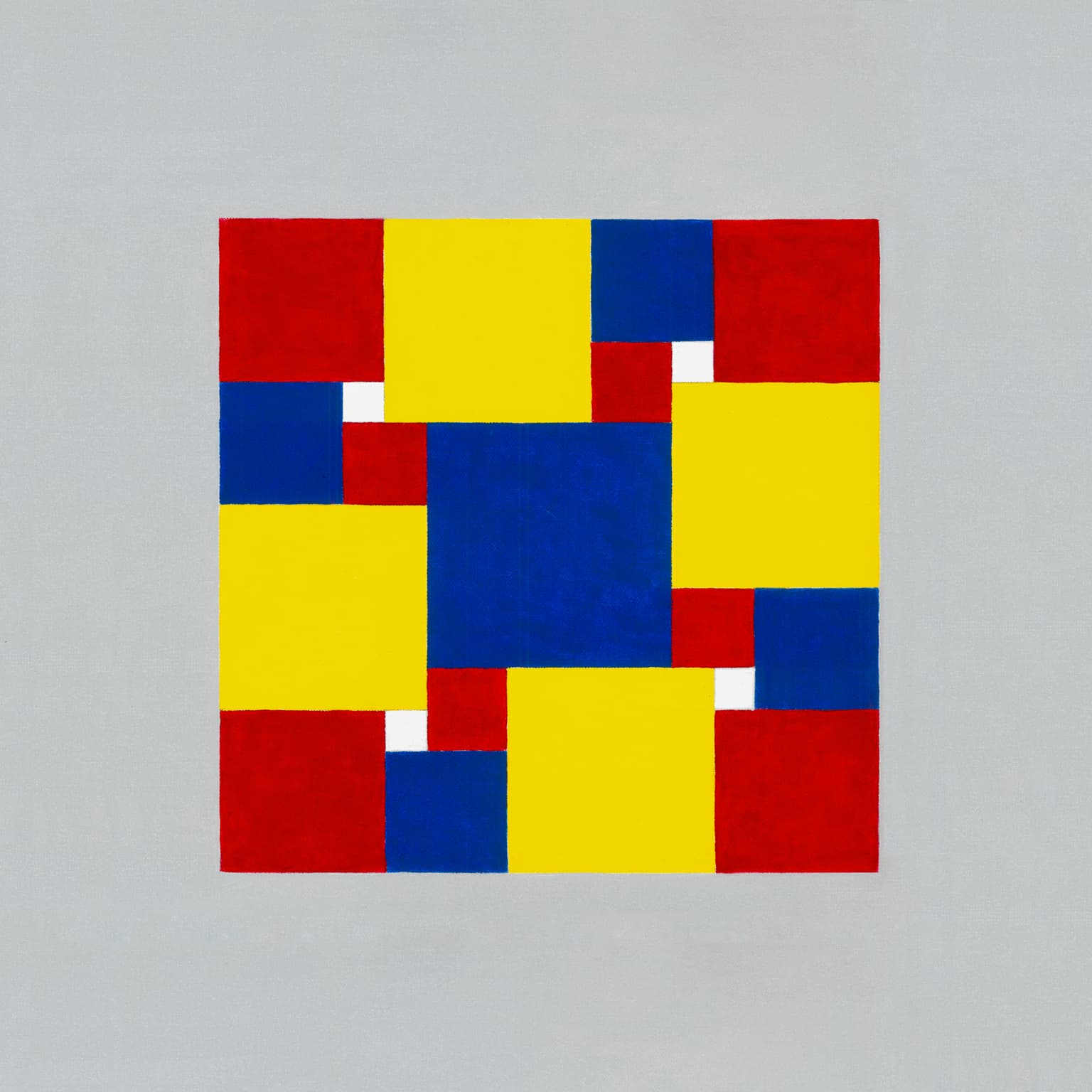
This artwork mixes three math problems: It is a Simple Imperfect Squared Square of order 17, colored using the Four-Color Map Theorem and design and painted with Symmetry. The 17 squares are not of different sizes which makes it imperfect; and simple because no subset of the squares forms a rectangle or a square. The Four-Color Map Theorem states that any map in a plane can be painted using four colors, so that regions sharing a common boundary do not share the same color. The main square has rotational Symmetry of order 4, since the tiling is invariant when rotated by 90 degrees.

An algebraic surface are the points in space that satisfy a polynomial equation and may be plotted in the Cartesian coordinate system x, y, z. I Love Chocolate! is a sextic algebraic surface, the one of the cube that makes the chocolate, along with another cubic surface forming the chocolate wrapper: (x^6+y^6+z^6-1) * -(x^3+y^3+z^3-0.5) = 0