2017 Joint Mathematics Meetings
Cynthia McGinnis
Artists
Cynthia McGinnis
Professor of Mathematics
University of Maryland Global Campus
Japan
Statement
Technology has allowed us to examine mathematics visually. Through visualizations and color, we can often discern patterns that we might not have noticed before. Using digital imagery, I strive to create visual mathematical concepts. Most of what I create I consider artist play with an underlying motivation towards the ideas and philosophies of mathematics.
Artworks
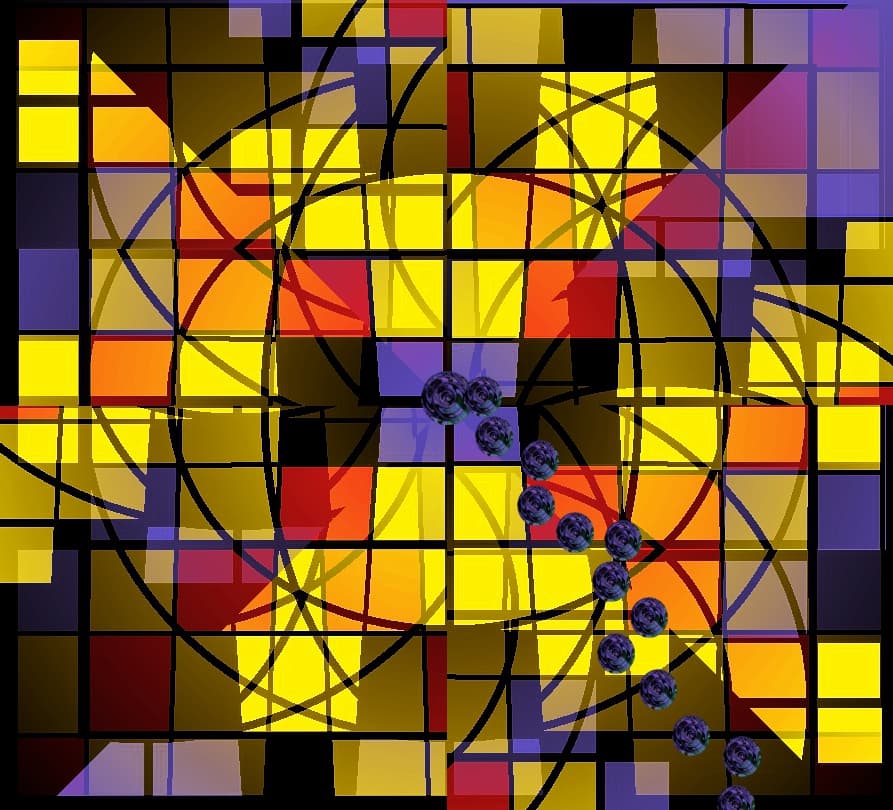
Fibonacci Numbers Mod 4
47 x 43 cm
Digital Art
2015
The digital graphic design is a matrix consisting of Fibonacci numbers. The matrix is reflected and rotated to create the underlying design. Shadows and circles are symbols of planetary nebula. Are the sphere's emerging from the center or are they being attracted by a black hole?

Cyna's Magic Square
50 x 50 cm
digital art
2015
In "Cyna's Magic Square", each square in the design is an integer matrix. Each integer in the matrix is randomly assigned a color. The squares are reflected vertically and horizontally to create a larger square. Two squares are placed side by side to create a golden rectangle. The golden rectangle is then rotated to give the viewer a feeling of a 3 dimensional object.