Robert Bosch
Artists
Robert Bosch
Artist/Professor of Mathematics
Oberlin College
Oberlin, Ohio, USA
Statement
The mathematician in me is fascinated by the various roles that constraints play in optimization problems: sometimes they make them much harder to solve; other times, much easier. And the artist in me is fascinated by the roles that constraints play in art. All artists deal with constraints, and many choose to impose constraints upon themselves. The benefit of this was well expressed by Joseph Heller (paraphrasing T.S. Eliot): "If one is forced to write within a certain framework, the imagination is taxed to its utmost and will produce its richest ideas."
Artworks
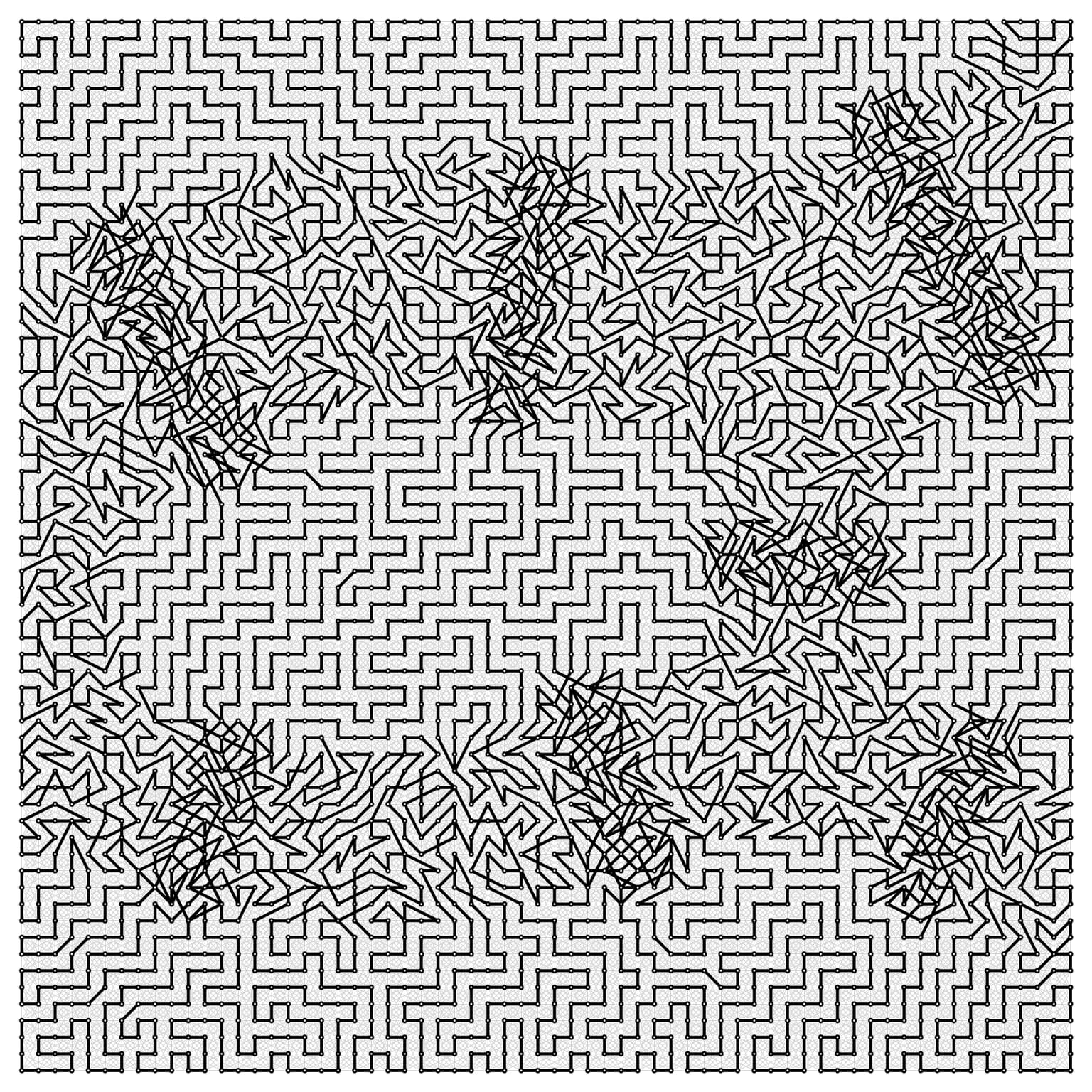
A new solution to Euler's Königsberg Bridge Problem
25 x 25 cm
Digital print on aluminum.
2018
From a distance, the viewer sees a part of the city of Königsberg: a river separating the city into four land masses (one at the top, one at the bottom, and two islands) as well as seven bridges that connect pairs of land masses. In 1736 Leonard Euler showed that it is impossible to take a walk that starts and ends at the very same place and crosses each bridge exactly once. Such a walk exists if and only if each land mass touches an even number of bridges (has even degree). Here, none of them do. From up close, the viewer sees a spanning-Eulerian-subgraph rendition, each vertex having degree two or four. In the subgraph, no matter where you start, there is a walk that uses each edge of the subgraph once and returns to where you started.