Kento Nakamura
I am interested in visualization of fractals, especially Kleinian groups. Kleinian group theory is one of the fields of mathematics studying Möbius transformations. Visualized Kleinian groups frequently have a complicated and beautiful fractal structure, and they feast our eyes on the wonders.
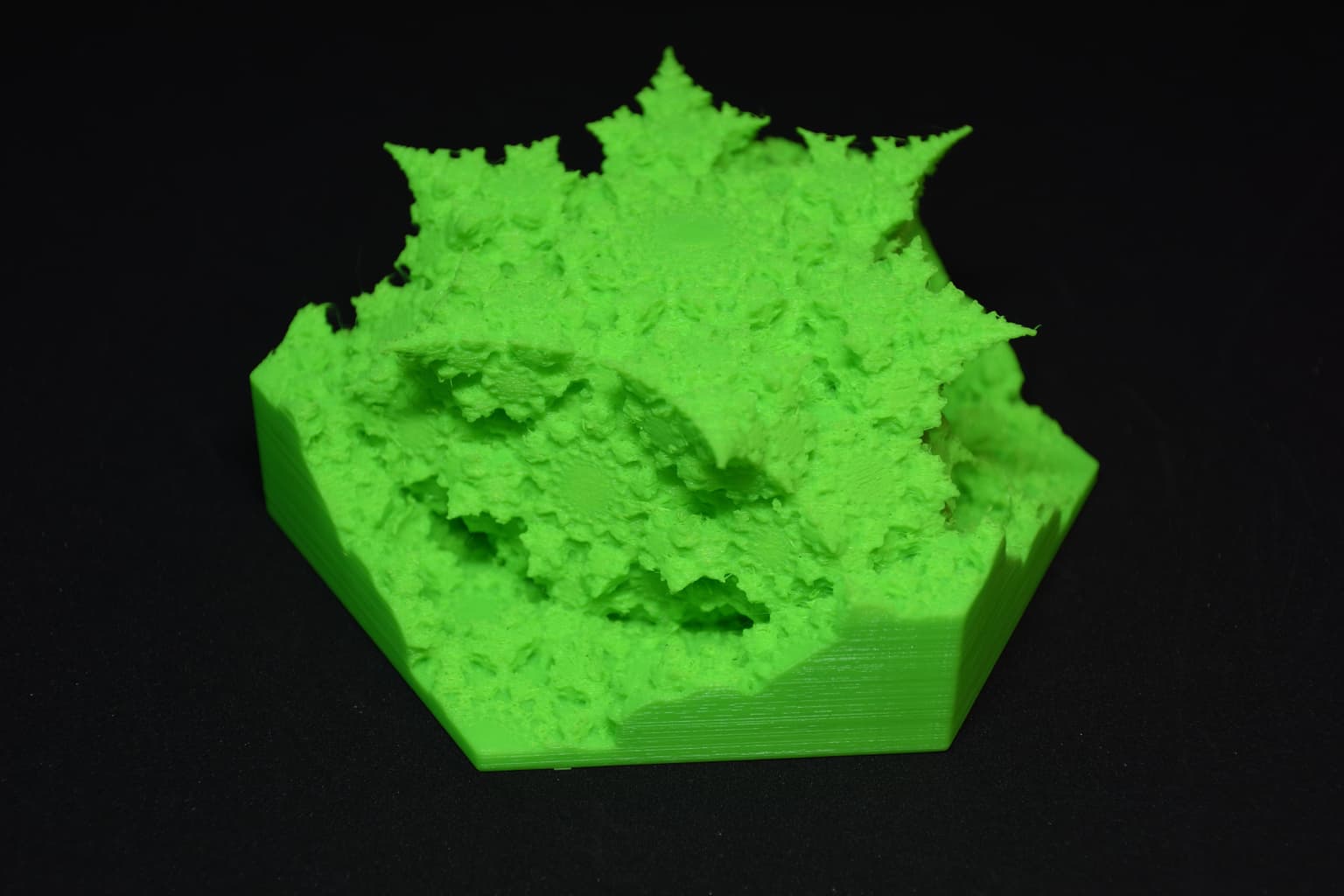
They are 3D-printed fractal objects called quasi-fuchsian fractals or quasi-spheres. They are made of tiling of a sphairahedra, which are analogous to polyhedra but with spherical faces. They are one of the early examples of the 3D fractal. For more details of mathematics, read the web page about sphairahedra and quasi-spheres at https://sphairahedron.net.
Recently, I'm working on visualizing these fractals by computer. I succeeded in rendering many fractal images that have never visualized. I also developed tools to generate the fractal data suitable for 3d-printing and printed them. By observing printed objects, you may be able to find the structure which you can not understand only by computer graphics images.
