2018 Joint Mathematics Meetings
Robert Bosch
Artists
Robert Bosch
Artist/Professor of Mathematics
Oberlin College
Oberlin, Ohio, USA
Statement
The mathematician in me is fascinated by the various roles that constraints play in optimization problems: sometimes they make them much harder to solve; other times, much easier. And the artist in me is fascinated by the roles that constraints play in art. All artists deal with constraints, and many choose to impose constraints upon themselves. The benefit of this was well expressed by Joseph Heller (paraphrasing T.S.~Eliot): "If one is forced to write within a certain framework, the imagination is taxed to its utmost and will produce its richest ideas."
Artworks
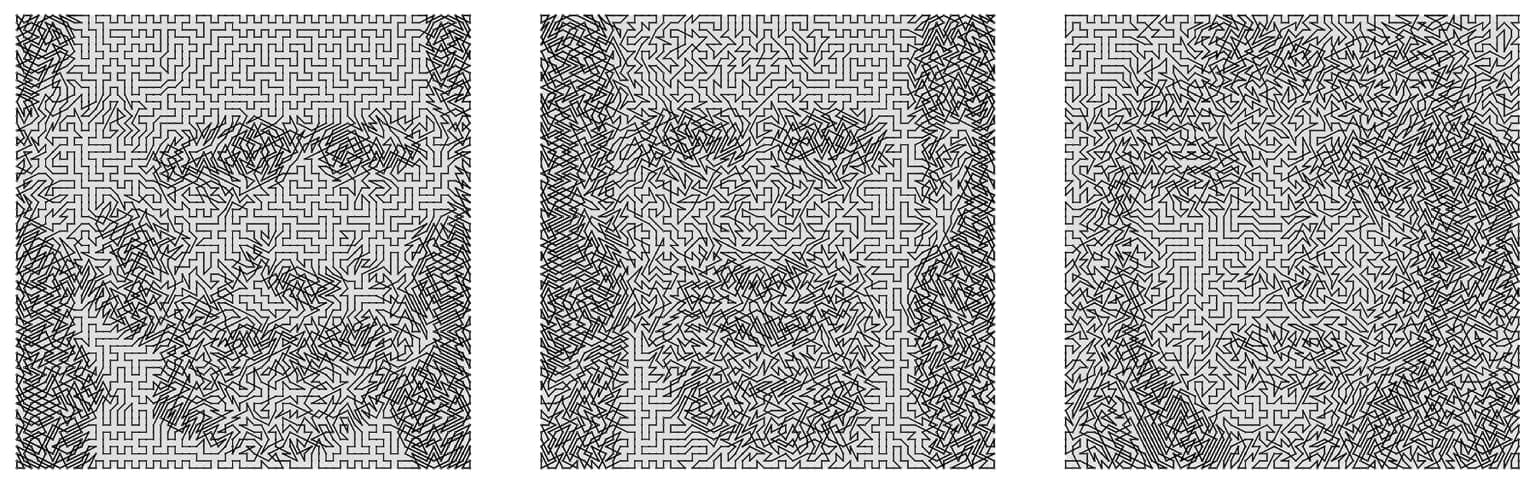
Three Hamilton Cycles of the Graph KiKn64x64
9 x 28 cm
Digital print on canvas
2017
A triptych of Hamiltonian-cycle portraits of William Rowan Hamilton, Lin-Manuel Miranda, and Linda Hamilton. Each has 4096 vertices. Each is a Hamiltonian cycle of the same graph, KiKn64x64. The vertex set is the squares of a 64x64 chessboard. The edge set are the moves that can be taken by a chess king or knight. Each Hamiltonian cycle was obtained by integer-programming-based local search, using Gurobi to solve the integer programs.

Three Hamilton Cycles of the Complete Graph on 4096 Vertices
9 x 28 cm
Digital print on paper.
2017
A triptych of Hamiltonian-cycle portraits of William Rowan Hamilton, Lin-Manuel Miranda, and Linda Hamilton. Each has 4096 vertices. Each was produced by solving a 4096-city TSP. The optimal tours were found using the Concorde TSP Solver.