2018 Joint Mathematics Meetings
Gregg Helt
Artists
Statement
I find beauty in the way that relatively simple mathematical equations can give rise to complexity, and I render this complexity as images. My artistic process is usually to study a particular technique in generative art, then experiment with enhancing or extending said technique in new ways to expand the artistic and mathematical toolkit. My art pieces are often demonstrations of those experiments.
Artworks
Elliptical Box
50 x 50 cm
Digital Print on Aluminum
2017
This piece is another result of my experiments with modifying the 2D Mandelbox by applying shape inversion as a substitute for the standard Mandelbox circle inversion. In this case I replaced circle inversion with shape inversion in an ellipse. Coloration is again determined based on the distance traveled by each point during iteration.
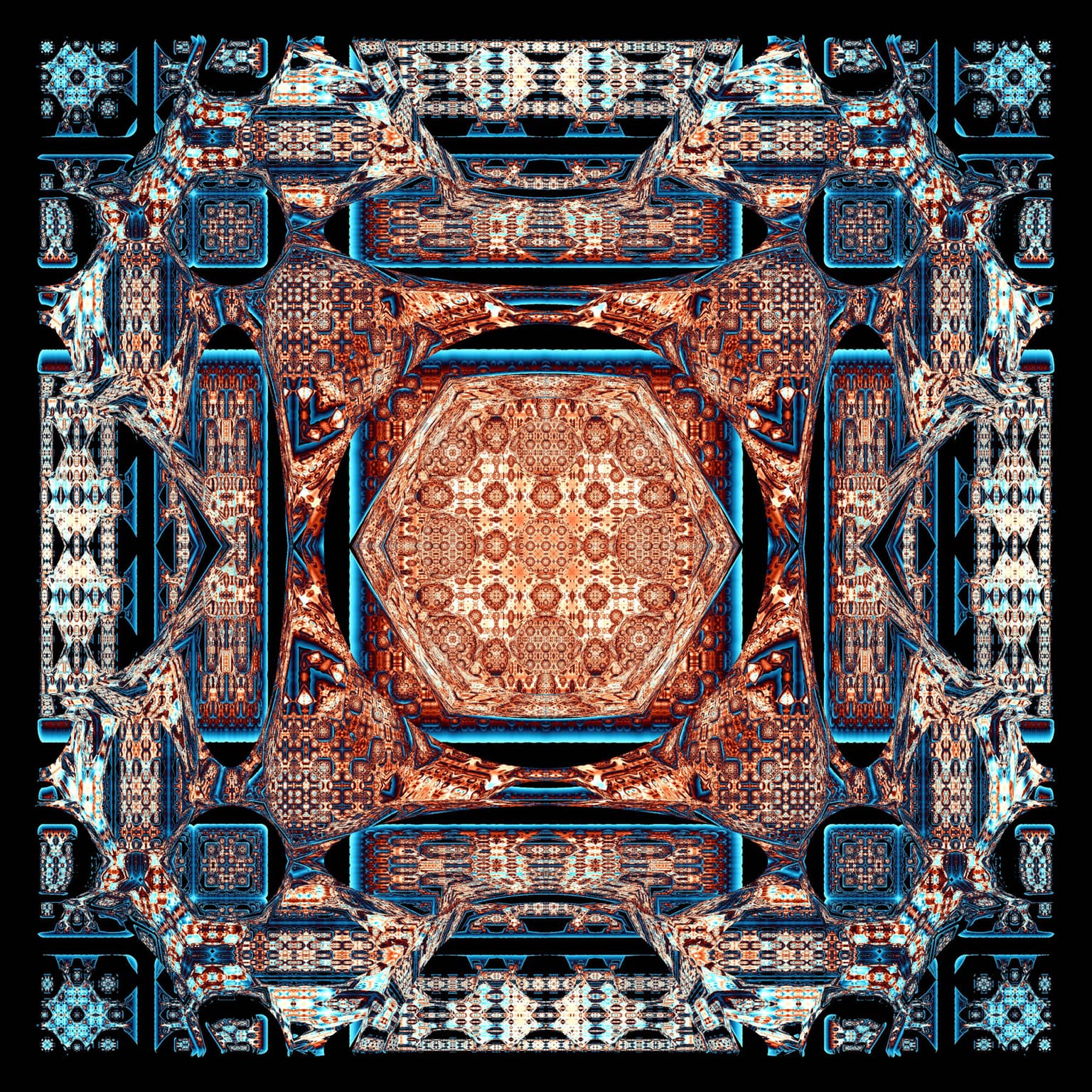
Hex Inverter
50 x 50 cm
Digital Print on Aluminum
2017
The Mandelbox is a class of escape-time fractals first discovered in 2010 that use a conditional combination of reflection, spherical inversion, scaling, and translation to transform a point under iteration. Although most artistic explorations of the Mandelbox have focused on 3D versions, it can be generated in any number of dimensions. I have recently experimented with modifying the 2D Mandelbox by applying shape inversion, or pseudoinversion, as a substitute for circle inversion (2D spherical inversion). In this piece I have replaced circle inversion with shape inversion in a regular hexagon. Coloration is determined based on the distance traveled by each point during iteration.