2018 Joint Mathematics Meetings
Nick Mendler
Artists
Nick Mendler
Undergraduate Student of Mathematics
University of San Francisco
San Francisco, California, USA
Statement
These images are linearly self-similar fractal sets generated by iterated function systems with linear affine transformations. I find the self similarity of natural scenes very fascinating, and my aim is to leave the viewer aware of these self similarities; yet also paying most attention to a fractal landscape or object reminiscent of something from the forest floor.
Artworks
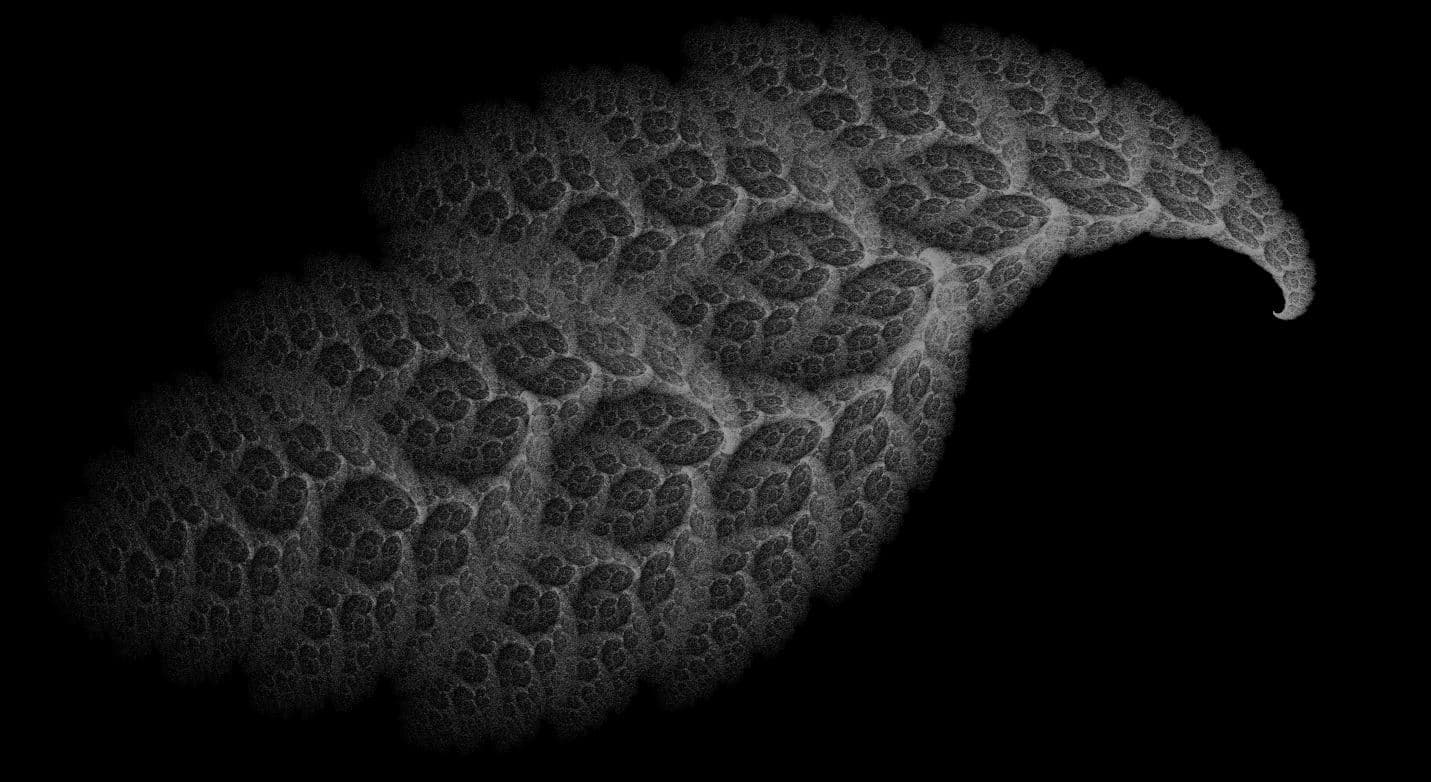
Elytrum
30 x 17 cm
Inkjet print
2017
This image reminds me of a leaf or an insects wing, which it is named for. This image is amazingly generated with only 2 affine mappings, and is thus only roughly 52 bits of information! I've spent a long time exploring the fractals generated by two affine maps and have never found one with a pattern quite so smooth and satisfying, I can't quite explain this long standing favorite.
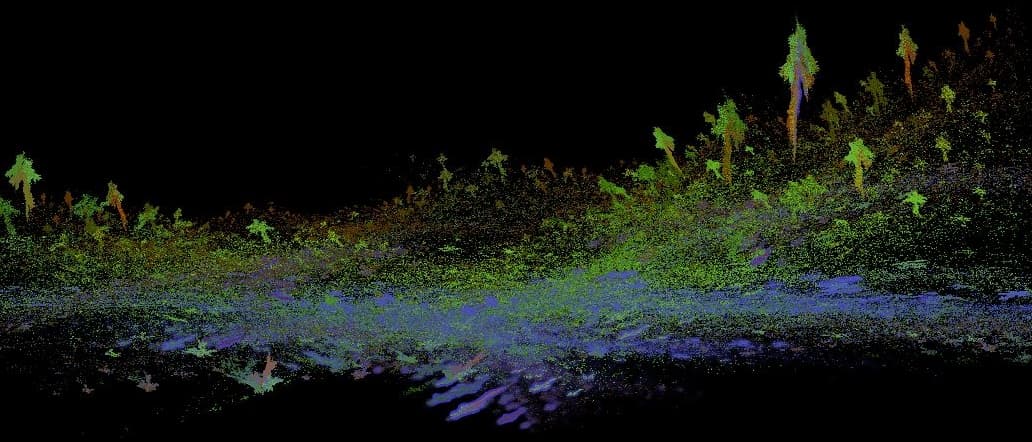
Valley
9 x 22 cm
Inkjet Print
2017
The landscape visible is in fact the attractor of a discrete dynamical system of 9 contractive linear affine mappings. I see hillsides covered in trees, and rivers running off into a bay with schools of fish. It's very intriguing that this complexity is captured by so little information; the 9 maps together with their associated colors make up 81 parameters such that our scene is a unique point in an 81-dimensional space, with 8-bit resolution we have 648 bits, not even a k of storage! What's I find most important about approximations of natural scenes is their ability to make us recognize that iteration is at the heart of what we observe, and notice that data from patterned events in reality may be extrapolated into their causes.