Douglas Burkholder
Artists
Douglas Burkholder
Chair and Professor of Mathematics
Lenoir Rhyne University
Hickory, North Carolina, USA
Statement
Long-long ago in a place far-far away, my love of art was placed on the back burner as science and mathematics consumed my life. The love of geometry and the desire for visualization for the sake of mathematical understanding has always been central to my teaching and comprehension. However, only decades later, have I begun to explore the artist within and attempted to merge art with my mathematics. My current math and art seeks to find patterns and beauty within non-periodic tiling of the plane. Most recently, I have been exploring hidden patterns within Penrose Tilings.
Artworks
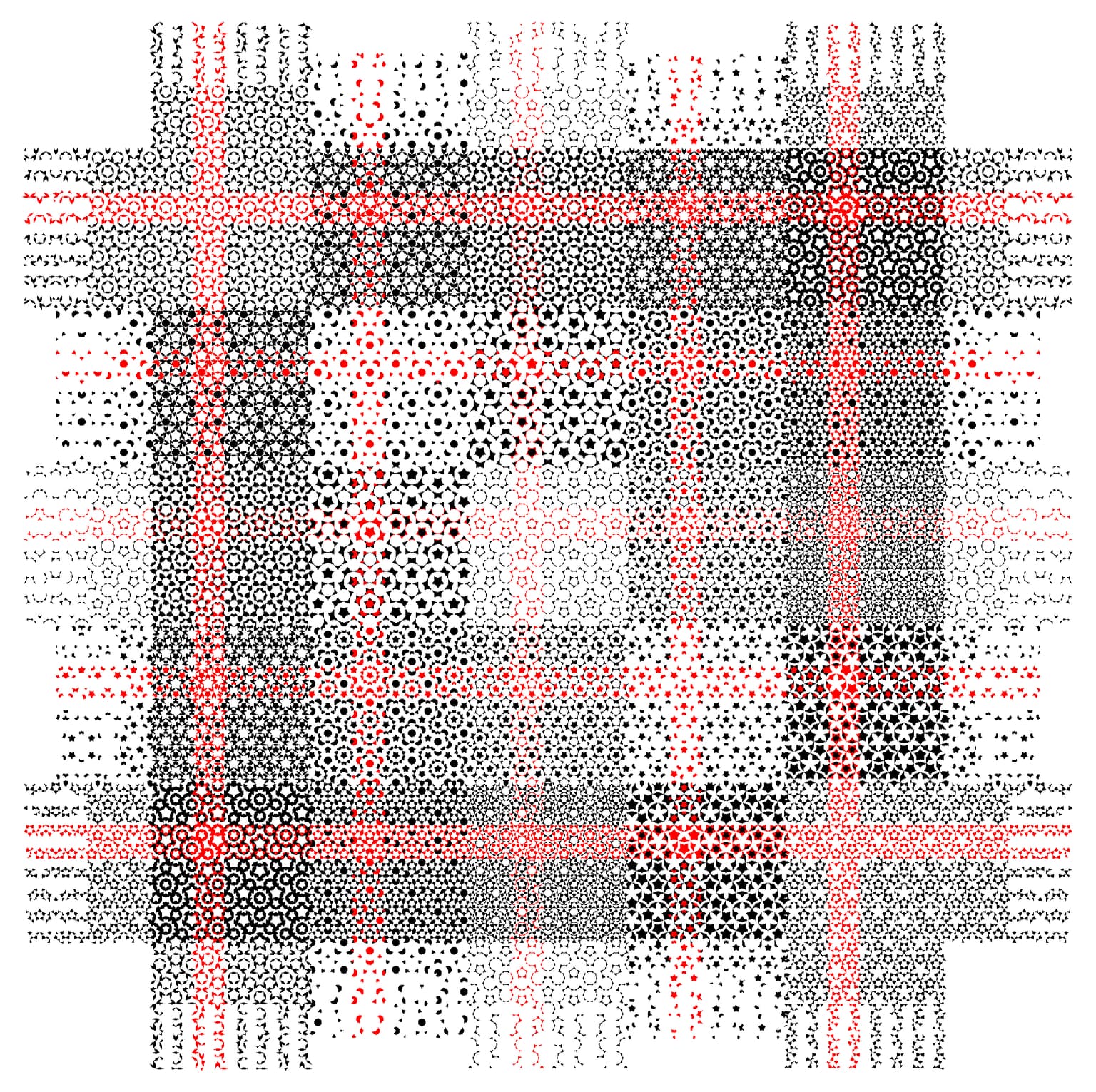
A Penrose Quilt
50 x 50 cm
Digital Print
2018
Starting from a Penrose Tiling of the plane with kites and darts, half darts and half kites were repeatedly subdivided into five smaller components until we had about 800,000 tiles within the desired square region. Each of these tiles were labeled A-E according to their relative location within the subdivision process. Next, the square is divided into five rows and five columns and, using the idea of Paint-by-Number, all the A tiles were painted in the first row, B tiles were painted in the second row, etc. The columns were similarly painted. This reveals 15 unexpected and distinctive patterns hidden within Penrose tiling. For example, the third column of the second row is the pattern resulting from painting all of the B and C tiles.