Arnaud Chéritat
Artists
Arnaud Chéritat
Researcher in Mathematics
CNRS/Institut de Mathématiques de Toulouse
Toulouse, FRANCE
Statement
My primary field of research is holomorphic dynamics, especially the study of rotation domains (Siegel disks). Lately, I got interested in geometry and topology. I have been doing a lot of programming for producing images related to my research in my early career, often trying to make them both aesthetic and informative, while avoiding clutter. More recently if have been focusing a lot on popularization actions around mathematics.
Artworks

A sphere eversion
20 x 12 x 12 cm
SLS 3d-printed nylon powder, acrylic paint, varnish, neodynium magnets
2015
After I found a new way to turn the sphere inside out (a.k.a. sphere eversion) and made a computer generated movie, I decided to have it 3D-printed. The whole collection contains 11 models representing stills of the movie. Since the eversion was desinged in a tomographic way, it was natural to slice the models so that one could see part of the tomography, and also look at the inside features. I used Blender to slice the model and add the the magnet holding protrusions, before ordering the 3D prints of the various pieces.
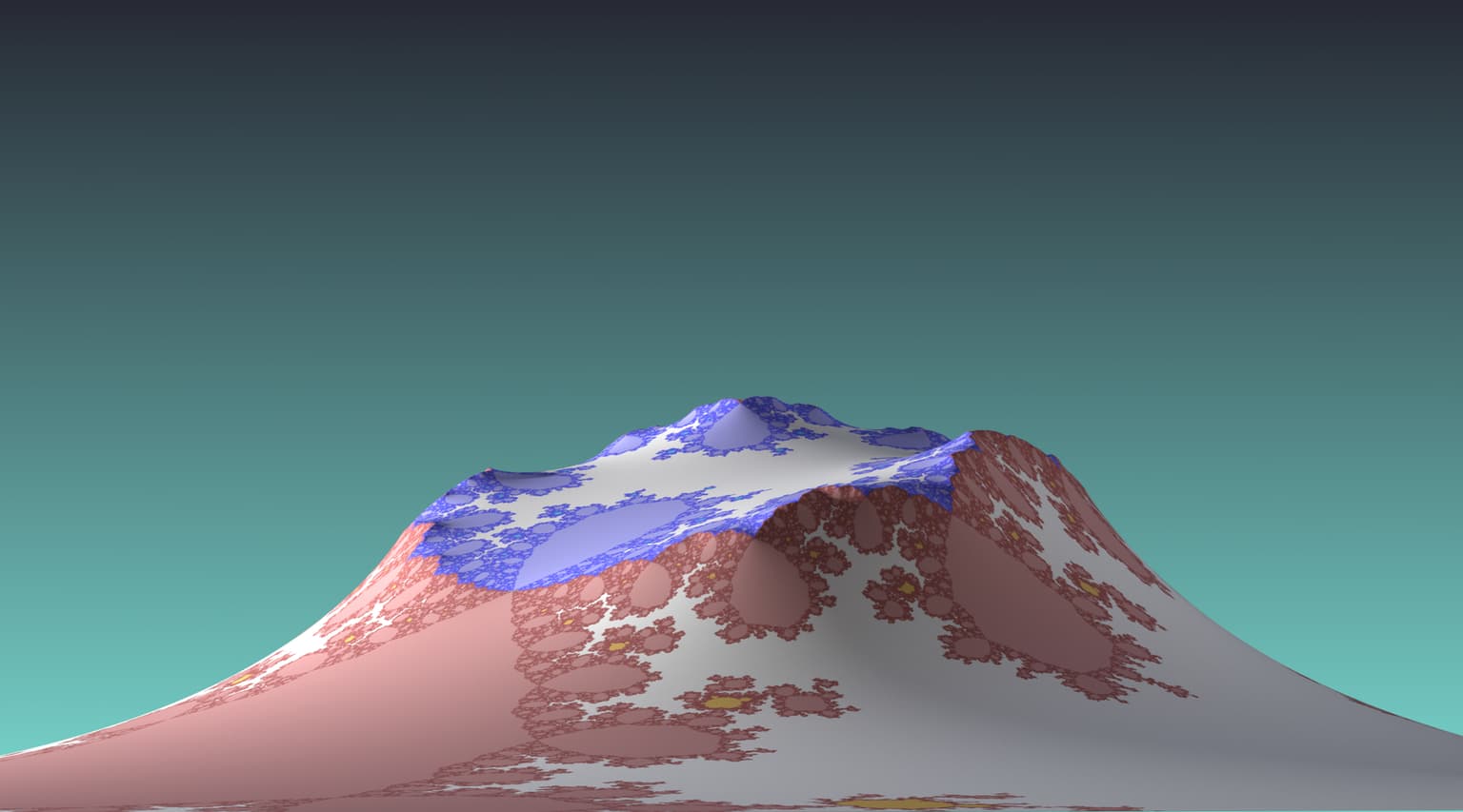
An exploration: mapping the size of rotation domains
Computer generated image
2019
Computer rendering of the graph of a function with a bifurcation diagram mapped over it. The height-coordinate z in the graph is related to the radius of convergence of the linearizing power series of a fixed point of a family of cubic polynomial parameterized by a complex number c=x+iy, as a function of x and y. This family is chosen to have a neutral fixed point with constant rotation number equal to the golden mean. The texture image corresponds the bifurcation diagram of this family. The result is reminiscent of an eroded volcano. Which critical point is on the boundary of the linearization domain depends on whether the parameter is within the caldera or not.