Dan Bach

Statement

I'm a former college math teacher from California. After using Mathematica for 25 years in the classroom, teacher workshops, and conference talks, I am now a 3D math artist and interactive book author. My students used to create mathematical graphics, movies, and sounds; either as class assignments or purely for pleasure. The present work involves Fourier series, trigonometry, and visual effects.

Artworks

Based on the Fourier Approximation of a square wave by trig functions, the x and y components are multiplied together to achieve a 3D function whose z-value spends most of its time near 1 or -1. Some fake water is added, and the whole thing has been zoom-blurred for your viewing pleasure. Can you tell how many sine terms were used? (click the More Info link to see this model in 3D!)

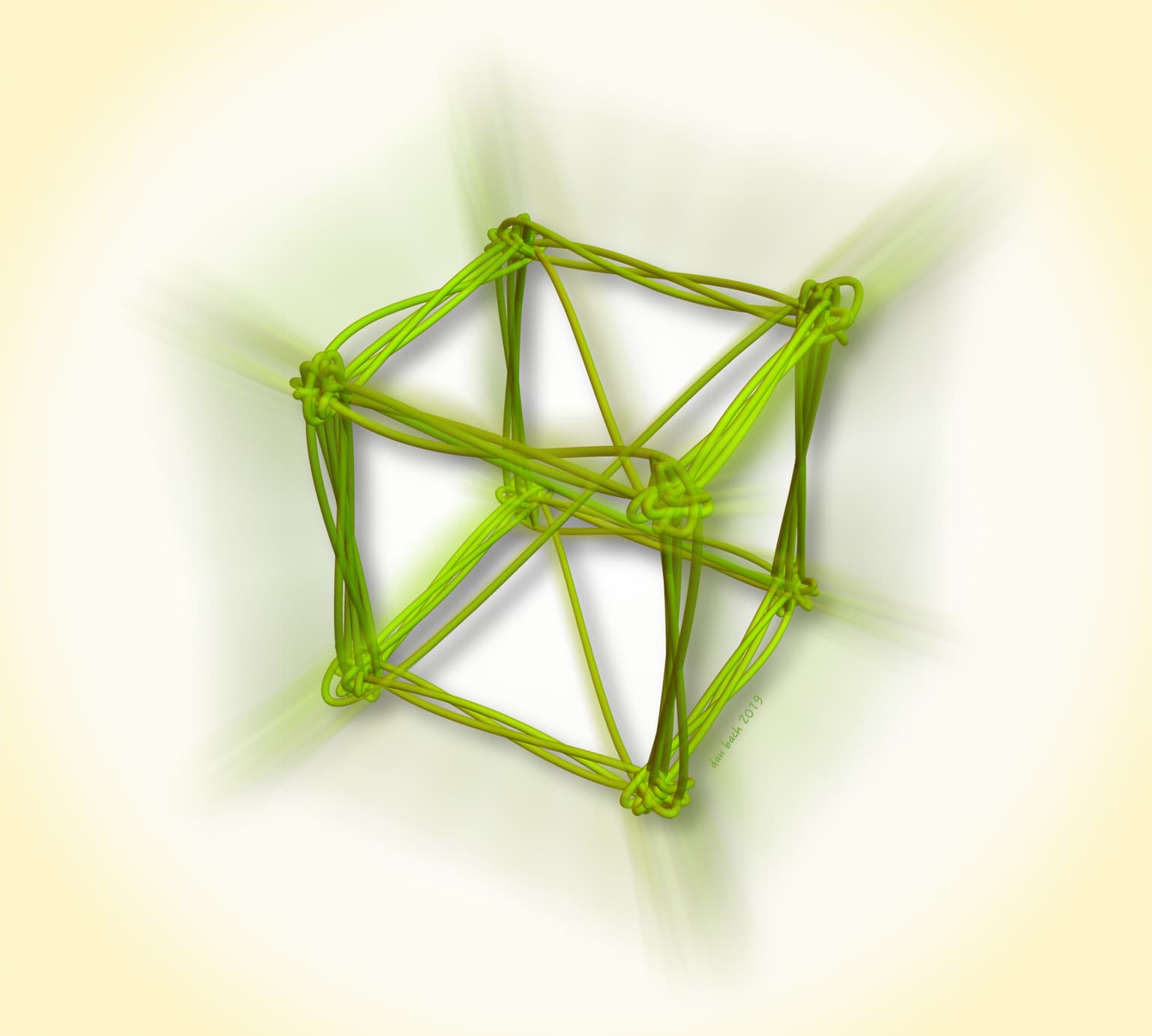
This is a rough trig estimate of a regular cube. Each coordinate (x, y, z) is given by different Fourier square wave approximations. The functions have different periods, and the three coordinates mostly hang out near 1 or -1. This is a continuous path so the curve can't trace out just the cube edges. Why is that? (click More Info to view the sloppy cube on the beach!)