Doug Dunham
Artists
Doug Dunham
Professor Emeritus of Computer Science
Dept. of Computer Science, University of Minnesota Duluth
Duluth, Minnesota, USA
Statement
I was originally inspired to make hyperbolic patterns by M.C. Escher's four "Circle Limit" prints. After succeeding in duplicating Escher's hyperbolic prints using computer methods, I noticed that such patterns came in "families" that were indexed by the multiplicities p, and q of the rotation centers. Such patterns are based on the regular tessellations {p,q} of regular p-gons meeting q at each vertex. This observation led to the creation of new hyperbolic Escher-inspired 2-dimensional patterns based on his Euclidean and even spherical repeating patterns. Some of Escher's patterns contain sublteties in their crystallographic classification, such as his Regular Division Drawing number 42 which appear to have symmetry group p4.
Artworks
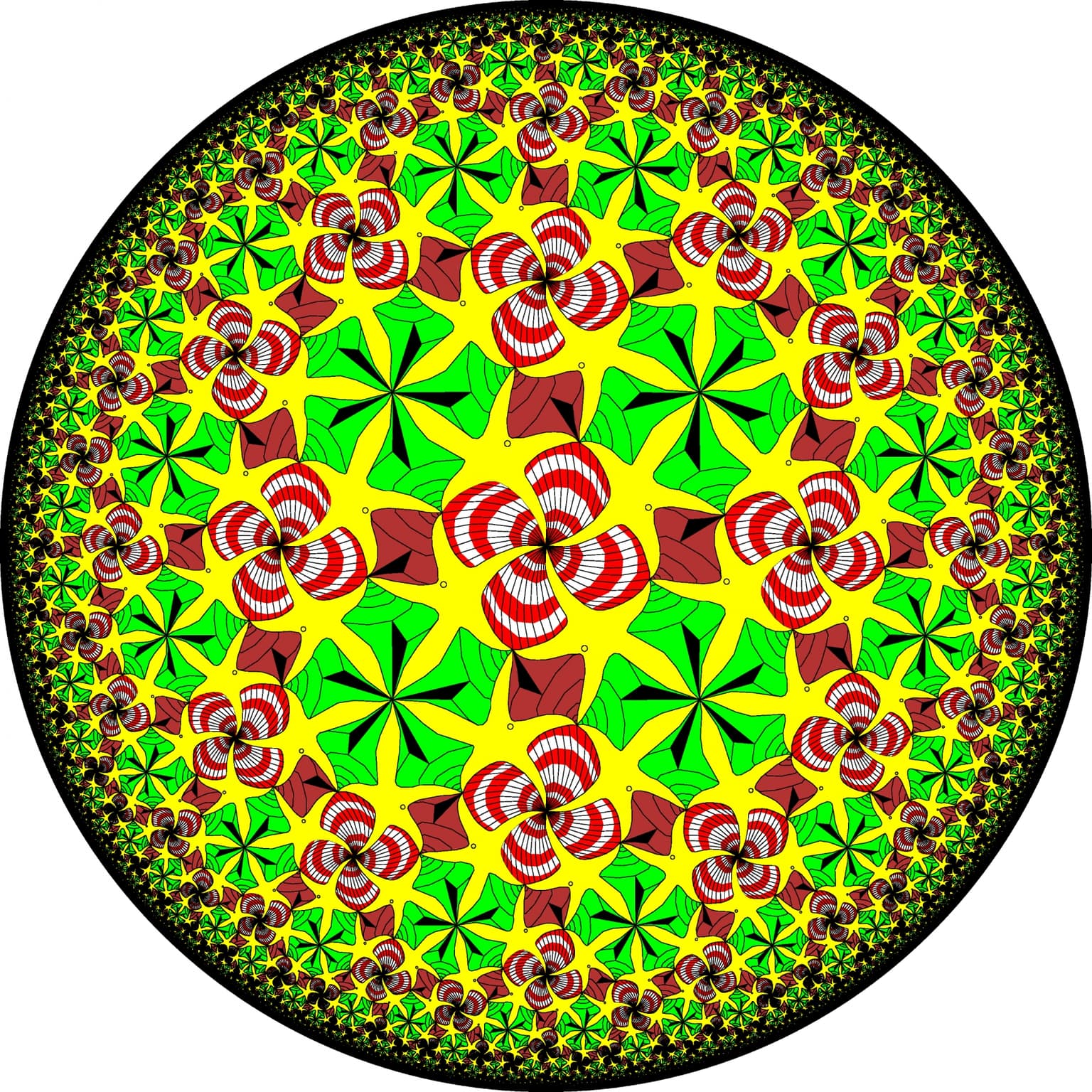
Shell pattern (4.5)
40 x 40 cm
Aluminum print
2018