Philippe Leblanc
Artists
Philippe Leblanc
Visual Artist - Architect Engineer
Brussels, Belgium
Statement
I am driven by art that stimulates and stills the mind at the same time, discipline and sensibility leading together to an aesthetic experience. Mathematics being a constant source of inspiration, I recently developed an interest in the writing of numbers of ancient civilizations, like the Maya, the Chinese and the Babylonians, adding a time and geographic dimension to my work. The Fibonacci series, closer to us, serves as a link between these distant numerations systems.
Artworks
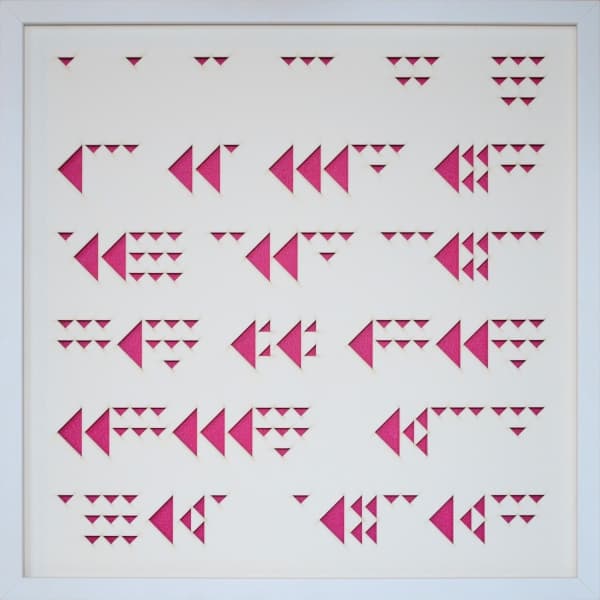
Babylonacci Pink Mini
38 x 38 cm
Watercolor paper, acrylic, wood, glass
2019
A laser cut white watercolor paper hides a colored background.
The perforations stand for the first 30 terms of the Fibonacci series written in the Babylonian numeral system, a sexagesimal positional numeral system inherited by the Sumerian civilization.
Two symbols (the arrow and the chevron worth ten arrows) suffice to write all numbers, since each sign’s position confers a value. Zero didn’t exist and was later represented by 2 inclined arrows.
In this work, arrows and chevrons were stylized using exclusively straight isosceles triangles of different sizes and orientations.
To represent numbers from 2 to 59, the system was simply additive.
For numbers larger than 59, the Babylonian used a place value system with a base of 60.

Mayanacci Gold Mini
38 x 38 cm
Watercolor paper, acrylic, gold leaf, wood, glass
2018
A laser cut white watercolor paper hides a background covered with gold leaf.
The perforations stand for the first 25 terms of the Fibonacci series written in the Maya numeral system, a vigesimal positional numeral system used by the Pre-Columbian Maya civilization.
Three symbols (a dot, a bar, and a shell) suffice to write all numbers, since each sign’s position confers a value, and they are read from the bottom up. The number 0 doesn't appear here.
Numbers 1 to 19 are written using repetitive additions, in which dots are worth 1 and bars are worth 5. Numbers larger than 20 (20 to the power 1) are written in two rows, numbers above 400 (20 to the power 2) in three rows, numbers above 8000 (20 to the power 3) in four rows, and so on.