Rashmi Sunder-Raj
Artists
Rashmi Sunder-Raj
Mathematical Artist
Waterloo, Ontario, Canada
Statement
I seek patterns to make sense of my world. Some of these I choose to interpret as visual images. Lately, I have been fascinated with the way that a simple linearly increasing sequence can be applied to shapes in an alternating manner to form serpentine patterns with interesting properties. This is an extension of my investigation into what I have been calling polygon and wedge squiggles where shapes, a sequence, and switching direction are used to construct compound shapes (see https://twitter.com/i/moments/1050938075222855680).
Artworks
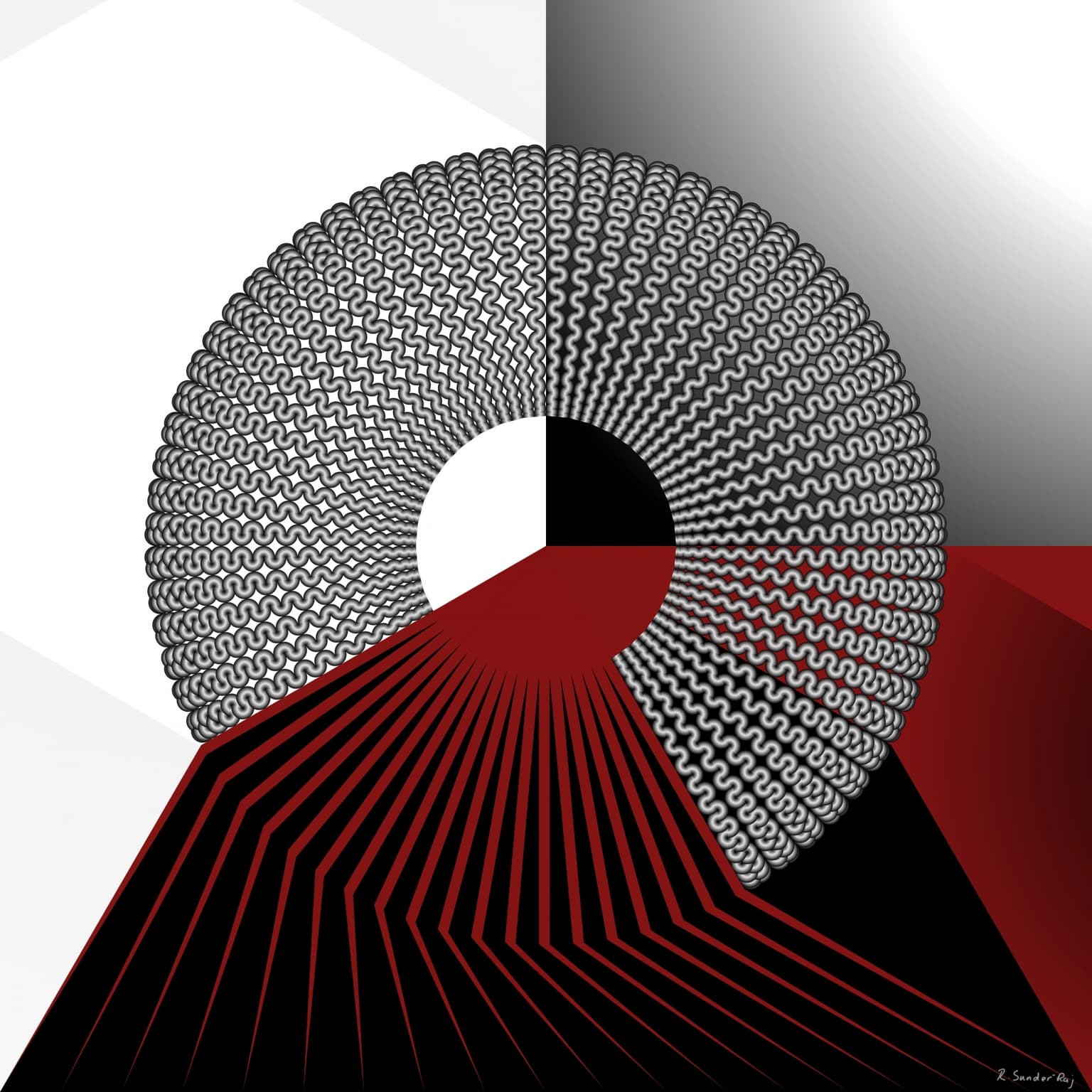
“Radial Sector Annulus” has a name inspired by that of a song...perhaps one can sense music trapped within.
It features a ring whose sectors consist of a variant of a wedge squiggle (a squiggle being visible down the center of the shape). The angle of each wedge is 10° (2π/36) and as a consequence, the shape obtained by the sequence of 1,2,3...36 wedges fits within a triangle whose least angle is 5° (2π/72). So 72 of them can fit together tightly to form a ring-like structure. Here, 1/4 of the ring has been obscured by radial lines which extend in a way which may or may not make sense. Hints of regular figures beckon beyond the boundaries for completion. How will perceptions and preconceptions influence what can be experienced?

One can construct a rhombus rosette by fitting n rhombs around a vertex, filling in the gaps with rhombs and continuing until the figure is convex. Alternatively, the same figure can be constructed using n copies of a crescent made of these rhombs. If n is even, this crescent has mirror symmetry and its concave part mates with its convex part when rotated from either corner, making it easy to use in constructing a squiggle-type shape.
This piece uses portions of a 22-fold rhombus rosette to construct such a shape, and from that, part of a ring. These shapes are split in two by colour. A casual glimpse may give the impression that one part is a shadow cast by the other...upon closer inspection each is revealed as part of a whole.