2019 Icerm Illustrating Mathematics
Albert Chern
Artists
Albert Chern
Postdoctoral Researcher of Mathematics
Institute of Mathematics, Technical University of Berlin
Berlin, Germany
Statement
As a visual artist and a mathematician whose research involves the 3-sphere in 4D, I seek familiar objects in the 3-sphere to wonder its geometric nature. Known as Hopf fibration, the 3-sphere has a consistent set of "vertical direction" analogous to the gravity direction in the world where we live. Contrasting our space, the corresponding horizontal planes in the 3-sphere cannot stitch together into a large portion of a floor. This phenomenon results in the famous "impossible staircase." The impossible staircase introduced by Penrose and M. C. Escher usually demonstrates that 2D drawings can represent objects not realizable in 3D. While not achievable in our 3D space, the impossible staircase is surprisingly everywhere in the 3-sphere!
Artworks
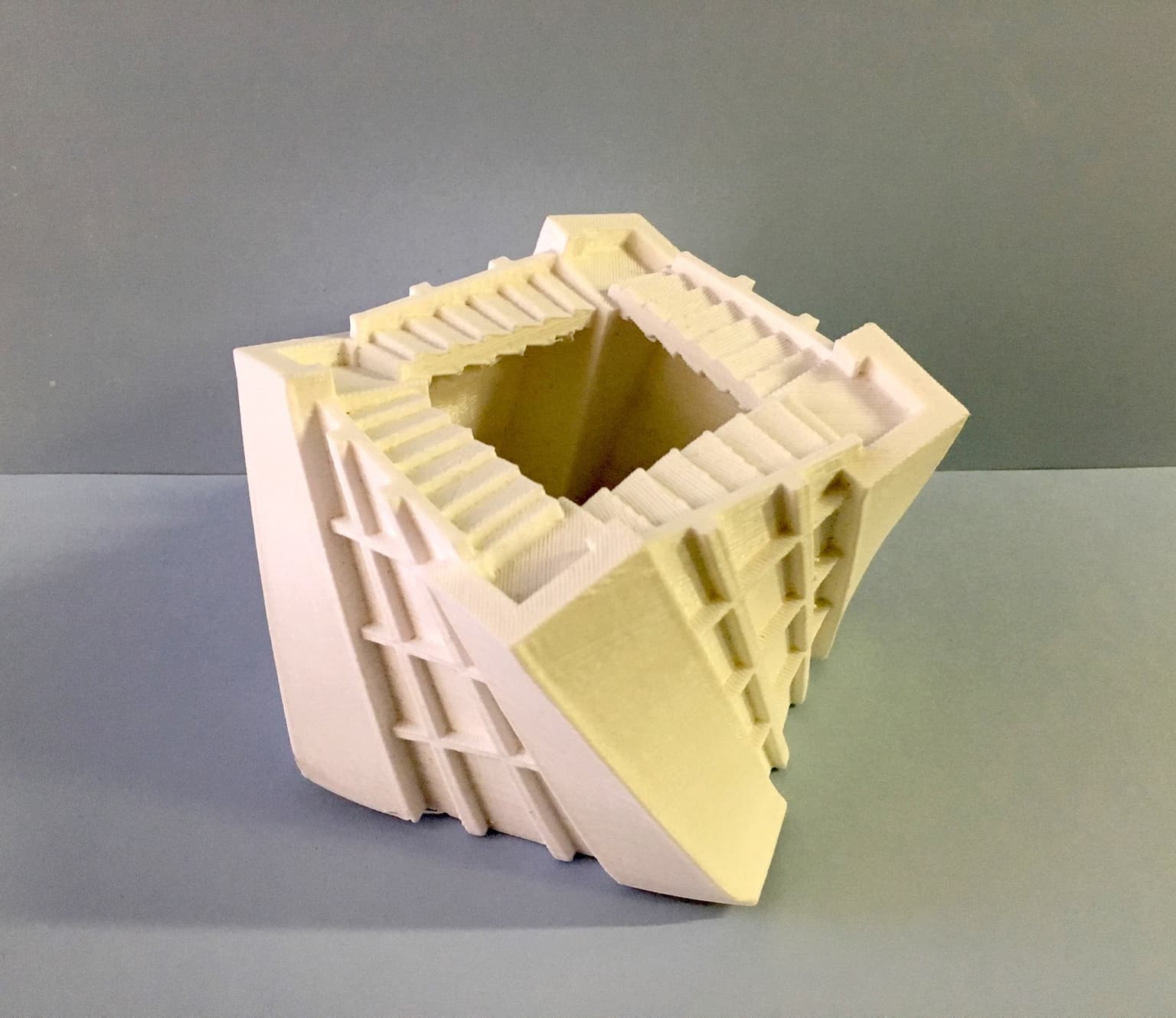
3D printed stairs in the 3-sphere
11 x 11 x 7 cm
3D print
2017