2019 Joint Mathematics Meetings
Diana Davis
Artists
Statement
I am a research mathematician studying geometry and dynamical systems. With Samuel Lelièvre, I have been studying periodic billiard paths on the regular pentagon, and we wrote a program in Sage to draw them. Periodic paths on the pentagon are so beautiful that I wanted to get them off the screen and into people's hands. I have made hundreds of pairs of earrings, and other objects decorated with these billiard pictures.
Artworks
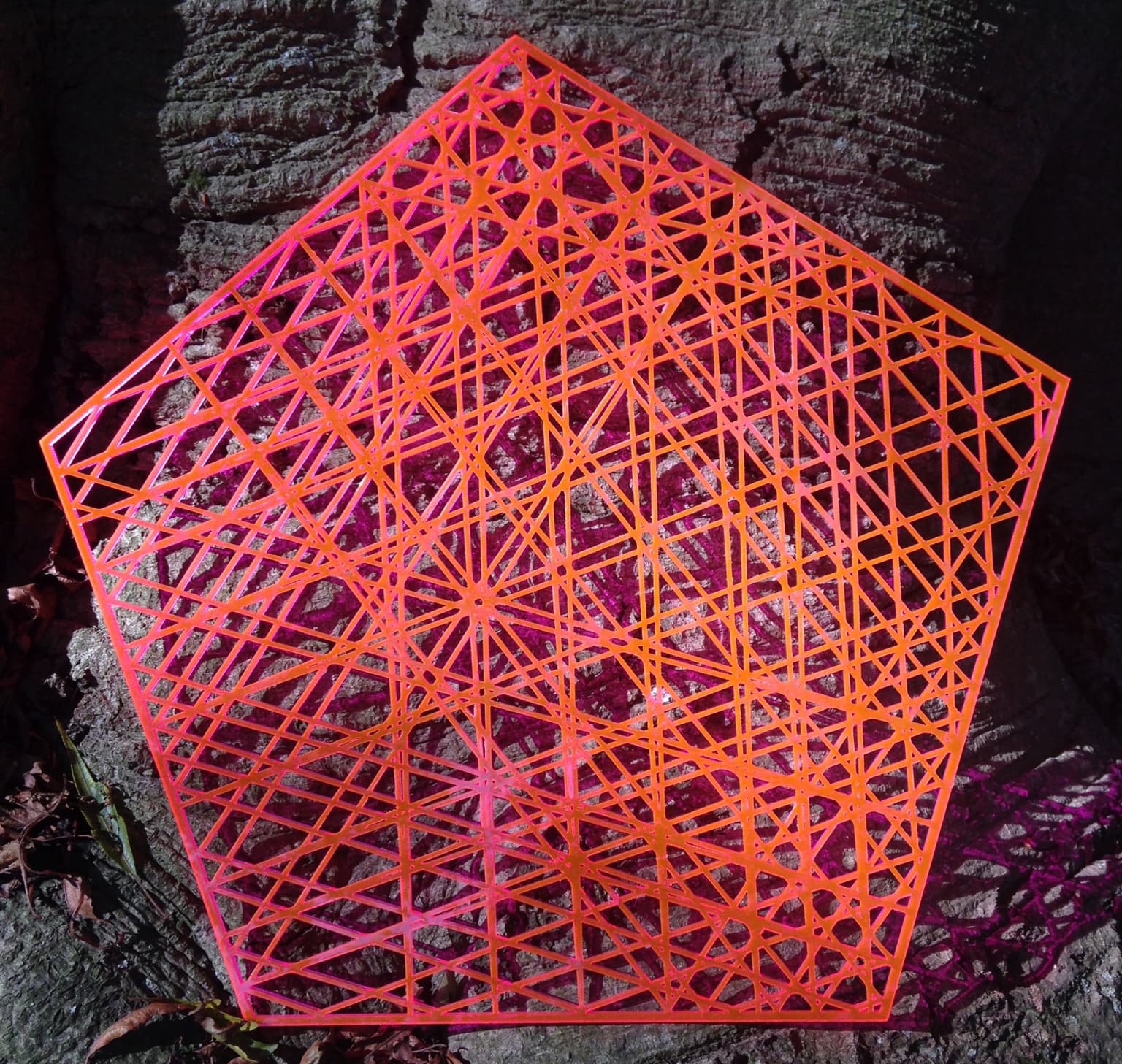
An asymmetrical periodic path on the regular pentagon
30 x 32 cm
Acrylic plastic
2018
This is a periodic billiard trajectory on the regular pentagon billiard table. The outer border is the table, and the trajectory is bouncing around inside it, following the usual billiard rule that the angle of incidence equals the angle of reflection. I have cut out the negative space outside of the billiard path and the border, to emphasize the trajectory. This trajectory passes through the midpoint of the lower left edge, which makes it especially symmetric. My research collaborator Samuel Lelièvre and I wrote a program in Sage to draw all of the (infinitely many) periodic billiard paths on the regular pentagon, and this is the most beautiful one whose only symmetry is reflection.
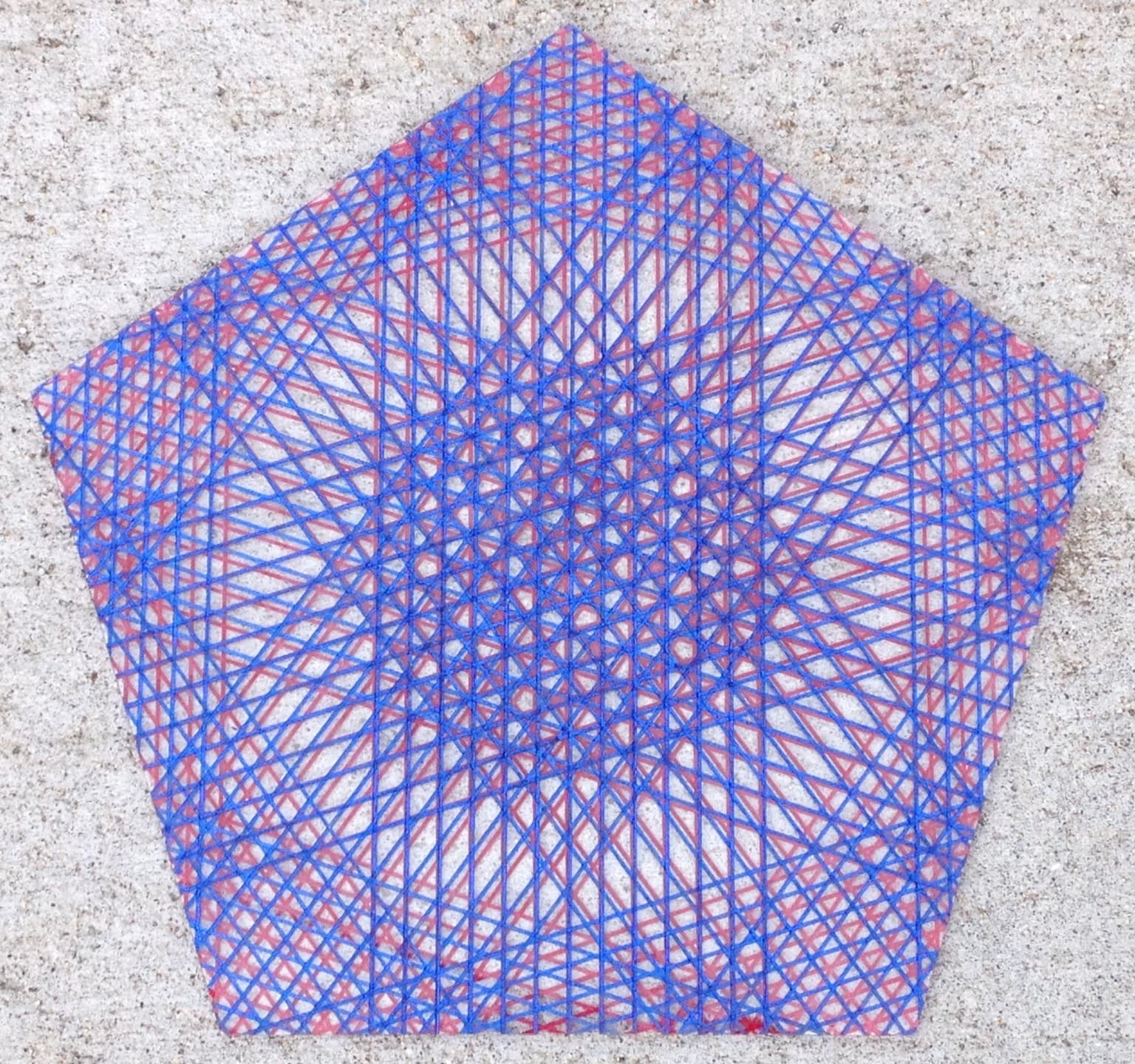
A periodic billiard path on the regular pentagon
30 x 32 cm
Acrylic plastic, nail polish
2018
This is a periodic billiard trajectory on the regular pentagon. The trajectory goes straight until it hits an edge, and then it bounces following the usual billiard rule that the angle of incidence equals the angle of reflection -- and in this case, it switches to the other side of the piece and changes color. Equivalently, if you had a ribbon that was red on one side and blue on the other and wrapped it around the table, this is the picture you would get. The two colors are mirror images of each other. When I show people a list of hundreds of periodic billiard trajectories and ask them which one is the most beautiful, about half of the people choose this one (or a similar one in the same family).