2019 Joint Mathematics Meetings
Debra Hydorn
Artists
Debra L. Hydorn
Professor of Mathematics
University of Mary Washington
Fredericksburg, Virginia, USA
Statement
I enjoy exploring relationships and patterns using Geometers Sketchpad and R. Most recently I have discovered some interesting patterns associated with the game of Bulgarian Solitaire. The game produces sequences of partitions of an integer n. The elements of the partition are ordered largest to smallest and the next partition is found by subtracting 1 from each element, creating a new element from those subtracted, and reordering largest to smallest. For example, 43211 would be followed by 5321. All such sequences will end in a cycle, regardless of the initial partition. Starting with a partition that is part of a cycle and overlaying that cycle with that of another integer has revealed some regular and irregular patterns.
Artworks
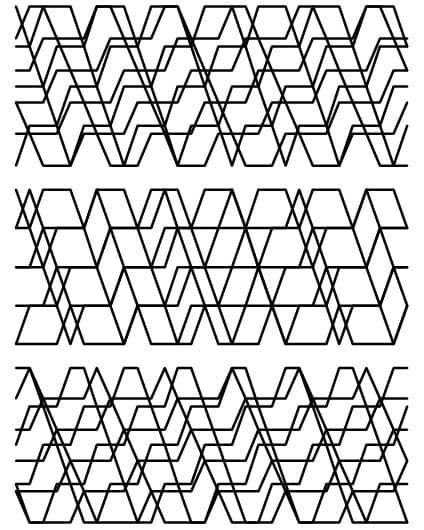
Partition Cycles for Integers Near 10
18 x 14 cm
Digital Print
2018