2019 Joint Mathematics Meetings
James Mai
Artists
James Mai
Professor of Art
School of Art, Illinois State University
Normal, Illinois, USA
Statement
My recent work has focused on the combinatoric development of "loop-forms", closed curves that connect 3 points on each of 4 radii of a circle. Loop-forms employ loops that orbit the circle's center 1, 2, or 3 times, singly or in combination, and never contact a point more than once. There are 6 types of loop-form, of which 3 are simple (using only 1 loop) and 3 are compound (using 2 or 3 loops). The complete set of 1+1+1-orbit compound loop-forms (3 independent 1-orbit loops) is comprised of 46 distinct forms, after elimination of any forms that repeat another after reflection or rotation. I have found forms from this set useful for studies in color relativity (simultaneous color contrast).
Artworks
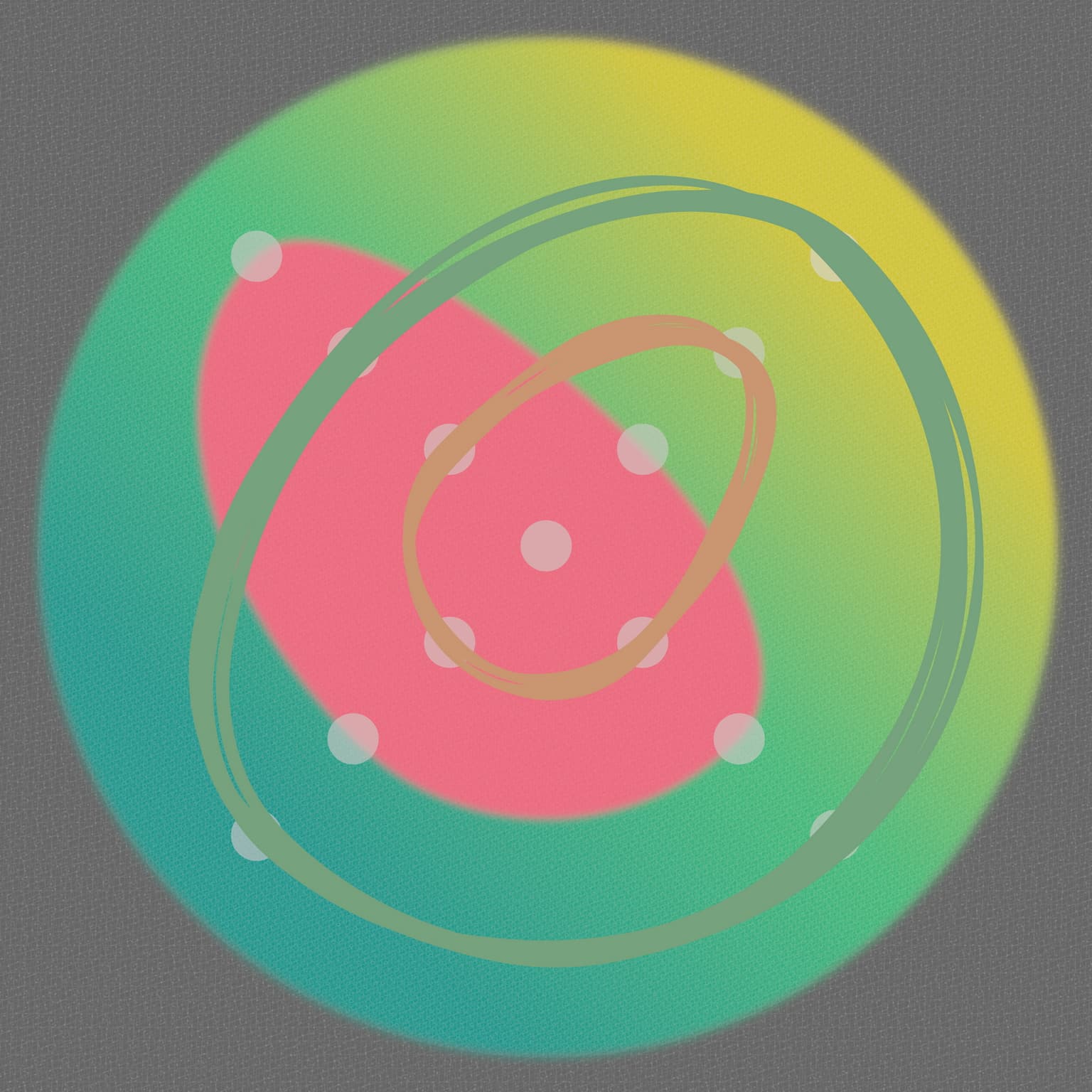
Roundabout (1211+2333+3122) (O+G on R+BG-YG)
20 x 20 cm
archival inkjet print
2018
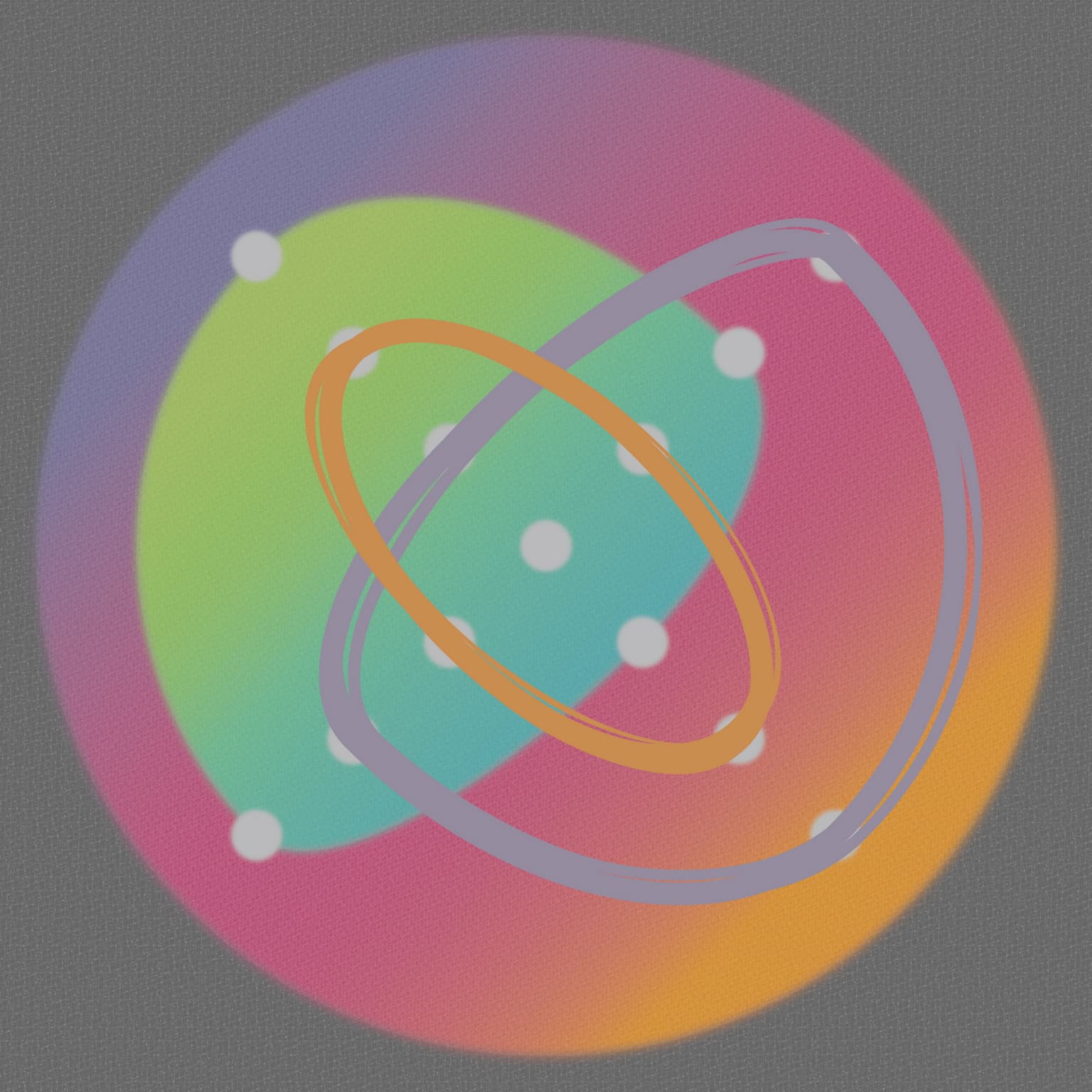
Roundabout (1332+2121+3213) (V+O on YG-BG+V-R-O)
20 x 20 cm
archival inkjet print
2018