2019 Joint Mathematics Meetings
Yuto Horikawa
Artists
Yuto Horikawa
Student
Department of Mechanical Engineering, Osaka University
Japan
Statement
I am interested in mathematics and crafting. Especially, visualization of a mathematical object is one of my lifeworks. Up to now, I have made various visualizations of mathematical objects using paper crafts, straw crafts and CG animations. Currently, I am a master course student in mechanical engineering of Osaka University, where I am developing a theoretical framework to construct a three-dimensional curved surface from pieces of elastic sheets which are embedded in the two-dimensional Euclidean space. Also, I am a Japanese translator of Desmos graphing calculator.
Artworks
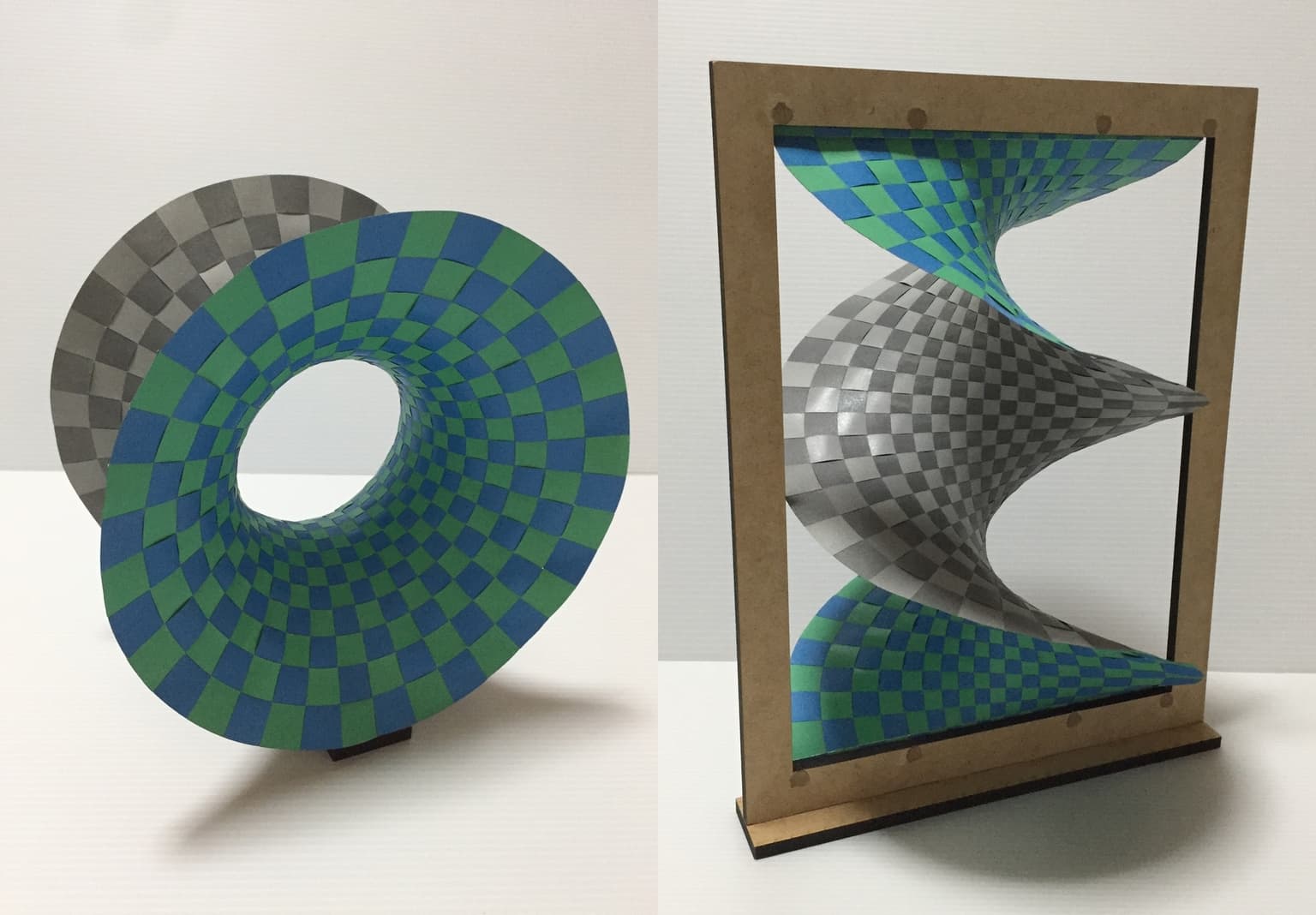
Isometric transformation between Catenoid and Helicoid
30 x 25 x 25 cm
paper, mdf, steel, magnet
2018
The left figure shows the paper-crafted catenoid surface and that of the helicoid is shown in the right. What is interesting here is that these surfaces can be transformed isometrically with each other, i.e. shape transformation without any straining. This is because the first fundamental form of the surfaces are identical. I made this model from weaving paper strips. The shape of the strips are obtained by minimizing the strain energy defined on a Riemannian manifold. The optimization problem is solved numerically using NURBS-based isogeometric analysis. This artwork is transformable, and thus the dimensions mentioned above are maximum sizes. The shape of the artwork will be sometimes changed by exhibitor during the event.