Aiman Soliman
Artists
Aiman Soliman
Research Scientist
NCSA - University of Illinois Urbana-Champaign
Champaign, Illinois, USA
Statement
I believe that mathematical art is a very unique form of creation. Unlike any other types of art, the rigor of mathematics provides a template for creativity, although it could be restrictive sometimes compared to free artistic expression. Yet these strict mathematical rules allow for an objective assessment of the success of an art project. I also find that mathematical artists share a similar experience with scientists in that solving a problem will usually lead to discovering another and, therefore, continuation of work in a natural way.
Artworks
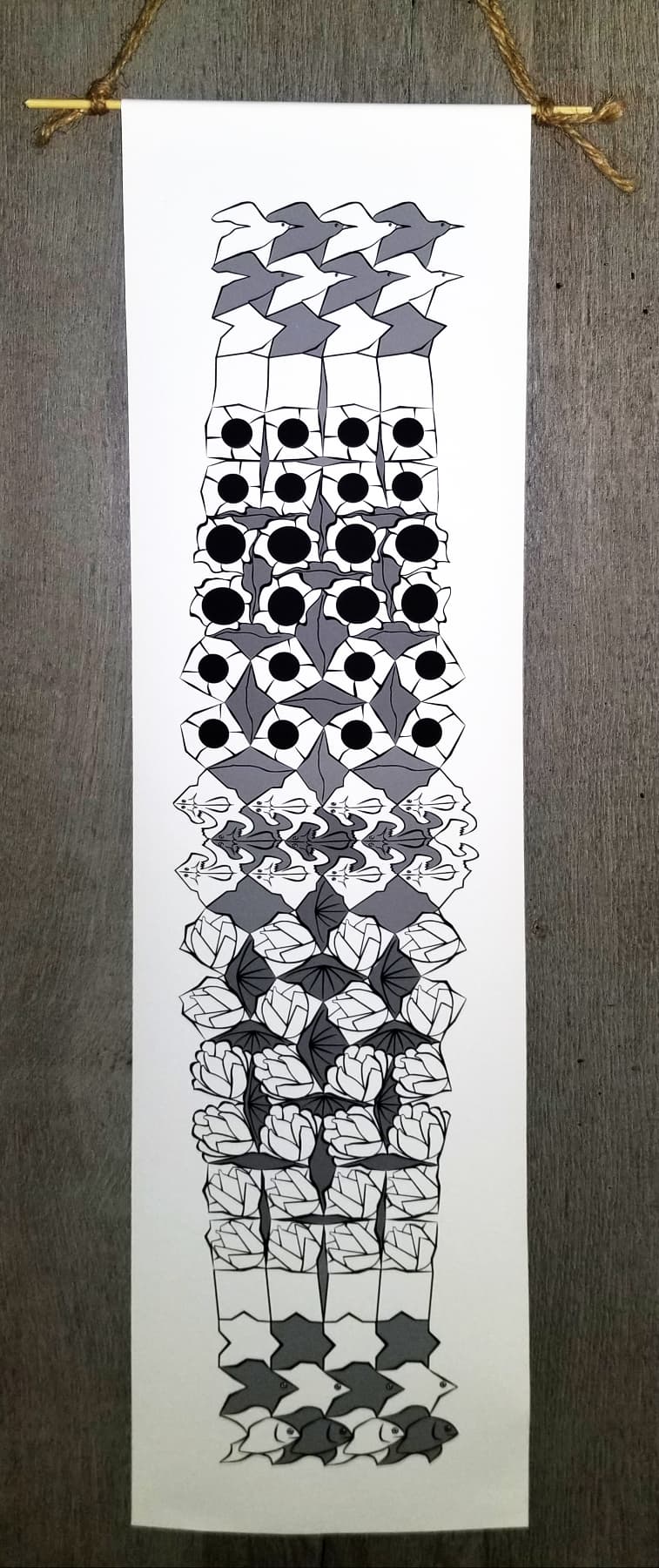
Auxetic deformation: birds, sunflowers, reptiles, water lilies, and fish.
50 x 25 cm
Archival print on fabric, a hanging scroll
2020
Unlike common elastic materials, Auxetic structures become wider when stretched (negative Poisson's ratio). One could imagine the upper most P1 tiling (birds) placed on a rubber sheet with slits made around the edges of each motif. As the design gets stretched the slits open leaving rhombic voids that transform the regular grid to a semi-regular one (sunflowers). The stretching reaches a maximum level when the rhombic voids become perfect squares (reptiles). If the stretch continues beyond this point, the grid transformation will be reversed passing by another semi-regular phase (water lilies) before returning to the original P1 grid (fish). The artwork is presented as a Japanese or a Chinese hanging scroll.