Curtis Palmer
Artists
Curtis L Palmer
Artist
Earth
Edmonton, Alberta, Canada
Statement
How does one represent an icosahedron? (0,±φ, ±1), (±1, 0, ±φ), (±φ, ±1, 0) works for some. In this work, In-spheres, Inter-spheres and Circum-spheres with origins on the vertices, mid-edges and face centres of an icosahedron define an arbitrary domain of competing forces acting upon an origin. Close attention to the orientation of these spheres results in pleasing patterns when viewed along axes of icosahedral symmetry.
Artworks
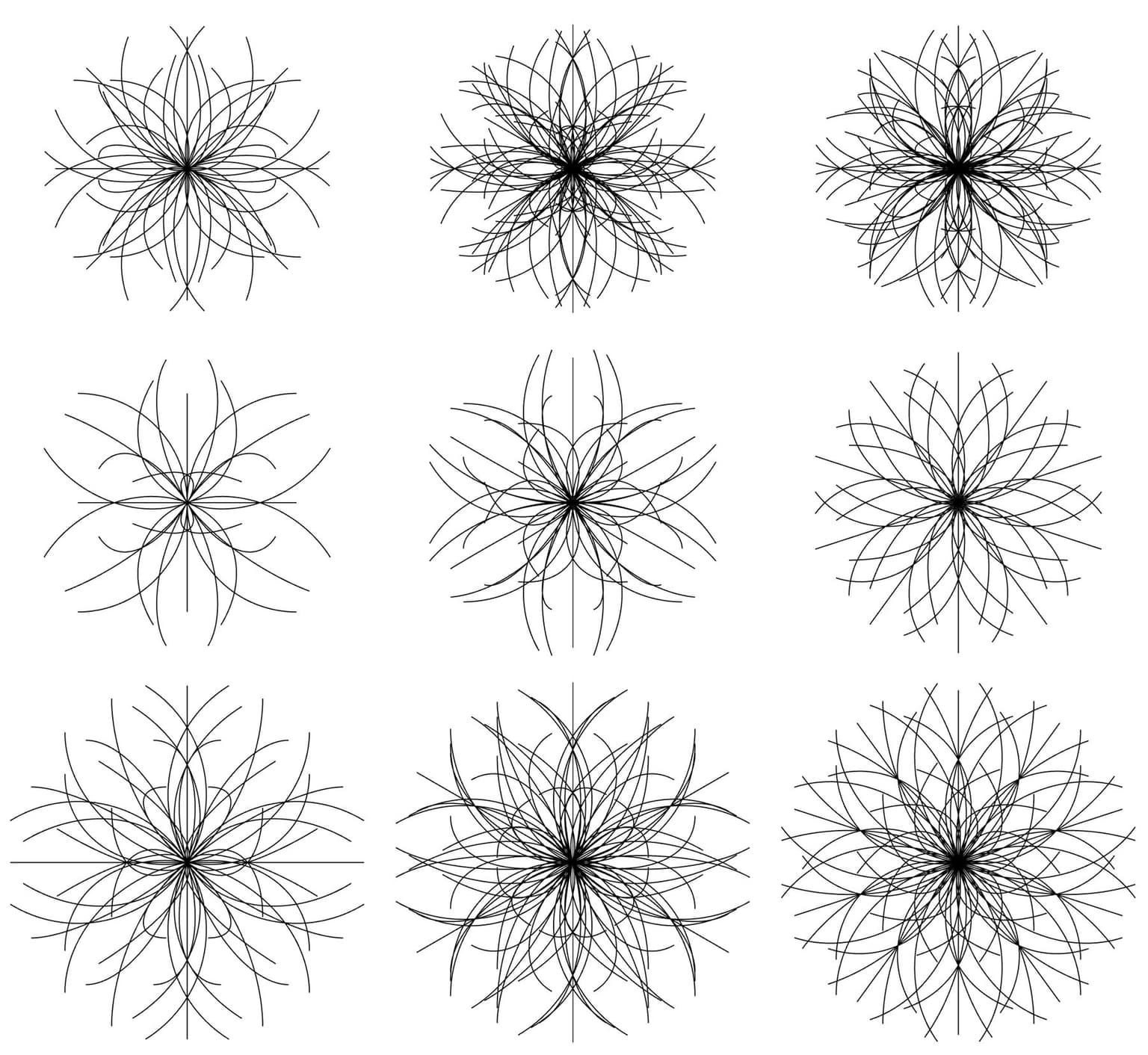
Tangent Cees of an Icosahedron
31 x 31 cm
Archival print
2020
Spheres in Rhinoceros 3D wire frame mode are represented as semi-circles, like the letter 'C'. Spheres of radii 1, 0.851=(cos(arctan(φ⁻¹))) and 0.795=(cos(arctan(2φ⁻²))) are positioned at the vertices, mid-faces and mid-edges of the icosahedron. They are all tangent to the origin. Five spheres at each vertex in a polar array with 72 degree rotation, three spheres at each face center with 120 degree rotation, and one sphere at each mid-edge of the icosahedron. These are plotted aligned to the axes of symmetry.