2020 Bridges Conference

Felicia Tabing

Artists

Felicia Tabing

Assistant Professor (Teaching) of Mathematics

University of Southern California

Los Angeles, California, USA

tabing@usc.edu

https://dornsife.usc.edu/feliciatabing/

Statement

In my recent series of artworks, my main goal is to utilize how I experience grapheme-color synesthesia to represent mathematical ideas in how I experience them in my mind. For example, each numeral has a color I associate to them, and the same with letters of the Arabic alphabet. I use the associated coloring to create work that represents special mathematical numbers as accurately as possibly to how I view them in my mind. I am experimenting with different media, such as watercolor pencil, acrylic paint, gouache, marker, and pen and pencil to get the right color effect and personality that a number has to me. I also use the idea of proofs without words to represent convergent series as a way to represent special numbers.

Artworks

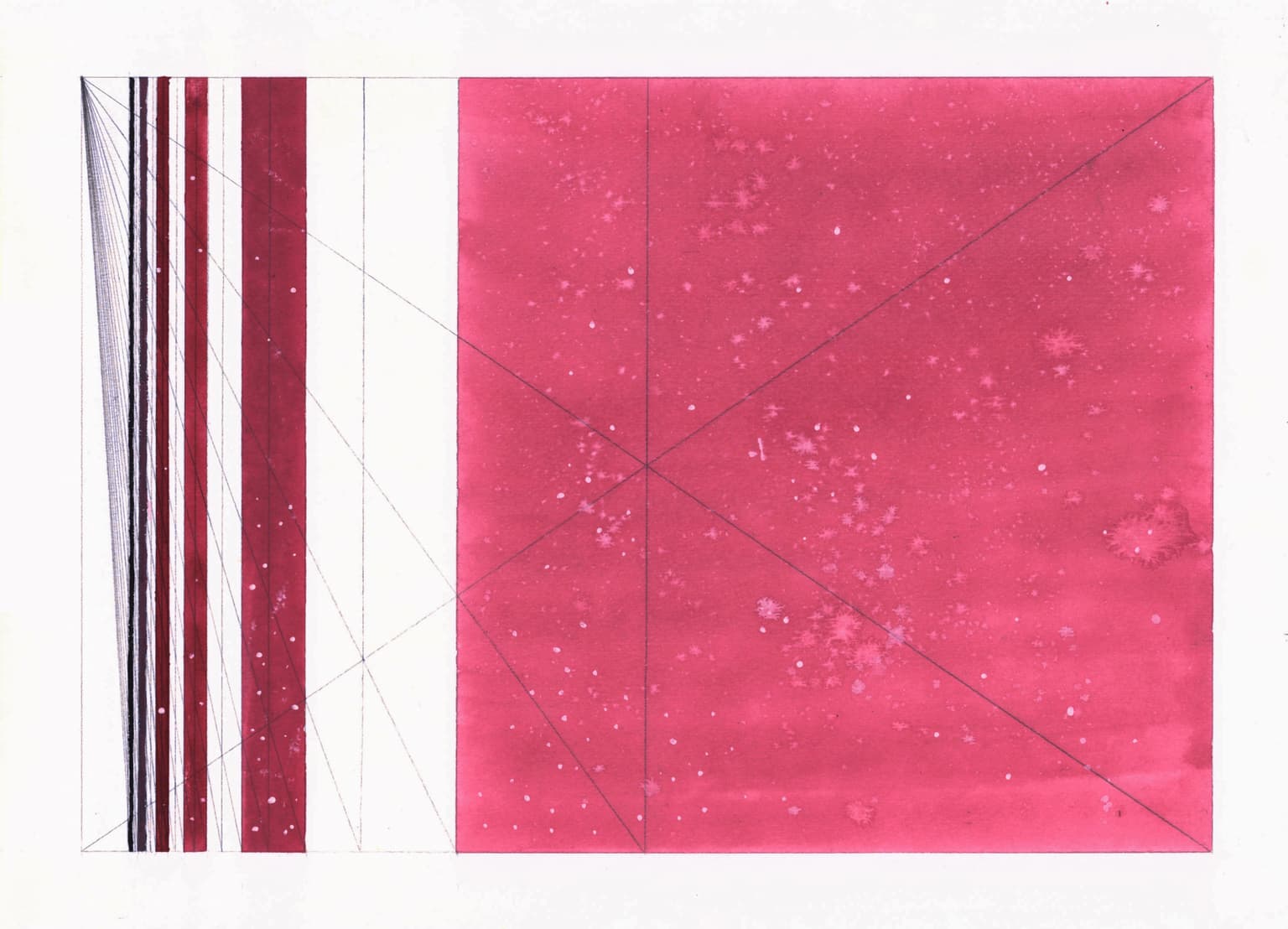
Image for entry 'Synesthesia Series: π/4'

Synesthesia Series: π/4

28 x 38 cm

Gouache and pencil on watercolor paper

2020

This represents the series for π/4, inspired by proofs without words. The larger rectangle framing the image represents area of unit 1. The construction lines are a technique I learned from perspective drawing in dividing a rectangle into n pieces, and are kept in the image to demonstrate the infinite sum. As the series for π/4 starts as "1–1/3," the rectangle was divided into thirds, and one-third of it was subtracted. The negative white space is subtracted out of the sum in total, while the colored-in rectangles are what remain in the infinite sum. The colors chosen represent the first three digits of π, 3.14, which are pink, white, and red respectively, with white flecks for the "sparkling" effect on my numbers from my synesthesia,