2020 Bridges Conference

Hanne Kekkonen

Artists

Hanne Kekkonen

Assistant professor

Delft Institute of Applied Mathematics, TU Delft

Delft, Netherlands

h.n.kekkonen@tudelft.nl

http://www.instagram.com/hannekekkonen/

Statement

I'm a mathematician interested in visualising topological shapes and using them to introduce otherwise difficult to grasp mathematical concepts to the general public. I was originally attracted to mathematical art by the possibilities offered by 3D-printing. However, I quickly realised that many printable shapes can also be crocheted. I create shapes from several different medium nowadays, but I still find crocheting the easiest way of making complex shapes. Recently, I have been experimenting on combining crocheting and plaster to create rigid, yet mathematically exact shapes. This method was used for creating the hyperbolic chalk 'board'.

Artworks

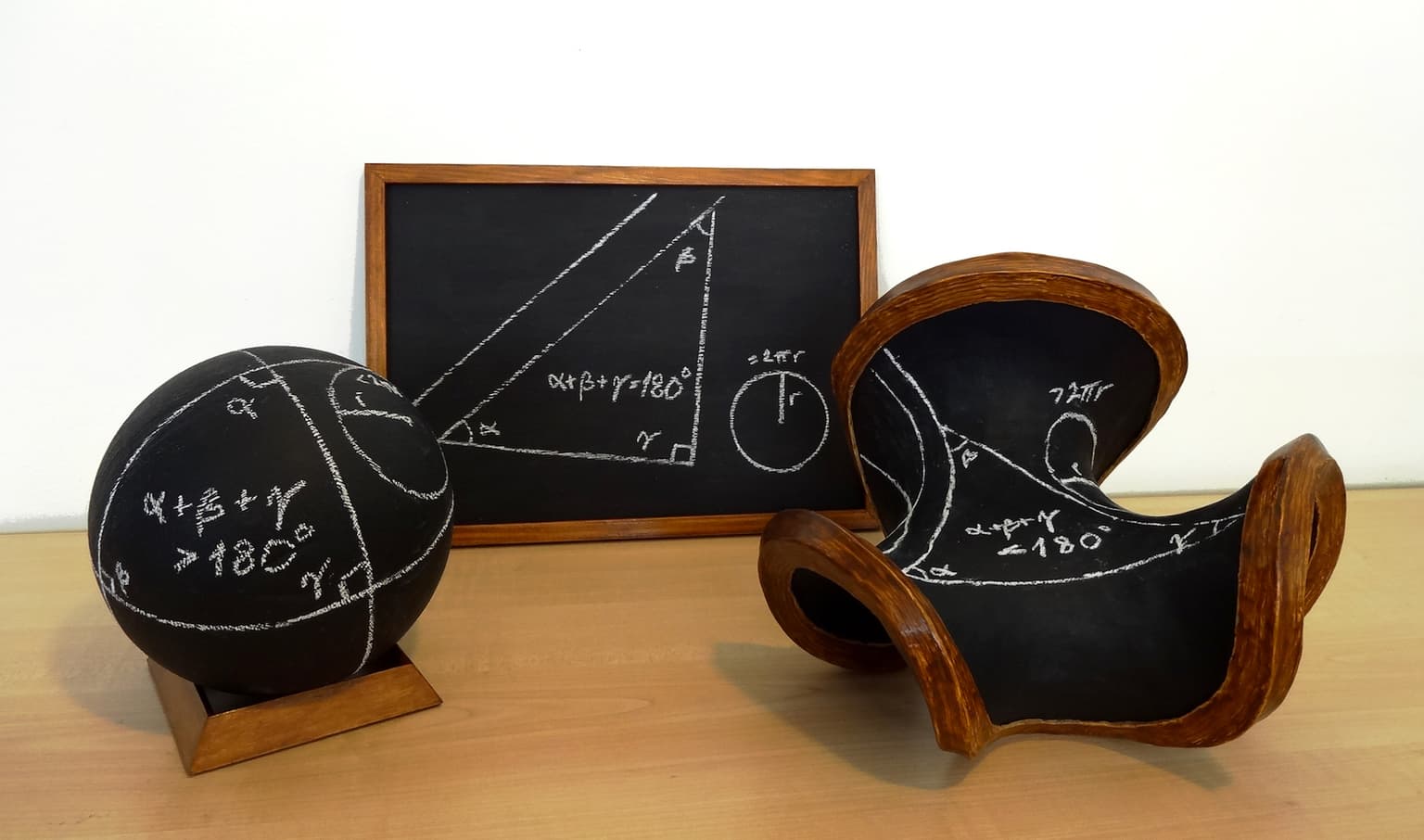
Image for entry 'Heresy'

Heresy

25 x 50 x 45 cm

Crocheted yarn, glue, plaster, wood, polystyrene, paint, chalk

2020

Demonstrating hyperbolic, spherical and Euclidean (flat) geometry with chalk surfaces. At school we learn certain things as fundamental truths: the angles of a triangle add up to 180°, a line has only one parallel line through a given point, and the circumference of a circle is 2πr. However, these facts are true only on flat surfaces. A sphere has constant positive curvature which affects the geometry: all triangles add up to more than 180°, there are no parallel lines and circles are shorter. A surface having constant negative curvature is called hyperbolic. On a hyperbolic plane triangles add up to less than 180°, a line has infinitely many parallels through a given point and circles are longer than 2πr.