Limor Cohen, Mirit Malihi, Shay Malkin
Artists
Limor Cohen, Mirit Malihi, Shay Malkin
Master students in Integrated Design, Applied Mathematics student
The Lab for Designing Mathematics, Holon Institute of Technology
Holon, Israel
Statement
In this art work we explore the Julia set f_c (z)=z^2+c where c∈(-2,0). The fractal curve in each of the 22 Perspex frames is the contour of the fractal. We believe that the 3D representation can help understand what we could only imagine – the behavior of fractal. This method indicates that indeed in this permutation for different c∈(0,2) lead to a convergence of the fractal -a line.
Artworks
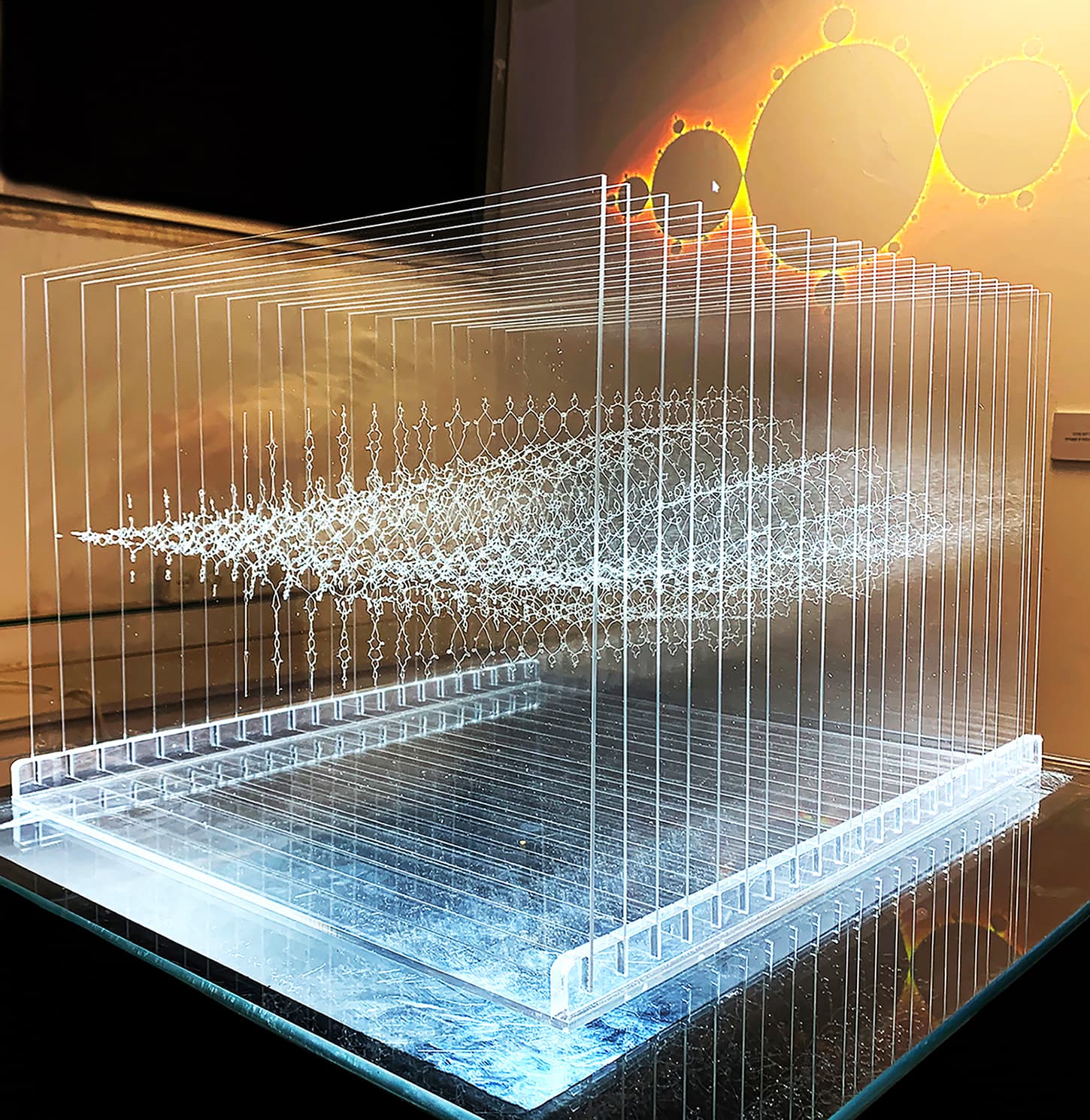
This art work is a three-dimensional object that describes a fractal convergence (Julia's group) following a 3D expression presented by a series of 22 different and parallel planes. These planes are built from 22 Perspex frames. On each Perspex engraved shape, the shape is obtained by changing the constant C from 0.6 to 1.4 in equal jumps. Together, a three-dimensional shape is created and gives a glimpse of the fractal in all three-dimensional sides.
The work sits on a podium with internal lighting that illuminates the engraving line and creates a special three-dimensional floating shape. In addition, the work is accompanied by a video showing all stages of fractal development from circle to line.