2020 Joint Mathematics Meetings
Dan Bach
Artists
Dan Bach
3d math artist / book designer
dansmathshop
oakland, california, usa
Statement
I am a former college mathematics teacher from California. I have used Mathematica for over a quarter century: in the classroom, for teacher workshops, and at conference talks. My students, over the years, created mathematical graphics, movies, and sounds; these were done either as class assignments or purely for pleasure. These days I'm a 3D math artist and interactive book author. For this art show, I have pre-paired two tall images, each depicting three related visuals about simple fractions, Farey sequences, or nested circles and spheres.
Artworks
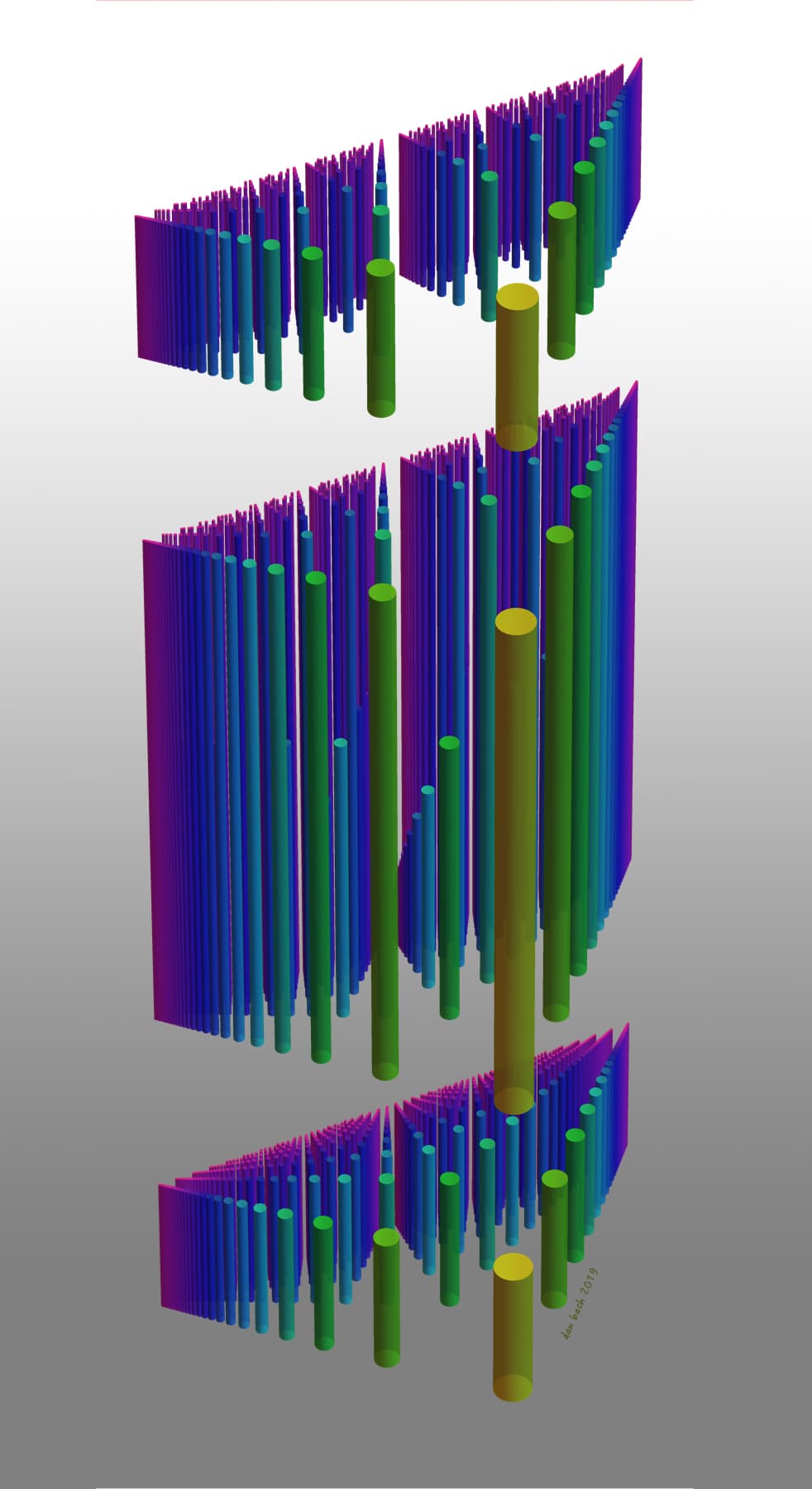
Proper fractions, rational numbers between 0 and 1, can be arranged in rows by denominator and represented by cylinders of decreasing radii and evolving colors. Some of the fractions will be in lowest terms, some won't. I figured out how to express this visually, with cylindrical columns full height if the numerator is prime to the denominator, and other cylinders cut down (or removed) according to their GCD. This creates a hint of fractality, and reminds us of Ford circles and infinite Farey sequences, but I decided the picture had to stop somewhere! (Click "Link" for an interactive 3D view)
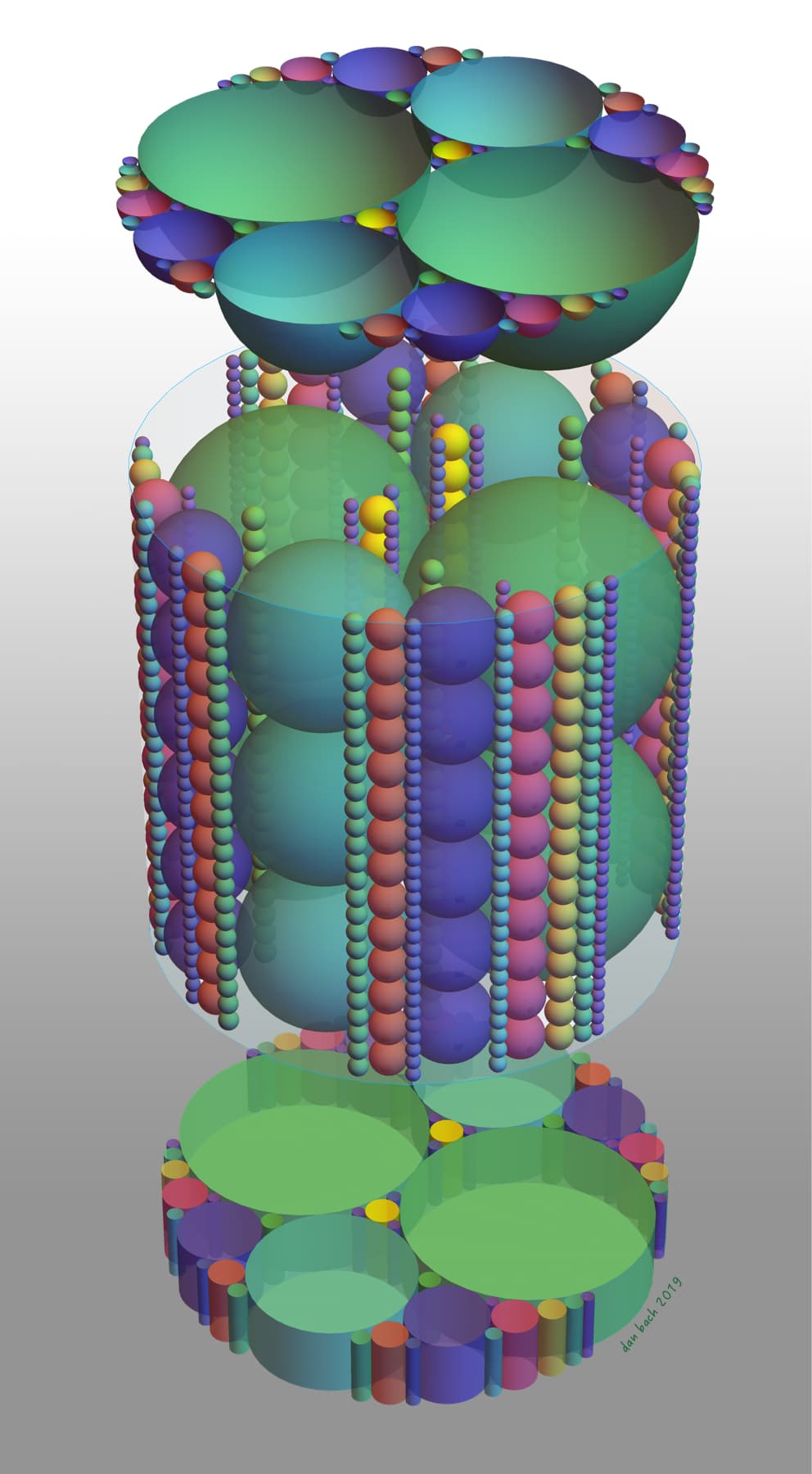
The Apollonian gasket consists of nested circles, of sizes formulated by Fermat in 1637. The radii of all circles in the standard gasket are unit fractions, and I wanted to show this using spheres that stack up to the exact height of the big cylinder. The number of spheres in each stack is the reciprocal of the radius, also known as the curvature! Here we have three views: the nested circles shown on top as a single layer of sliced spheres, then as stacks of spheres in a cylinder, and on the bottom just nested cylinders. This is not the same as filling all the spaces with the largest possible spheres, although those radii would obey a similar formula. (Click "Link" for an interactive 3D view)