Doug Dunham and Lisa Shier

Statement

We have been experimenting with different technologies for creating hyperbolic art, with a concentration on patterns in the Poincare circle model of hyperbolic geometry. In the past, we use a computer controlled embroidery machine to good effect. We are currently investigating the use of a computer controlled plotter/paper-cutter to create our patterns. Some schools have computer controlled paper cutters or plotters, so similar projects would be accessible to their students. Papercrafting is a way for primary and secondary school students to experience the creation of mathematical art. The current marketing of papercrafting to females suggests that it could be an excellent way to engage girls in both math and mathematical art.

Artworks

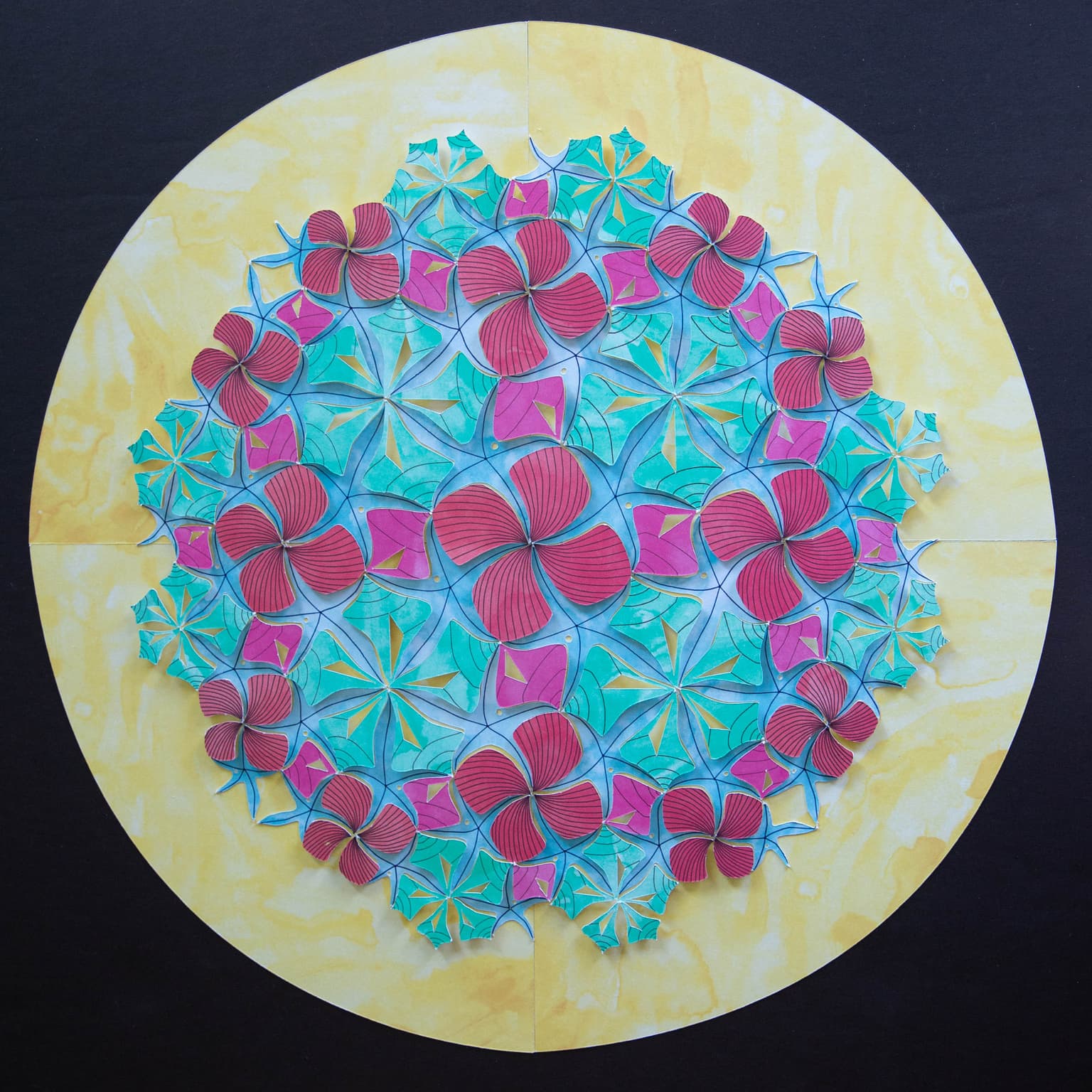
This pattern is inspired by M.C Escher's Regular Division Drawing 42, which is mathematically interesting since the apparent 4-fold rotation centers of scallop shells only have 2-fold rotational symmetry, and there are two kinds of 4-fold rotations at the meeting points of conchs. So Escher's pattern has symmetry group p4, or 442 in orbifold notation. Our hyperbolic version preserves the 2-fold scallop meeting points and has two kinds of 5-fold conch rotation centers, yielding the symmetry group 552 in orbifold notation. We placed spacers under the conchs, scallops, and snails in order to produce a 3D-like effect. We also used different colors than Escher. By experimentation, we chose paper colors that were fairly scale-invariant.