2020 Joint Mathematics Meetings

Douglas McKenna

Artists

Douglas McKenna

Software developer/artist/math researcher/eBook publisher

Mathemaesthetics, Inc.

Boulder, Colorado USA

doug@mathemaesthetics.com

http://www.mathemaesthetics.com/HilbertCurves.html

http://www.mathemaesthetics.com/MathArtPrints.html

Statement

The tension between symmetry and asymmetry in a mathematical object is where I feel true beauty lies. Satisfying mathematical art means finding a balance between platonic ideal and aesthetic choice. My holy grail is a mathematical object that a non-math-minded person finds indistinguishable from a purely aesthetic piece of art. Also gratifying is when one's aesthetically motivated explorations of a combinatorial space leads to new mathematical discoveries. Much of my artwork—starting with some of the plates in Mandelbrot's “The Fractal Geometry of Nature”—involves exploring recursive geometries, especially research into and enumerations of new space-filling curve motifs. And then having fun graphically with the results.

Artworks

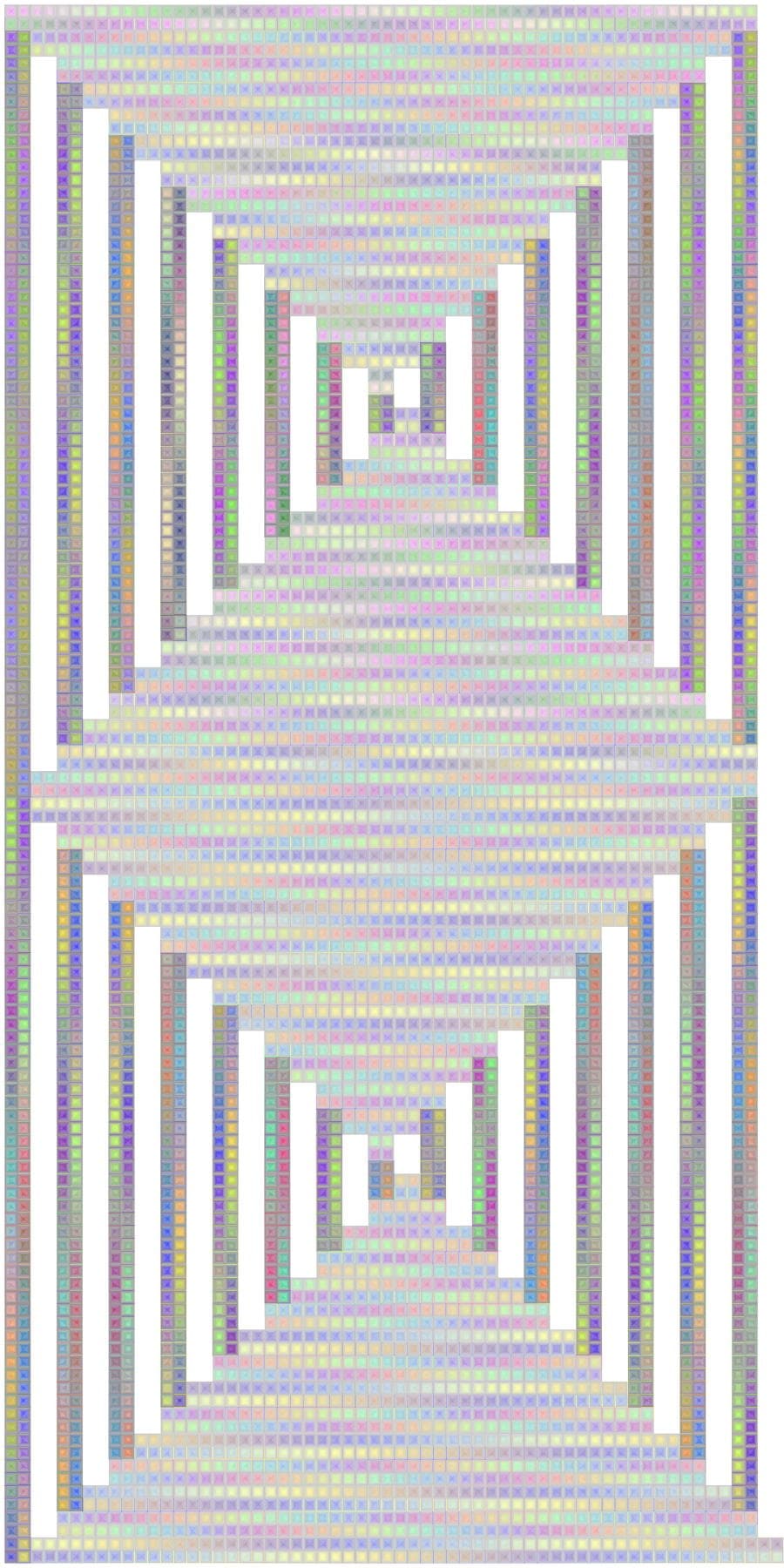
Image for entry 'A Unit Domino'

A Unit Domino

106 x 66 cm

Giclée print

2015

This piece is based upon an artist-discovered “half-domino” space-filling curve. The drawing comprises some half-million connected line segments, arranged in two perfectly recursive levels of double-spiral pairs, slowly changing color, in a single, over-one-mile-long self-avoiding path from lower left to lower right (the lower right square that sticks out is an integral part of its self-negative structure). The limiting curve covers a self-similar gasket tile with an infinitely long, almost-everywhere linear border. With an upside-down copy of itself, two such gaskets of unit area exactly cover a 1x2 domino, without overlap. The artist's app/eBook “Hilbert Curves” for iPad/iPhone explains how he discovered these beautiful constructions.